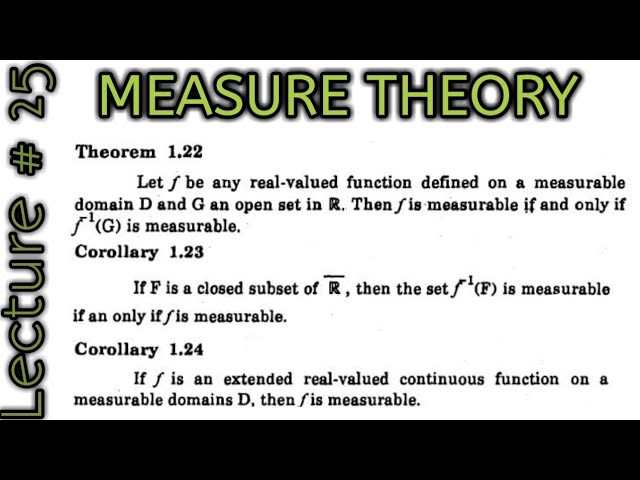
f is measurable iff inverse image of every open(Closed) set is measurable

preview_player
Показать описание

  1. Pure maths with Usama
Рекомендации по теме
f is measurable 0:16:09

f is measurable iff inverse image of every open(Closed) set is measurable

step function and 0:24:30

step function and inverse function of borel set is measurable

Every continuous function 0:28:31

Every continuous function is measurable||inverse image is measurable LEC 10(measure Theory)

Lecture 9: Lebesgue 1:24:57

Lecture 9: Lebesgue Measurable Functions

Real Analysis -Measurable 0:12:54

Real Analysis -Measurable function if f and g are real valued measurable function then proof ...

MEASURE THEORY: GOF 0:10:15

MEASURE THEORY: GOF is a measurable function under some conditions

Measurable Function Exercise 0:18:00

Measurable Function Exercise

Prove that a 0:01:46

Prove that a set is measurable iff (if and only if) complement of E is measurable | Measure Theory

If F and 0:12:13

If F and g are measurable functions then F+g and F-g are measurable, Mathematics/M.SC/PG/semester-2

The Inverse Image 0:06:52

The Inverse Image of a Set Under a Function: Definition and Examples

mod08lec51 - Various 0:30:39

mod08lec51 - Various modes of convergence of measurable functions

Measurable Functions in 0:09:55

Measurable Functions in Real Analysis

Measure Theory & 1:23:04

Measure Theory & Functional Analysis 6: Measurable Functions

3.6 - Measurable 0:26:10

3.6 - Measurable functions

MEASURE THEORY: Characteristic 0:28:16

MEASURE THEORY: Characteristic function of a set is measurable if the set is measurable

Lebesgue measurable functions: 0:25:10

Lebesgue measurable functions: almost everywhere concept and its implications (MAT)

ST342 031 0:05:40

ST342 031 Measurable functions 1 of 3

measurable function equivalent 0:22:05

measurable function equivalent statement proof simple approach full explanation .

Introduction to Lebesgue 0:15:04

Introduction to Lebesgue Measurable Functions

4.1 - Measurable 0:32:27

4.1 - Measurable functions

Characteristic Function of 0:02:18

Characteristic Function of a Set A is Measurable iff A is measurable

[Measure Space] Measurable 1:12:05

[Measure Space] Measurable function

If f be 0:13:44

If f be bounded and measurable then f is lebesgue integral UNIT 3RD (MEASURE THEORY) BY SONU

11 Measurable functions 1:30:53

11 Measurable functions

Комментарии
Автор

AOA sir plz lec ki number ING kary shukriya

afzaalahmad