filmov
tv
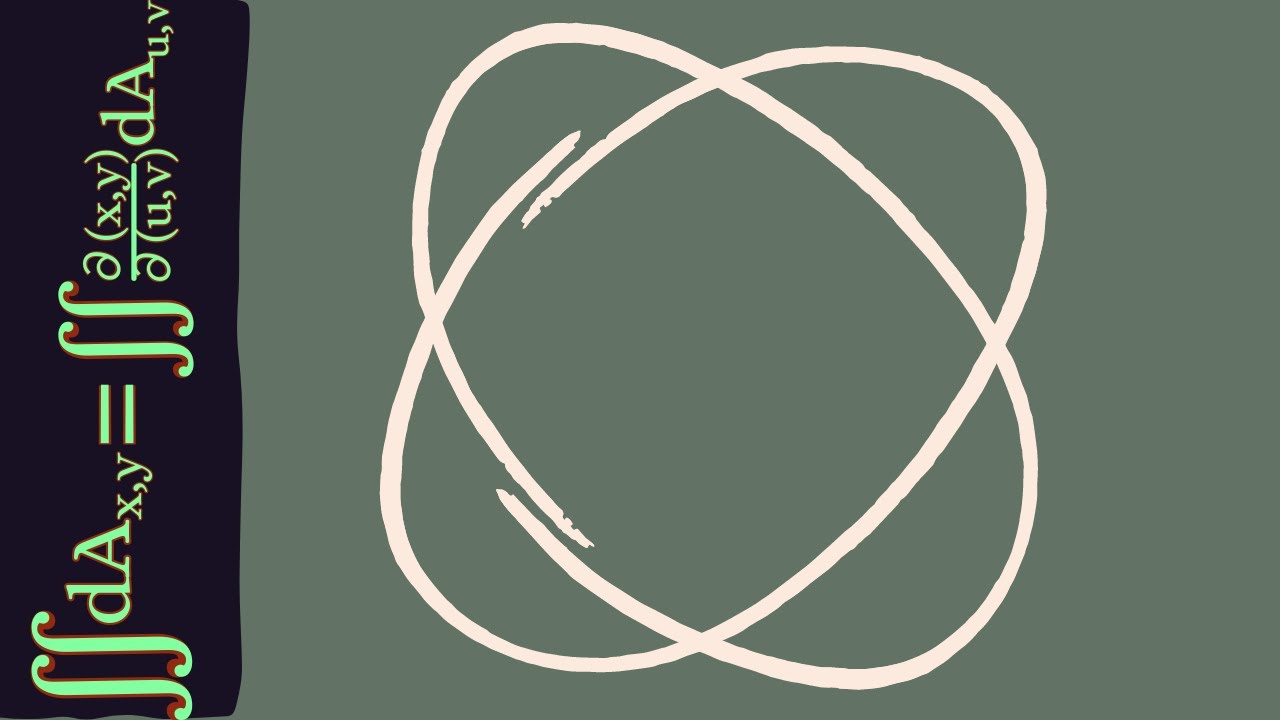
Overlapping area of ellipses.
Показать описание
If you are going to use an ad-blocker, considering using brave and tipping me BAT!
Books I like:
Abstract Algebra:
Differential Forms:
Number Theory:
Analysis:
Calculus:
My Filming Equipment:
Overlapping area of ellipses.
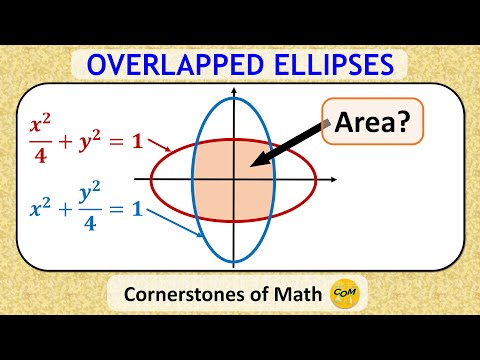
Calculating the Overlapped Area of Two Ellipses
Why slicing a cone gives an ellipse (beautiful proof)
How Can We Find The Area of the Intersection of Two Circles? - Solving Problems Together
Writing Equations of Ellipses In Standard Form and Graphing Ellipses - Conic Sections
Points of intersections of ellipses
HOW TO DRAW THE ELLIPSE BY INTERSECTION ARC OF CIRCLE METHOD
How to Draw Ellipse by four centre method in Engineering Drawing
Ellipses
Intersection of circle and ellipses
Draw ellipse by arc of circle method || All In One
Fitting ellipses for Carolina Bays - Definitely not thermokarst!
Ellipse by Arc of Circle Method
Mathematica Demo of the 3Blue1Brown Video on Ellipses as Conic Sections (use Dandelin Spheres)
Ellipse by Rectangle or Oblong Method.
Three Ellipses Doubling #shorts
Overlapping Ellipses
11 SN Ellipses and Points of Intersection with a straight line
R - calculating overlap between two ellipses
Ellipses inside Ellipses #shorts
Overlapping Ellipses
Overlapping Ellipses
Overlapping Ellipses
Ellipses Laws 101: Unraveling the Mysteries
Комментарии
 0:08:45
0:08:45
 0:06:49
0:06:49
 0:12:52
0:12:52
 0:04:35
0:04:35
 0:31:39
0:31:39
 0:07:58
0:07:58
 0:06:29
0:06:29
 0:05:40
0:05:40
 0:01:57
0:01:57
 0:14:29
0:14:29
 0:06:33
0:06:33
 0:09:05
0:09:05
 0:06:21
0:06:21
 0:39:35
0:39:35
 0:07:26
0:07:26
 0:00:59
0:00:59
 0:03:31
0:03:31
 0:08:42
0:08:42
 0:03:41
0:03:41
 0:00:59
0:00:59
 0:03:11
0:03:11
 0:03:23
0:03:23
 0:03:18
0:03:18
 0:00:52
0:00:52