What is a Theorem, Corollary, Conjecture, Lemma, Axiom, and Proposition?

preview_player
Показать описание
In this video, I will explain the differences between a theorem, corollary, conjecture, lemma, axiom, and proposition. You need to know these terminologies if you are taking a class about proofs or discrete mathematics. A proposition is a statement that is either true or false. An axiom is a statement that we assume to be true (self-evidently true) and we do not need to prove it. A lemma is a small result that has been proved, and it is used to prove a theorem. A theorem is a significant result that has been proved. A corollary is a theorem that follows on from another theorem. A conjecture is something that someone (usually a famous person) says is true but they have not been able to prove or disprove it.
  1. Quoc Dat Phung
  2. theorem
  3. corollary
  4. conjecture
  5. lemma
  6. axiom
Рекомендации по теме
What is a 0:03:00

What is a Theorem, Corollary, Conjecture, Lemma, Axiom, and Proposition?

What is a 0:01:38

What is a Theorem, a Corollary, an Axiom, a Lemma

Corollary 1 of 0:07:23

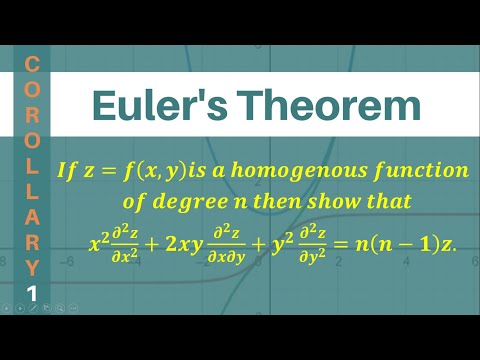
Corollary 1 of Euler's Theorem Formula and Proof

Inscribed Angle Theorem 0:02:15

Inscribed Angle Theorem Corollary | Maths

Theorem, Lemma, Corollary, 0:04:38

Theorem, Lemma, Corollary, Axiom and Conjecture. Are they Same? | Discrete Mathematics

Mean Value Theorem: 0:06:56

Mean Value Theorem: Corollary Theorems

What is the 0:01:00

What is the difference between a Theorem, a Lemma, and a Corollary? with examples.

Axiom, Theorem, Lemma, 0:08:47

Axiom, Theorem, Lemma, Corollary

Corollary of Thales 0:12:00

Corollary of Thales theorem( Basic Proportionality Theorem)

What is the 0:03:38

What is the corollary of the exterior angle theorem ?

🔵 Theory Theorem 0:15:51

🔵 Theory Theorem Hypothesis Postulate Corollary Axiom - Theory Postulate Corollary Axiom Meaning

Euler's Theorem |Corollary 0:06:32

Euler's Theorem |Corollary 1

AXIOMS -POSTULATES -THEOREM- 0:05:30

AXIOMS -POSTULATES -THEOREM- COROLLARY - Basics

5.3.1 Theorem, Proof, 0:10:38

5.3.1 Theorem, Proof, & Corollary

Proof of Fundamental 0:04:29

Proof of Fundamental theorem corollary in Graph theory.

what is corollary? 0:00:39

what is corollary? Understanding Corollaries: Key Points and Significance in Mathematics

Corollary of Basic 0:07:11

Corollary of Basic Proportionality Theorem | Class 10 | Geometry | Math's |2020

Difference between Axioms, 0:09:45

Difference between Axioms, Definition, Lemma, Theorem, Corollary, Conjecture, Equation, and Formula

Terminologies; Proof,Axiom(or postulate),Theorem,Hypothesis,premise,Lemma,Corollary,Conjecture 0:01:30

Terminologies; Proof,Axiom(or postulate),Theorem,Hypothesis,premise,Lemma,Corollary,Conjecture

Theorem and Corollary 0:05:41

Theorem and Corollary Lecture-5 EUCLID GEOMETRY (class-9)

Inscribed Angle Theorem: 0:06:34

Inscribed Angle Theorem: Corollary Properties

UNIVERSAL TRUTHS:- AXIOMS 0:11:57

UNIVERSAL TRUTHS:- AXIOMS & POSTULATES. STATEMENT, THEOREM & COROLLARY - All Definitions| CL...

Theorem + corollary 0:08:52

Theorem + corollary

Mathematics: What's the 0:04:11

Mathematics: What's the difference between theorem, lemma and corollary? (8 Solutions!!)

Комментарии
Автор

Straight to the point and informative, very helpful video!

spaceiscool
Автор

What about “ 2+ 2 = 4” is this an axiom because we cannot prove it is true but we assume it is?

MathCuriousity
Автор

Also out of curiosity can you help me understand what an “axiomatic approach” in math is and what would the opposite approach be?! I thought ALL math fields were axiomatic?

MathCuriousity
Автор

Wait a minute. I just read that a proposition is statement that has been proven true - just like a theorem - except not as important.

MathCuriousity