What is General Relativity? Lesson 8: Intro to the metric connection and the induced metric.

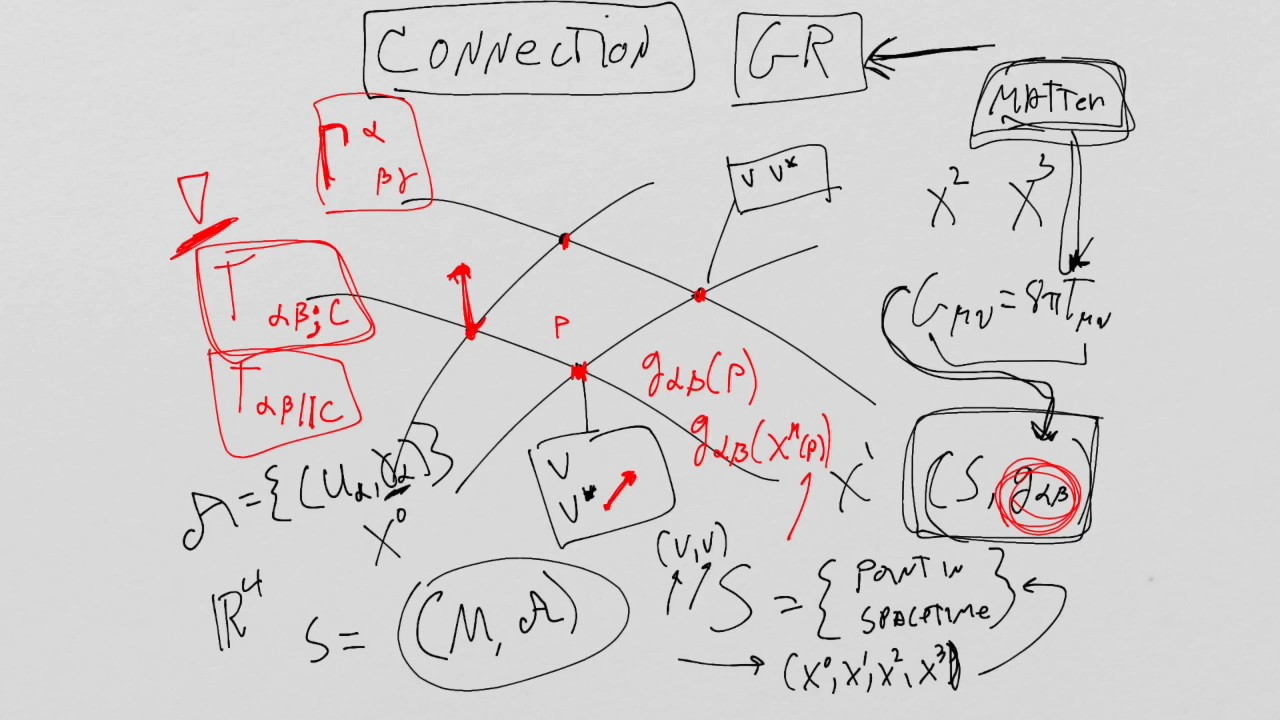
This lesson is an introduction to the concept of the metric connection followed by a long exercise in classical differential geometry. It is a long lesson because I complete a full example: the derivation of the metric of the "glome" induced by the Euclidean metric of 4-dimensional space. It is probably fine to skip the full calculation, it is not needed for basic GR work, but it does help develop facility and familiarity with the metric.

[keshavshrestha]

As we can intrinsically measure distance by metric tensor and curvature by using Riemann curvature tensor, similarly can we know the shape of any geometric object intrinsically.? Meaning can we intrinsically measure the shape of universe using GR..?

[Malamba]

When you mention “inside the glome” versus “in the higher dimensional space”, is that the same as the terms “intrinsic geometry” versus “extrinsic geometry”? Thank you very much for these lectures.

[jason_liam]

You've done a lot of mistakes in the lesson 7 [The Glome]: Kindly correct or annotate those.The following are some of the mistakes:
At 32:14 shouldn't the 1st term be just *r cos(phi_1) e_1* instead of
**r^2cos(phi_1)^2 e_1* ? Also at 27:57 the 2nd summation term should be
*sum(X^k)^2* and there will be no e^k inside this sum as you've written.

[tinhadziveljkovic]

Hey, thanks for the video. At the end of the video you compared the space part of the Minkowski metric to the metric on the glome, but if I think of the glome, I don't "cover" the whole space with it. Are we "describing" the 3 spatial coordinates with the glome due to some homeomorphism? (Like we were able to "chart" the 2D plane with a 2D sphere, stereographic projection)? If not, I'm not sure how those glome coordinates work for parametrization of the whole 3D spatial space. Thanks in advance! :)

[Gooch]

What a great channel! Starting with the series on manifolds and tensors, and now GR. Especially thanks for emphasizing the basis of a tensor and not getting tired of dragging around the vectors and covectors!
One minor note: at 9:05 when defining the inverse metric, wouldn't it be more natural to just contract on one index and get the delta symbol as the result? In case we sum over both indices we should get a 4 instead of a 1, shouldn't we?
Pls keepthis awesome videos going :-)

[tursinbayoteev]

Is glome a hypersurface? 3-dim surface of a sphere embedded into 4-dim?

[maheshudupa]

This is just great, I'm excited finally as a mathematics student I feel I'm having the best seat in viewing the fabric of the space time. I've a doubt though, I'm having a hard time visualising 'metric' as a 3*3 (in this example) 'matrix'. How does it result in a 1*1 sort of matrix, which is the result of the innerproduct i.e the metric. TIA

[cvictor]

at 1:18:10, how did u jump to conclusion so quickly that metric on glome has no off diagonal terms?