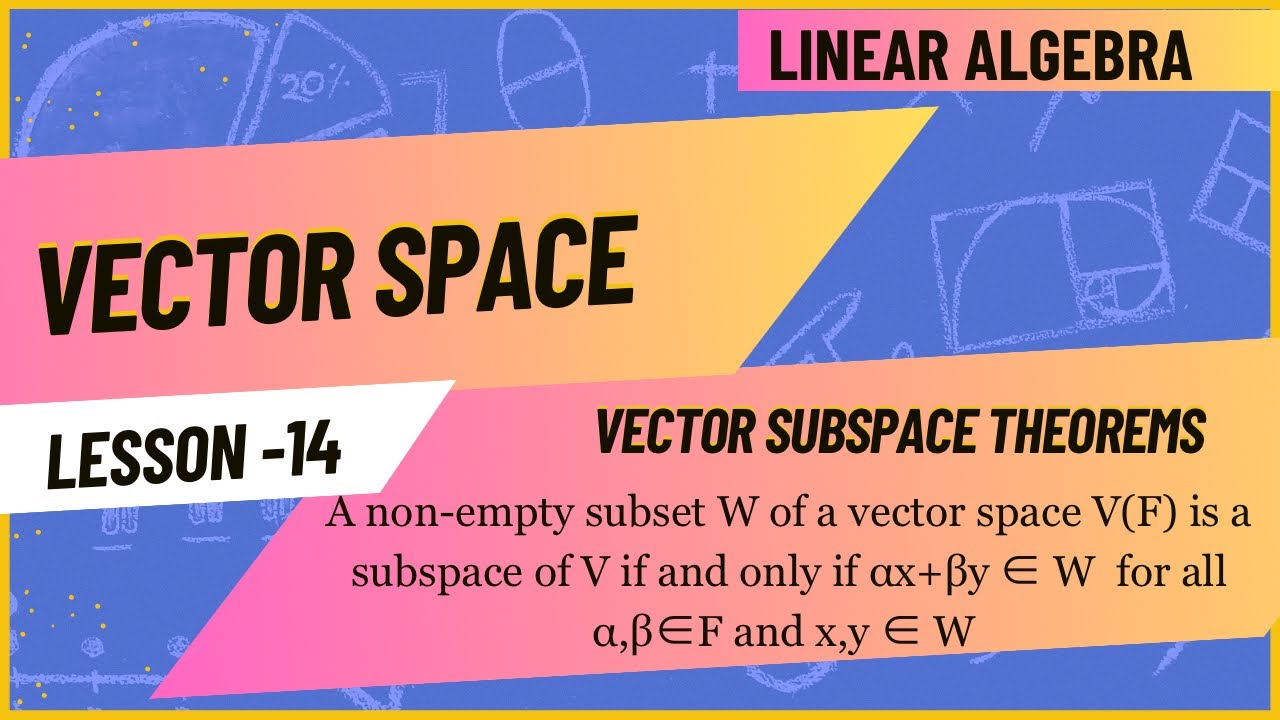
A non-empty subset W of vector space is subspace of V iff αx+βy ∈ W for all α,β∈F and x,y∈W

Watch other vector space videos here:

Lesson 1 Vectors and fields in Linear Algebra (Introduction to vector space) :

Lesson 2 Vector Space, properties of addition, properties of scalar multiplication

Lesson 3: General properties of vector space i) α.0 = 0 ∀ α∈F ii)0.x = 0 ∀ x∈V

Lesson 4: General properties of vector space [ α(-x) = - (αx ) = (-α)x ∀ α∈ F and x ∈ V ]

Lesson 5: General properties of vector space i) α(x-y) = αx-αy ∀ α∈ F and x,y∈ V

Lesson 6 : Every field is a vector space over itself ( vector space therorem)

Lesson 7: Prove that the set of real numbers is a vector space over itself.

Lesson 8: prove that the set of all complex numbers is a vector space over itself

Lesson 9: Prove that the set of complex numbers is a vector space over the field of real numbers

Lesson 10: Let R be a field of real numbers and let V = {( x,y):x,y ∈R }.

Lesson 11:Prove that set of m*n matrices is a vector space over F under addition and multiplication of matrix.

Lesson-12 Vector Subspace | Linear Algebra

Lesson 13: A non-empty subset W of vector space is subspace of V iff αx+βy ∈ W for all α,β∈F and x,y∈W