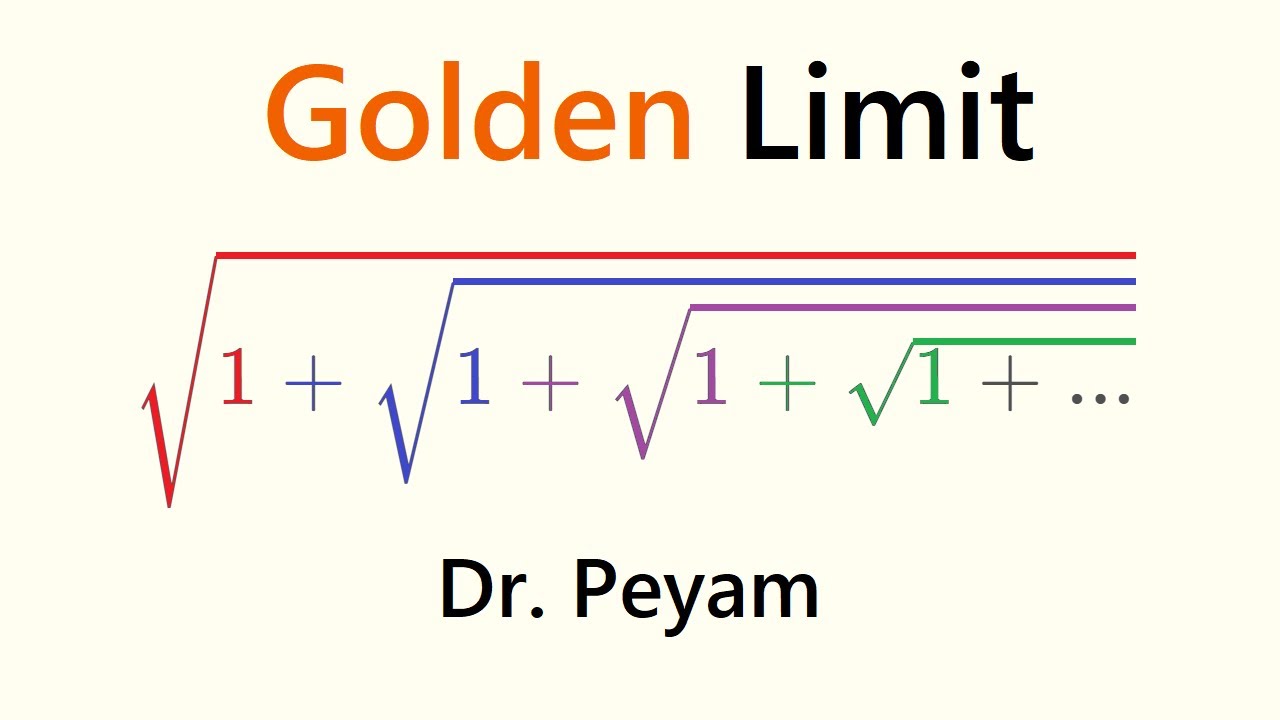
Golden limit

Limit using Monotone Sequence Theorem

I am back from summer vacation! In this video, I calculate an interesting limit using the monotone sequence theorem. It might have to do with bunnies, who knows? :)

[Galileosays]

Nice proof and example. Another series to play with: S(n+1)=ln(S(n)+a), with S(n=1)=1.It converges for a>1 to a positive value. For a=1 it converges to zero.

[libardouribe]

Me gustó esta forma de encontrar el límite de esa sucesión continua.👏

[pablorestrepodiaz]

Hi peyam, soy de colombia y me gustan mucho tus videos, i also speak english

[sebmata]

Dr. Peyam, I see the reasoning behind your steps in 7:40, but it doesn't seem very formal. Do you have any hints for a more formal way to show that the golden ratio is the limit of the sequence? I've been breaking my head over this problem for the past two days.

[plaustrarius]

Welcome back! very fun video thank you!

[deemotion]

It's was amazing. Thank you so much for this Nice prove.

[Vannishn]

Wooo spooky nice ! 😁 Didn't know about that !

[josephascencio-parvy]

I was wondering if we could solve this “more simply” by studying sqt(x+sqt(x+...
We set this function equal to y. We then notice that y=sqt(x+y)
Square both sides: y^2=x+y therefore y^2-y-x=0
Here x=1 so we solve a simple quadratic and find Phi.
I guess it’s just using a parameter

[saradinduruidascst]

Sir plz help to solve this of 1/sqrt(2x^3-9x^2+12x+4)

[yassinemran]

Can you help me
F(x)+F(x+1)=1/x F(x)?

[madushansamudika]

Please name your videos from the mathematical terms, it will be helpful to people who are searching these kind of things