filmov
tv
Visualizing Taylor polynomial approximations | AP Calculus BC | Khan Academy
Показать описание
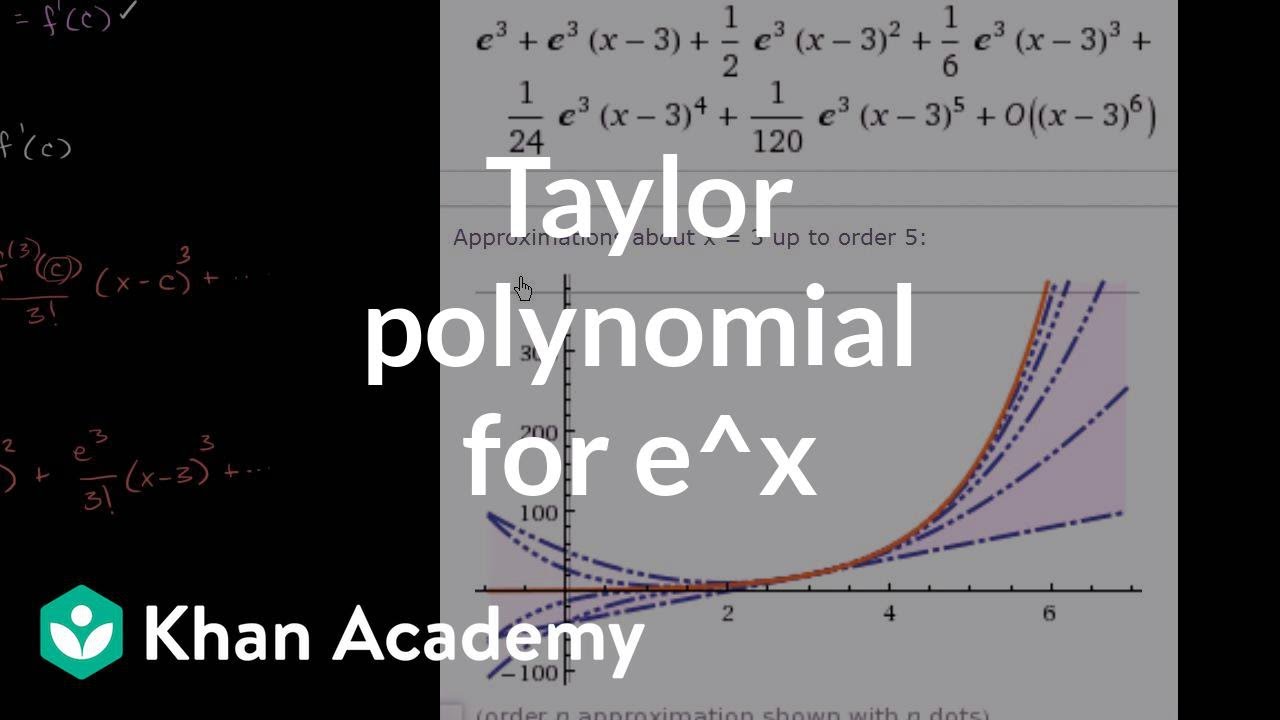
Approximating e_ with a Taylor polynomial centered at x=3. In the video we find the first few terms of such a polynomial and graph it to see how close it gets to e_. Created by Sal Khan.
AP Calculus BC on Khan Academy: Learn AP Calculus BC - everything from AP Calculus AB plus a few extra goodies, such as Taylor series, to prepare you for the AP Test
For free. For everyone. Forever. #YouCanLearnAnything
Visualizing Taylor polynomial approximations | AP Calculus BC | Khan Academy
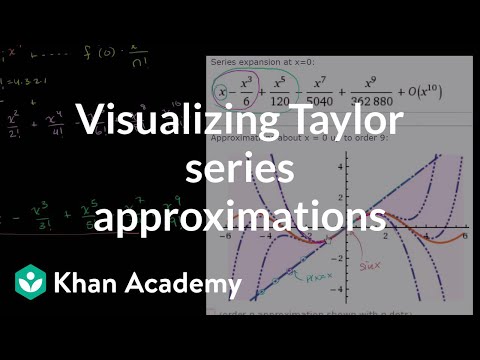
Visualizing Taylor series approximations | Series | AP Calculus BC | Khan Academy
Intro to Taylor Series: Approximations on Steroids
Taylor series | Chapter 11, Essence of calculus
Visualization of the Taylor Series
Visualizing Taylor Series Approximations
Visualizing Taylor Polynomials
Dear Calculus 2 Students, This is why you're learning Taylor Series
Visualization of Taylor/Maclaurin series approximation
Taylor Polynomial Dance
Visualization of the universal approximation theorem
Taylor Swift explains the Taylor series in 90 seconds
The Intuition Behind Taylor Series: Visualizing Function Approximations via Graphs and Programming
Taylor Series Approximation Animation.
Taylor Series and Power Series Made Easy (with Pictures): Review of Calculus
Visualisation of Taylor Series
Math Integral Calculus - Visualizing Taylor polynomial approximations
Calculus BC – 10.11 Finding Taylor Polynomial Approximations of Functions
M4ML - Multivariate Calculus - 4.8 Multivariate Taylor
When mathematicians get bored (ep1)
How REAL Men Integrate Functions
Taylor & Maclaurin polynomials intro (part 1) | Series | AP Calculus BC | Khan Academy
Taylor Polynomials : A Graphical Look at What They Do
Visualizing Taylor Polynomials Using Maplesoft
Комментарии
 0:06:38
0:06:38
 0:06:01
0:06:01
 0:12:43
0:12:43
 0:22:20
0:22:20
 0:00:35
0:00:35
 0:06:01
0:06:01
 0:08:03
0:08:03
 0:12:36
0:12:36
 0:03:35
0:03:35
 0:00:15
0:00:15
 0:00:31
0:00:31
 0:01:29
0:01:29
 0:08:04
0:08:04
 0:01:02
0:01:02
 0:28:55
0:28:55
 0:01:37
0:01:37
 0:06:38
0:06:38
 0:19:46
0:19:46
 0:06:28
0:06:28
 0:00:37
0:00:37
 0:00:35
0:00:35
 0:12:59
0:12:59
 0:06:55
0:06:55
 0:13:26
0:13:26