filmov
tv
An Application of Fermat's Last Theorem

Показать описание
Can you find all integers n such that n+9 and n^2+27 are both cube numbers? The solution to this problem uses Fermat's Last Theorem in a really cool way exhibiting that Fermat's Last Theorem has real world applications.
Follow me on Instagram: @jpimaths
And, as always, any comments, feedback or suggestions are welcomed!
Thanks.
PS. I'm also available for private tutoring! As someone who has successfully studied maths at Oxford University, I can help you in achieving your dreams of studying maths at a top university. I have over 2 years of experience tutoring maths to prospective Oxbridge students! Email me using the email address above to get more details!
Follow me on Instagram: @jpimaths
And, as always, any comments, feedback or suggestions are welcomed!
Thanks.
PS. I'm also available for private tutoring! As someone who has successfully studied maths at Oxford University, I can help you in achieving your dreams of studying maths at a top university. I have over 2 years of experience tutoring maths to prospective Oxbridge students! Email me using the email address above to get more details!
Proving Fermat' s Last Theorem (almost) in just 2 minutes !
What is Fermat's Last Theorem?
Fermat's Last Theorem - Numberphile
An Application of Fermat's Last Theorem
Elliptic Curves and Modular Forms | The Proof of Fermat’s Last Theorem
The Heart of Fermat's Last Theorem - Numberphile
What Is Fermat's Last Theorem?
Fermat's Last Theorem
Fermat's Theorem (Part 4, Burton)
The Bridges to Fermat's Last Theorem - Numberphile
Fermat's Theorem - Application of Derivatives - Calculus 1
Mathematicians explains Fermat's Last Theorem | Edward Frenkel and Lex Fridman
Fermat's Last Theorem solved
Pingu reacting to proof of Fermat's Last Theorem
How Andrew Wiles proved Fermat's Last Theorem
Ramanujan, 1729 and Fermat's Last Theorem
Applying Fermat's Last Theorem: Proving 2^(1/n) Is Irrational
360 Years To Prove Fermat's Last Theorem | A Historic Breakthrough | #Shorts | Infinity Learn J...
Fermat's Last Theorem
Fermat's Little Theorem
How did Fermat Inspire Newton? #shorts
Fermat's Last Theorem - Book Review
Andrew Wiles: Fermat's Last theorem: abelian and non-abelian approaches
Proving weird Fermat's Last Theorem in just 2 minutes !
Комментарии
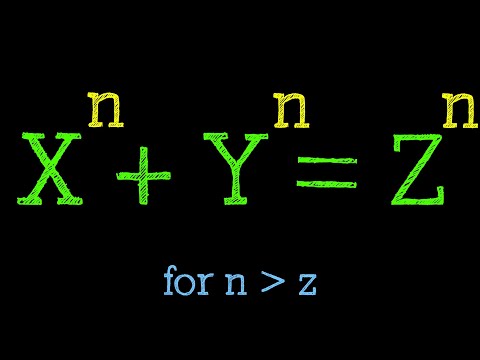 0:02:00
0:02:00
 0:01:44
0:01:44
 0:09:31
0:09:31
 0:05:47
0:05:47
 0:10:14
0:10:14
 0:09:28
0:09:28
 0:00:55
0:00:55
 0:00:48
0:00:48
 0:23:51
0:23:51
 0:27:53
0:27:53
 0:05:03
0:05:03
 0:15:14
0:15:14
 0:01:09
0:01:09
 0:00:10
0:00:10
 0:01:00
0:01:00
 0:16:48
0:16:48
 0:00:57
0:00:57
 0:00:47
0:00:47
 0:00:09
0:00:09
 0:07:31
0:07:31
 0:00:20
0:00:20
 0:05:22
0:05:22
 0:53:12
0:53:12
 0:02:00
0:02:00