Acceleration of unsteady PDE constrained optimization under PETSC/TAO

Oana Marin, Emil Constantinescu and Barry Smith

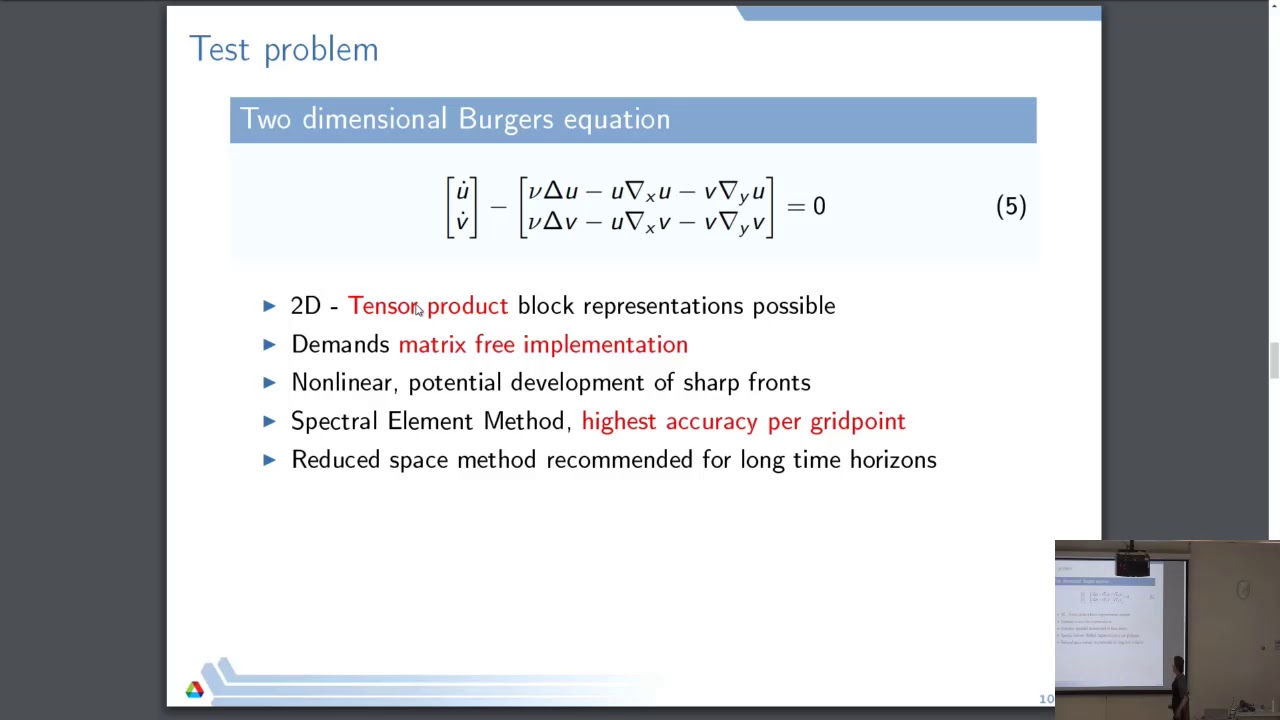
The optimization of problems governed by partial differential equations is inherently computationally expensive. If the original simulation requires a number of N timesteps then the optimization requires $2Nm$, where $m$ is the total number of iterations up to convergence towards a local mininum/maximum. It is therefore crucial to operate at the limit of accuracy and efficiency. In this regards we explore the impact of matrix-free highly vectorized spatial discretizations, checkpoint reduction, and multigrid approaches. The problem under consideration, two-dimensional Burger's equation, encompasses all the difficulties that cripple PDE-constrained optimization problems, such as ill-conditioning, lack of global extremum, restrictive stability bounds as well as a strong coupling between forward and adjoint solves.