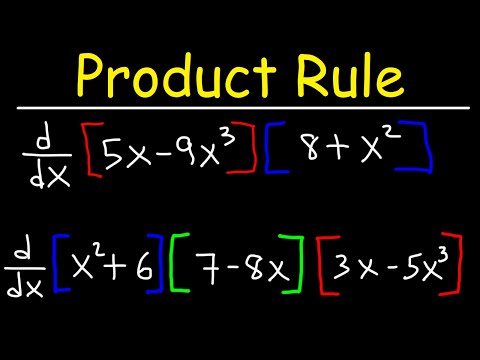
Use the product rule to differentiate 5x(2x+1)^3

Welcome to our YouTube video on using the product rule to differentiate 5x(2x+1)^3!

In this video, we will walk you through step-by-step on how to use the product rule to differentiate this function. The product rule is a fundamental rule of differentiation that allows us to find the derivative of the product of two functions.

Before we dive into the process of differentiating the function 5x(2x+1)^3, let’s review the product rule. The product rule states that if we have two functions, f(x) and g(x), their product, h(x) = f(x)g(x), can be differentiated using the following formula:

h'(x) = f'(x)g(x) + f(x)g'(x)

where f'(x) and g'(x) represent the derivatives of f(x) and g(x), respectively.

Now, let’s apply the product rule to differentiate 5x(2x+1)^3. To do this, we first need to identify our two functions, f(x) and g(x). In this case, we have:

f(x) = 5x
g(x) = (2x+1)^3

Next, we need to find the derivatives of f(x) and g(x), which we can do using the power rule and the chain rule, respectively. The power rule tells us that the derivative of x^n is nx^(n-1), while the chain rule tells us how to differentiate composite functions.

So, we have:

f'(x) = 5 (using the power rule)
g'(x) = 3(2x+1)^2(2) (using the chain rule)

Now that we have our two functions and their derivatives, we can plug them into the product rule formula:

h'(x) = f'(x)g(x) + f(x)g'(x)

h'(x) = 5(2x+1)^3 + 5x[3(2x+1)^2(2)]

h'(x) = 5(2x+1)^3 + 30x(2x+1)^2

And there you have it – the derivative of 5x(2x+1)^3 using the product rule!

Now that we have found the derivative of this function, we can use it to answer a variety of questions. For example, we can find critical points, determine intervals of increase and decrease, and identify local extrema. We can also use the derivative to sketch the graph of the original function and determine its concavity and points of inflection.

In summary, the product rule is a powerful tool that allows us to find the derivative of the product of two functions. In this video, we have demonstrated how to use the product rule to differentiate the function 5x(2x+1)^3. We hope that this tutorial has been helpful in enhancing your understanding of the product rule and its applications in calculus. Thank you for watching!