filmov
tv
The Magic Number➖➕by Howard Thurston #mentalism

Показать описание
The Magic Number➖➕by Howard Thurston #mentalism
The magic numberby howard thurston mentalism
The magic number ➖➕ by howard Thurston #mentalism #shorts #shortfeed #youtubeshorts
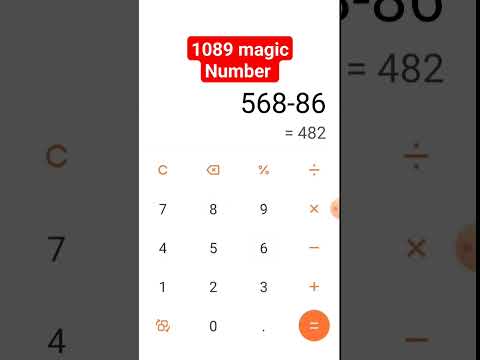
Magic number -, + 1089 universal magic number #viral #shortsvideo #trending #mathstricks
The Other Side Of Magic S01 E01 - Howard Thurston
Howard Thurston - How a boy who ran away from home became the World’s Greatest Magician? | Biography...
The Magic of Frank Thurston
Cut in half in public 🤭
Magic Question: What are the 'RULES' of Performing Magic?
Magic Made Easy and 400 Tricks You Can Do
A Card Trick With Invisible Cards?! #shorts
Magic has been a popular form of entertainment for centuries. In 1900
The Life and Work of Jim Steinmeyer to this point
Magic compilation tiktok Videos. #shorts
POV: you’re 6’9” 400 pounds and booked the middle seat
Magic Trick in top world#pakistan#india#indianarmy#pakistani#shorts#ytshorts
Simple but BRILLIANT Card Trick
The Hex System, Magick and Spell Casting
cheer comp #cheer #2023 #athlete
The Last Greatest Magician in The World by Jim Steinmeyer | Book Club
“I own Frankston”
Meghan Markle takes a dig at William and Kate
The Golden Age of Magic
A Beautiful Piece of Magic by Dai Vernon. Easy, Self-Working and Beginner-Friendly, Yet So Powerful.
Комментарии
 0:00:45
0:00:45
 0:00:50
0:00:50
 0:01:01
0:01:01
 0:00:36
0:00:36
 0:06:43
0:06:43
 0:05:58
0:05:58
 0:02:21
0:02:21
 0:00:59
0:00:59
 0:08:20
0:08:20
 0:15:30
0:15:30
 0:01:00
0:01:00
 0:01:03
0:01:03
 0:22:00
0:22:00
 0:00:25
0:00:25
 0:00:18
0:00:18
 0:00:15
0:00:15
 0:01:00
0:01:00
 0:27:27
0:27:27
 0:00:11
0:00:11
 1:19:30
1:19:30
 0:00:15
0:00:15
 0:00:39
0:00:39
 0:17:23
0:17:23
 0:03:58
0:03:58