filmov
tv
Scalar Dot Product As Vector Projection Component
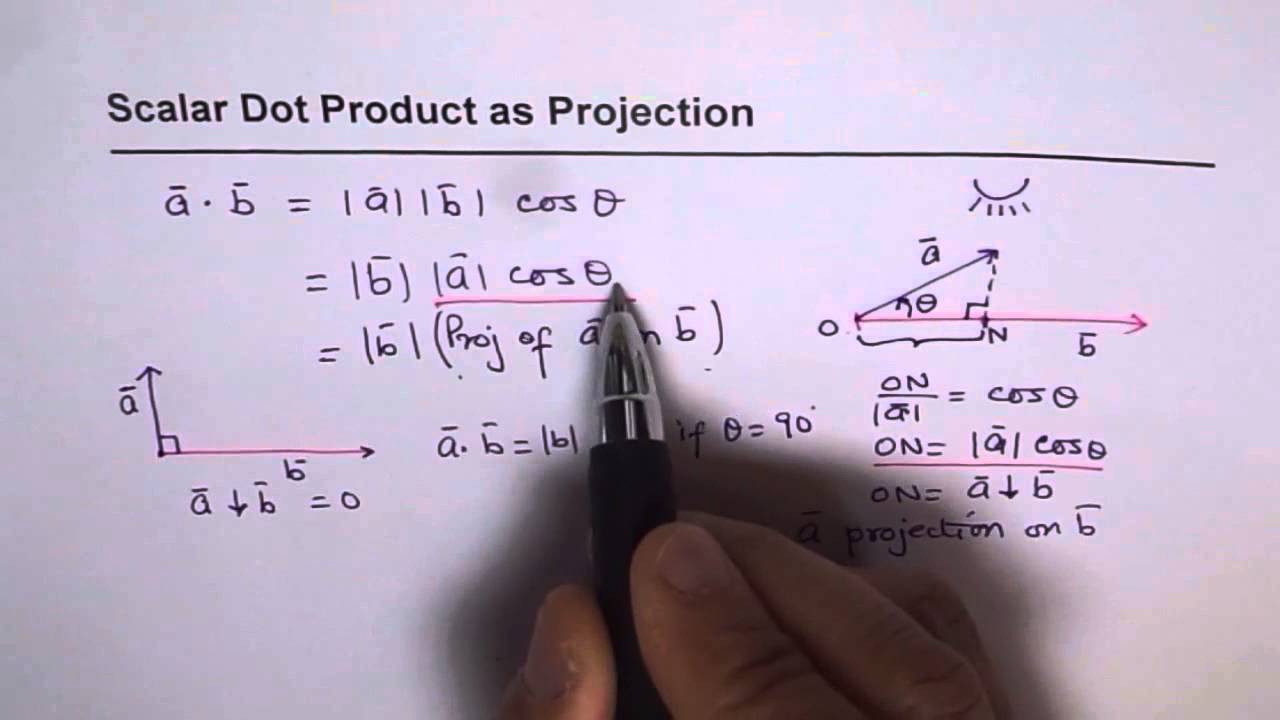
Показать описание
#vectors_MCV4U #vectors_IBmath #vectors_application #vector_geometry #MCV4U_Vectors #vectors_edexcel #vectors_gcse #vectors_dotproduct #vectors_crossproduct
The Vector Dot Product
Dot Product and Scalar Product of Vectors, Physics
Dot products and duality | Chapter 9, Essence of linear algebra
Class 11 Chapter 4 : VECTOR 05 :SCALAR PRODUCT OF VECTORS|| DOT PRODUCT OF VECTORS ||
Calculus 3 - The Dot Product
Cross Product and Dot Product: Visual explanation
Scalar Product or Dot Product between Vectors || Multiplication of vectors || Dot or Cross Product
Vectors 09 : Scalar Product Or Dot Product of Vectors
TRICK OF SCALAR PRODUCT #maths #trianglelawofvectoraddition #class11maths #exam
Scalar Product | Dot Product | Product of vectors | Easier explanation | Urdu | Hindi
Vector dot product and vector length | Vectors and spaces | Linear Algebra | Khan Academy
The Dot Product - A Visual Explanation
Scalar Dot Product As Vector Projection Component
How to calculate the DOT PRODUCT of vectors (scalar product)
scalar product or dot product class 11 | scalar product class 11 | National book foundation | NBF
The real world applications of the dot product
Dot Product or Scalar Product of Two Vectors - Motion in a Plane | Class 11 Physics
Scalar Product (Dot Product) | National Book Foundation | NBF | Class 11th Physics CH No 02
Scalar Product of Unit Vectors
The meaning of the dot product | Linear algebra makes sense
Scalar or dot product unit 2 kinematic class 11 New physics book | Product of two vectors chapter 2
Cross and Dot Product NEET 2024/25 | Vectors class 11th and Droppers
The Vector Cross Product
Scalar product | dot product | class 11 physics | Mechanics | lecture 1 | BS physics | BSc
Комментарии
 0:06:59
0:06:59
 0:10:35
0:10:35
 0:14:12
0:14:12
 0:33:00
0:33:00
 0:13:34
0:13:34
 0:04:08
0:04:08
 0:07:12
0:07:12
 1:53:18
1:53:18
 0:00:37
0:00:37
 0:30:30
0:30:30
 0:09:10
0:09:10
 0:01:50
0:01:50
 0:09:20
0:09:20
 0:01:44
0:01:44
 0:28:54
0:28:54
 0:12:49
0:12:49
 0:11:31
0:11:31
 0:24:25
0:24:25
 0:02:13
0:02:13
 0:13:04
0:13:04
 0:25:03
0:25:03
 0:22:41
0:22:41
 0:06:46
0:06:46
 0:10:37
0:10:37