filmov
tv
Arc Length of A Polar Curve (proof)

Показать описание
Arc Length of A Polar Curve,
Calculus 2
Calculus 2
Arc Length of Polar Curves
Arc Length of A Polar Curve (proof)
Arc Length of a Polar Curve
Deriving the arc length of a Polar Equation in 4 minutes (with examples!)
Arc Length of Polar Curves | Calculus 2 Lesson 51 - JK Math
POLAR ARC LENGTH
Area and Arc Length for Polar Curves
Arc Length of a Polar Curve
E&M Reading Group Ch. 9 Part 2, Arc Length in Polar Coordinates, Tangents to y=x^3 Through (4,0)
Calculus-Finding Arc Length of Polar Curves
Ex 2: Arc Length of a Polar Curve
Derivation and Example of Arc length for Polar Form of an Equation
Polar Parametric Curve - Arc Length (KristaKingMath)
Finding the Arc Length of a Polar Curve
Arc length of polar curves
Arc length of petal of polar graph
Arc Length along Polar Curve r = e^{-O}
Arc Length of a Polar Curve (KristaKingMath)
Areas and Arc Length in Polar Coordinates
Justification for polar arc length formula
Deriving the Arc Length in Cartesian and Polar Coordinates
Arc Length in Polar Coordinates
Arc length of a polar curve (Integrals)
Set up integral for arc length of a polar curve
Комментарии
 0:10:33
0:10:33
 0:08:11
0:08:11
 0:07:38
0:07:38
 0:04:09
0:04:09
 0:39:59
0:39:59
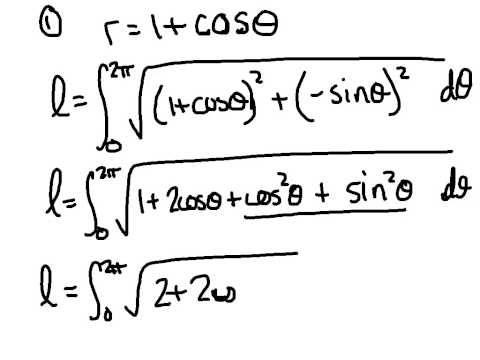 0:09:53
0:09:53
 0:07:53
0:07:53
 0:05:01
0:05:01
 5:41:01
5:41:01
 0:10:05
0:10:05
 0:07:34
0:07:34
 0:06:28
0:06:28
 0:07:04
0:07:04
 0:09:18
0:09:18
 0:09:33
0:09:33
 0:06:23
0:06:23
 0:06:06
0:06:06
 0:07:56
0:07:56
 0:41:16
0:41:16
 0:11:45
0:11:45
 0:13:48
0:13:48
 0:07:56
0:07:56
 0:02:20
0:02:20
 0:01:19
0:01:19