filmov
tv
Proof of the Chain Rule

Показать описание
In this video, I provide a neat proof of the chain rule, and I also explain why I call it the Chen Lu. Enjoy!
Chain rule proof | Derivative rules | AP Calculus AB | Khan Academy
Proof of the Chain Rule
A Proof of the Chain Rule
Visualizing the chain rule and product rule | Chapter 4, Essence of calculus
Proof of the Chain Rule
A Proof of the Chain Rule
proving the chain rule
don't use the chain rule, bro
Extremal Combinatorics Lecture 32: Shearer's Lemma and the Loomis-Whitney Inequality
Derivative Rules: Proof of Chain Rule
The Chain Rule - Intuitive Proof
Chain Rule Method of Differentiation | Derivatives
3.11 Chain rule - The theorem
Proof of Chain Rule for Differentiation
Chain Rule For Finding Derivatives
Proof of Chain Rule
An epsilon-delta proof of the chain rule
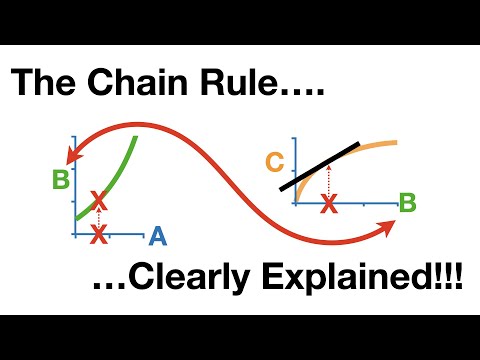
The Chain Rule
Chain Rule
An introduction to, and proof of: the chain rule
CHAIN RULE PROOF (DERIVATIVE OF COMPOSITE FUNCTION) #40
Chain rule | Derivative rules | AP Calculus AB | Khan Academy
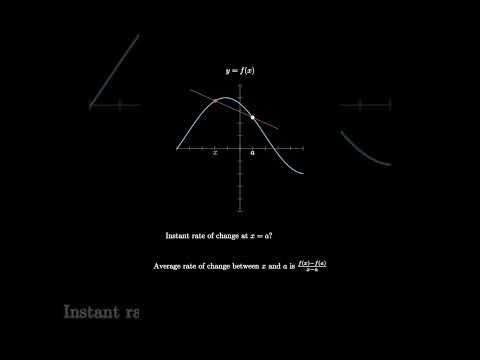
Visual Derivative Definition!
The Multi-Variable Chain Rule: Derivatives of Compositions
Комментарии
 0:05:39
0:05:39
 0:07:29
0:07:29
 0:05:58
0:05:58
 0:15:56
0:15:56
 0:16:05
0:16:05
 0:08:33
0:08:33
 0:00:56
0:00:56
 0:00:22
0:00:22
 0:46:15
0:46:15
 0:12:27
0:12:27
 0:03:36
0:03:36
 0:06:01
0:06:01
 0:09:37
0:09:37
 0:07:23
0:07:23
 0:18:40
0:18:40
 0:09:25
0:09:25
 0:16:59
0:16:59
 0:18:24
0:18:24
 0:05:27
0:05:27
 0:14:49
0:14:49
 0:07:17
0:07:17
 0:05:07
0:05:07
 0:01:00
0:01:00
 0:10:47
0:10:47