Gravitational potential energy derivation | Work & Energy | Physics | Khan Academy

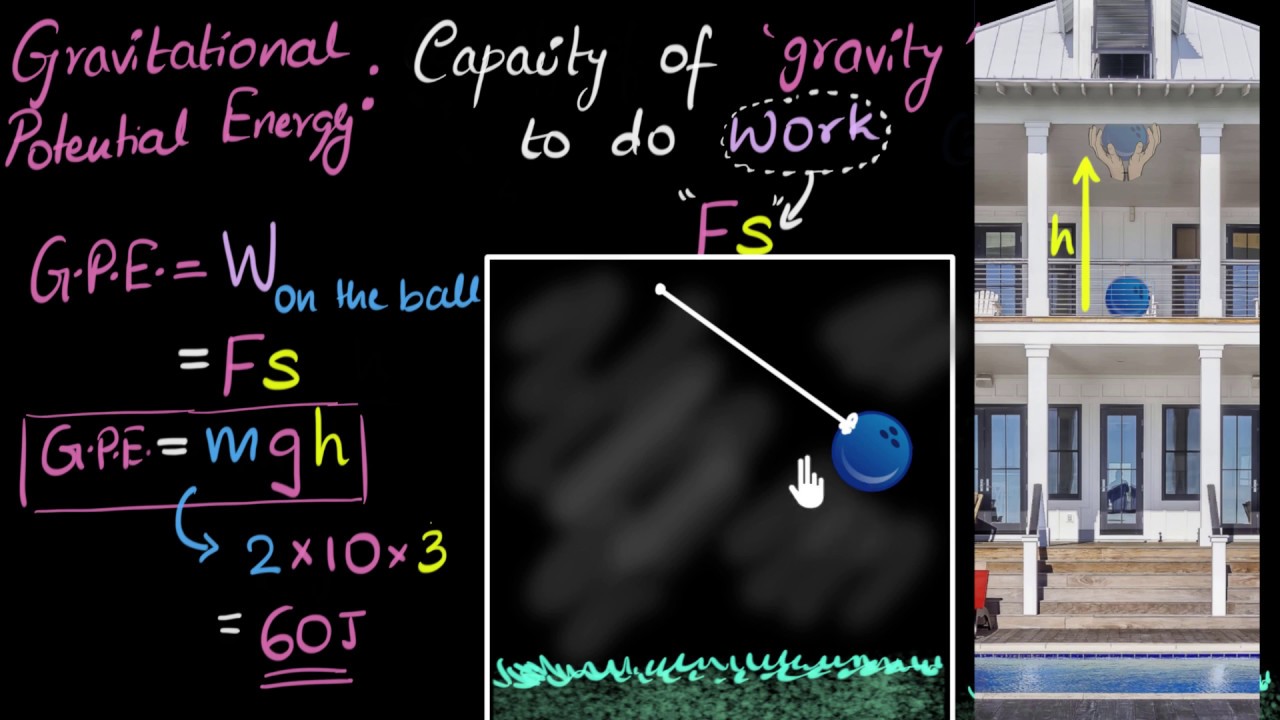
Let's derive expression for gravitational potential energy.

Khan Academy is a nonprofit organization with the mission of providing free, world-class education for anyone, anywhere. We offer quizzes, questions, instructional videos, and articles on a range of academic subjects, including math, biology, chemistry, physics, history, economics, finance, grammar, preschool learning, and more. We provide teachers with tools and data so they can help their students develop the skills, habits, and mindsets for success in school and beyond. Khan Academy has been translated into dozens of languages, and 15 million people around the globe learn on Khan Academy every month. As a 501(c)(3) nonprofit organization, we would love your help!

Created by Mahesh Shenoy

[Hino_]

Energy is the capacity to do work, it's a number that tells you how much work you can do.

Nicee, I finally understand the concept of energy and work more clearly!



Kinetic Energy = how much work a moving object can do when it hits something


Potential Energy = how much work a stationary object can do when it starts moving. Hence, it is a stored energy waiting to be kinetic energy. It has the "potential" to move or it has the potential to be kinetic.



NOTE: Kinetic energy is based on motion WHILE Potential energy is based on configuration/position.

[AbcXyz-ogcy]

Wow! That was amazing 🤩.
Thank uu...u guys just explained it like it was child's play...

[MrYahya]

h is pointing in the opposite direction to mg, so shouldn't W= F (dot) displacement=-mg*(delta h), rather than mg*(delta h), given that the dot product of vectors in opposite directions is the negative of their product?

[BappiDebnath-qohn]

Bro in my book potential energy has been said that the total work done of the ball to rise it in h hight with zero acceleration is the potential energy . What is correct plz say 😭🙏🏻

[samuelramesh]

Hi I have a question, let’s say I lift the ball of 1Kg to 1M height with a velocity of 1M/S and what if I change the velocity to move the ball vertically to 2M/S then what will be the GPE, I think the GPE will be the same irrespective of the velocity by which I move the ball vertically, I am I correct

[dakshkholia]

in last video you said that K.E = M*U^2/2 but on google it shows KE = M*V^2/2 .
plz help me out.

[samuelramesh]

@Khan Academy India
Help me understand below two cases:
1) Ball of mass 2 Kg lifted to height of 5 Meters @ velocity of 1M/s upward (so to lift this ball to 5M it should have taken 5 seconds), So how much joule of energy did I spent on this ball?
2) Ball of mass 2 Kg lifted to height of 5 Meters @ velocity of 2M/s upward (so to lift this ball to 5M it should have taken 2.5 seconds), So how much joule of energy did I spent on this ball?

I think case 2) should have taken twice the energy than the case 1 (When we let the ball drop, we get same Kinetic Energy, where as the input energy spent will be different in both the cases ).

Please let me know how to calculate the energy spent on the ball considering the time factor?