filmov
tv
Playing tricks with 2-variable limits

Показать описание
Oh hey look, a function!
0:00 Intro
1:57 Counterexamples to checking some straight lines
3:40 Counterexample to checking all straight lines
4:39 A more 'natural' counterexample (and touching grass)
6:54 Outro
The graphing program used in this video is the Grapher app that comes with Mac computers.
0:00 Intro
1:57 Counterexamples to checking some straight lines
3:40 Counterexample to checking all straight lines
4:39 A more 'natural' counterexample (and touching grass)
6:54 Outro
The graphing program used in this video is the Grapher app that comes with Mac computers.
Playing tricks with 2-variable limits
Trick to Find Limit at Infinity of Rational Functions | SHS 2 ELECTIVE MATH
Memorization Trick for Graphing Functions Part 1 | Algebra Math Hack #shorts #math #school
Finding Limits using Algebraic Trick
how you can trick calculus students with this limit
Short trick for limit
Calculus 1 - Introduction to Limits
Lesson: Algebra Tricks for Limits
Advanced Calculus: limits and continuity in normed linear spaces of finite dimension, 1-16-25 part 1
Change of Variables & The Jacobian | Multi-variable Integration
How To Find The Limit At Infinity
Indian vs Japanese Maths 🔥| Vedic Maths Trick for Fast Calculation | Speed Maths #trending #shorts...
A Limit Example Combining Multiple Algebraic Tricks
Horizontal asymptote #asymptotes #verticalshift #vertical-asymptote #tricks #shorts #mathstricks
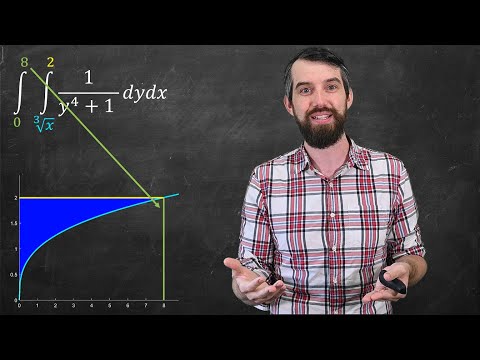
Change the order of integration to solve tricky integrals
Calculus|| Trick to find the limit of a function||finding limit without using L Hospital Rule #limit
(2/3) Quick Trick for Finding Horizontal Asymptotes! #math #algebra #mathtrick #mathematics
Differentiation short trick| #Differentiation #calculus
Evaluate [limit xsecx] best trick #shorts #maths shorts #maths tricks
3 Step Continuity Test, Discontinuity, Piecewise Functions & Limits | Calculus
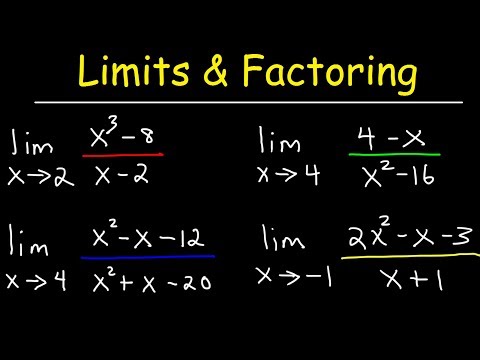
Evaluating Limits By Factoring
TRICKS you can do in SCIENTIFIC CALCULATORS🔥#viral #shorts
Amazing Exponential Trick. #viralmathshorts #mathshort
#Trick | limits | Continue| Simultaneous Limit| Calculus| #mastercadre #PGTmaths #Shorts #PGTmaths
Комментарии
 0:07:49
0:07:49
 0:03:20
0:03:20
 0:00:15
0:00:15
 0:10:30
0:10:30
 0:03:15
0:03:15
 0:00:16
0:00:16
 0:20:20
0:20:20
 0:16:10
0:16:10
 0:59:51
0:59:51
 0:10:07
0:10:07
 0:13:14
0:13:14
 0:00:13
0:00:13
 0:07:23
0:07:23
 0:00:13
0:00:13
 0:07:39
0:07:39
 0:01:01
0:01:01
 0:00:31
0:00:31
 0:00:42
0:00:42
![Evaluate [limit xsecx]](https://i.ytimg.com/vi/ubs7kTg-zXg/hqdefault.jpg) 0:00:16
0:00:16
 0:10:10
0:10:10
 0:11:35
0:11:35
 0:00:25
0:00:25
 0:01:00
0:01:00
 0:01:00
0:01:00