filmov
tv
The biggest MYTH in math 😳
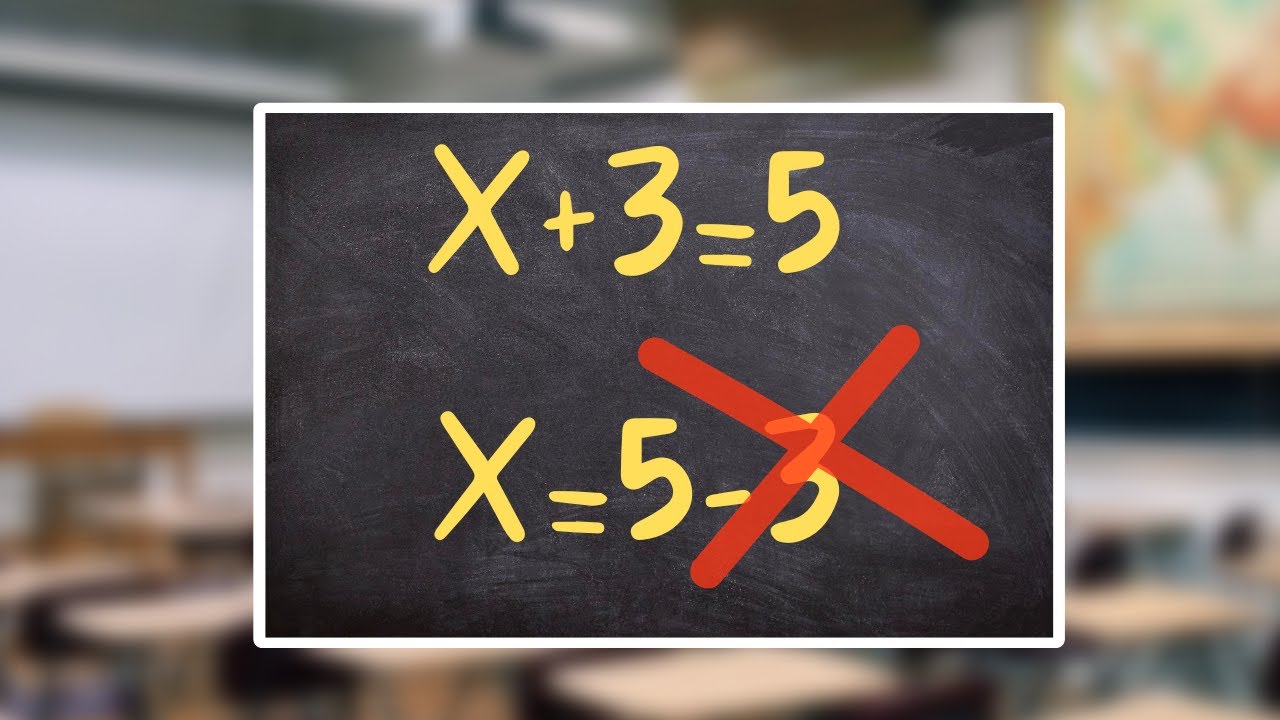
Показать описание
99% of students and teachers still think this is true. Here's the truth instead.
================================================
Follow me at
================================================
Support me and learn more physics at the same time by enrolling in my Udemy course
================================================
================================================
Follow me at
================================================
Support me and learn more physics at the same time by enrolling in my Udemy course
================================================
The biggest MYTH in math 😳
The Biggest Myth In Education
Busting the biggest myth of number sequences | The next term #math #maths
The Biggest Myth in Actuarial Exam Prep!
The Biggest Myth of all 😲 | #maths #education #shorts
The Biggest Myth in Speedrunning History
The myth of the 'maths person'
The Man, the Myth, the Legend: Scott Sterling
Mathematics in the digital world
The Math People Myth | Nate Brown | TEDxPSU
The Math Myth in Machine Learning... Again and Again
Myth-busting Mathematics | Professor Kate Smith-Miles
Challenges in Math #6 - Myth that Faster is Better
'bad at math' is a myth
Einstein and the Math Myth #einstein #maths
Busting the myth!/Did Albert Einstein had really failed in his maths test?
Making Sense of Math: The New Math Myth
The Golden Ratio: Is It Myth or Math?
Pythagoras Unplugged: The Man, The Myth, The Math
The myth and the fact: 🙄 👀 Albert Einstein failed math class.
Albert Einstein failed in math - myth debunking | #shorts #viral #myths #facts
Breaking The Myth of Inborn Mathematical Ability. | James Juma | TEDxYouth@Upanga
Sexist Math Myth Debunked by Science!
The Biggest Myth In Education #1
Комментарии
 0:05:12
0:05:12
 0:14:27
0:14:27
 0:04:53
0:04:53
 0:00:40
0:00:40
 0:00:56
0:00:56
 0:19:37
0:19:37
 0:01:40
0:01:40
 0:00:28
0:00:28
 2:02:56
2:02:56
 0:12:31
0:12:31
 0:02:05
0:02:05
 0:17:17
0:17:17
 0:03:44
0:03:44
 0:11:59
0:11:59
 0:00:47
0:00:47
 0:00:16
0:00:16
 0:02:35
0:02:35
 0:22:55
0:22:55
 0:02:38
0:02:38
 0:00:57
0:00:57
 0:01:00
0:01:00
 0:17:57
0:17:57
 0:01:51
0:01:51
 0:00:59
0:00:59