filmov
tv
4. Spin One-half, Bras, Kets, and Operators
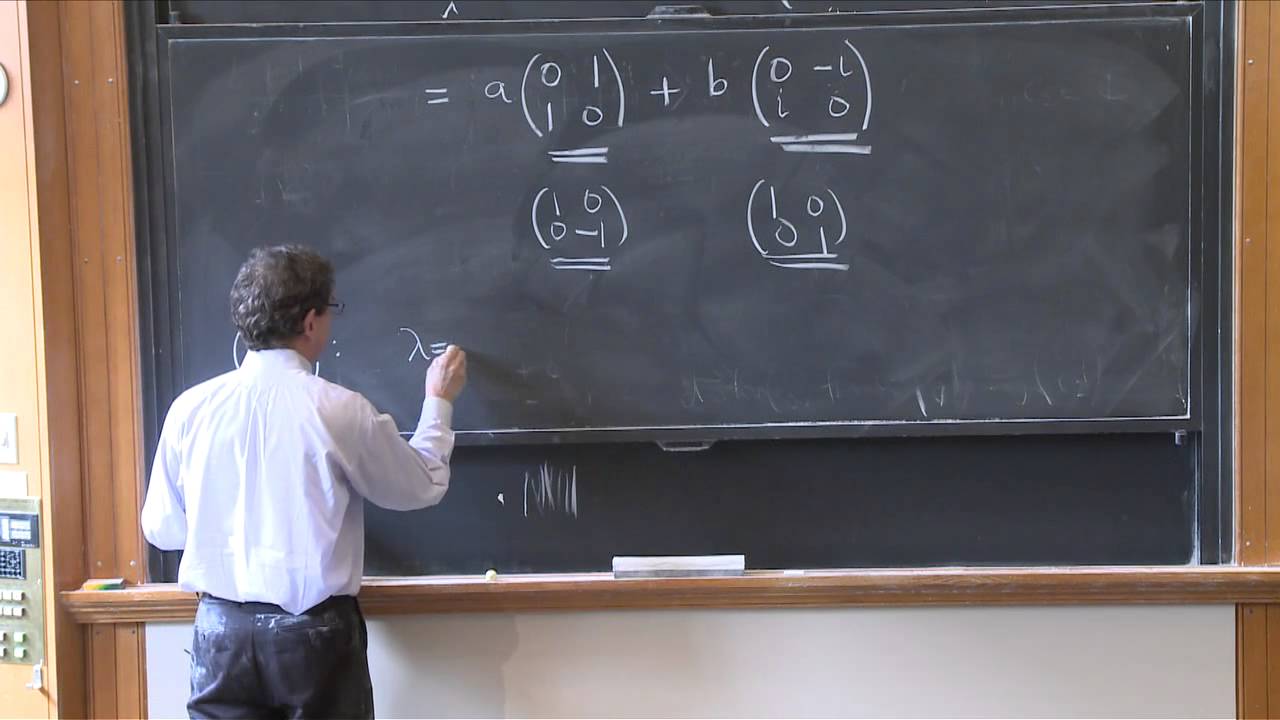
Показать описание
MIT 8.05 Quantum Physics II, Fall 2013
Instructor: Barton Zwiebach
In this lecture, the professor talked about spin one-half states and operators, properties of Pauli matrices and index notation, spin states in arbitrary direction, etc.
License: Creative Commons BY-NC-SA
Instructor: Barton Zwiebach
In this lecture, the professor talked about spin one-half states and operators, properties of Pauli matrices and index notation, spin states in arbitrary direction, etc.
License: Creative Commons BY-NC-SA
4. Spin One-half, Bras, Kets, and Operators
Ch 6: What are bras and bra-ket notation? | Maths of Quantum Mechanics
Ket States - Dirac notation for spin-1/2
Bra-Ket Formalismus (inneres Produkt berechnen)?!
Probability in bra-ket Example Calculation
Basic approach of calculating probability in bra-ket notation
Bra Ket Notation | Quantum Mechanics
Quantum probability calculations for a spin-1 state
Bra-ket notation - Spin-1 system (brief intro)
34. Bra–ket notation
Bra-ket notation - Brief example of possible results and probabilities (Spin-1 system)
1. Bras, Kets And Operators | Weinberg’s Lectures on Quantum Mechanics
Spin in Quantum Mechanics: What Is It and Why Are Electrons Spin 1/2? Physics Basics
Inner product and Dirac Notation | Quantum Mechanics | LetThereBeMath |
Problem 1-10 find THE Probability of Spin along x y and z axes using Bra Ket Dirac Notations
4.14 Adding spins
Bra-ket notation - Measurement of the spin component Sx
9. Dirac's Bra and Ket Notation
The Pauli matrices
Lecture 4-2, Ch 5 Part 2: Bra Ket Operations 1 and Vector Normalization
Bra-Ket Operators | Quantum Mechanics
Quantum Spin: Triplet and Singlet states
The biggest misconception about spin 1/2
CompChem: Relativistic: 4. Pauli spin matrices
Комментарии
 1:24:32
1:24:32
 0:10:03
0:10:03
 0:06:59
0:06:59
 0:05:32
0:05:32
 0:07:27
0:07:27
 0:06:47
0:06:47
 0:02:07
0:02:07
 0:19:31
0:19:31
 0:12:20
0:12:20
 0:09:44
0:09:44
 0:02:54
0:02:54
 1:11:37
1:11:37
 0:11:52
0:11:52
 0:24:51
0:24:51
 0:21:16
0:21:16
 0:35:11
0:35:11
 0:08:34
0:08:34
 1:20:53
1:20:53
 0:16:58
0:16:58
 0:19:59
0:19:59
 0:02:04
0:02:04
 0:07:00
0:07:00
 0:34:06
0:34:06
 0:09:59
0:09:59