filmov
tv
The biggest misconception about spin 1/2
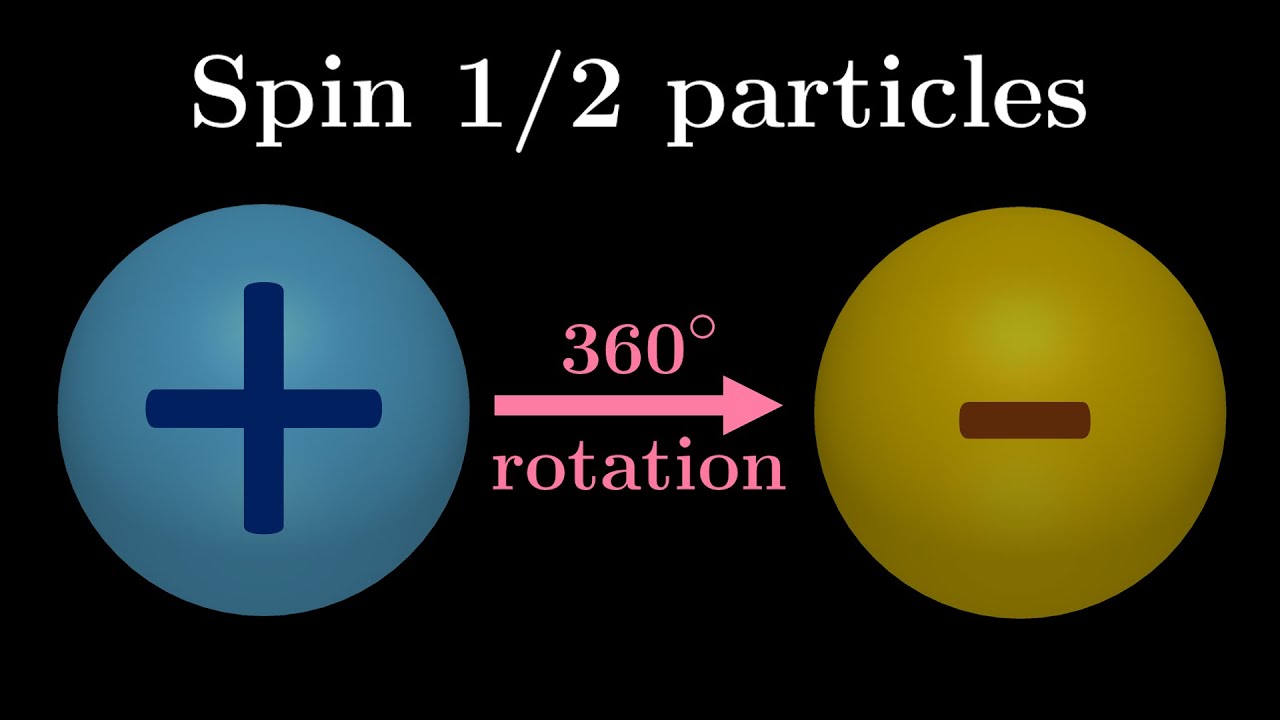
Показать описание
“If you rotate a spin 1/2 particle by 360 degrees, it doesn’t go back to its original state, rather you need 720 degrees”. This is only technically correct if you interpret the words “rotate” and “state” in the way it’s intended, and here is the video on what they really mean, and what this sentence is saying.
----------------------
Many people have talked about spin 1/2 before, but I do want to chime in because there are a few things that I myself am not satisfied with all the explanations.
(1) I personally don’t like **any** “demonstration” of spin 1/2, aimed at demonstrating in some physical situations you need two full rotations to get back to where you started, like the Dirac belt trick / spinning your hand trick. This is simply because physically, you literally can’t tell the difference before and after rotation - even in principle. You can only tell the difference when you have a superposition. And these demonstrations, to me, give the false perception that physically there is something different. This is, I think, an extremely important point that people miss out when talking about spin 1/2 and how “weird” this is.
(2) From U(2) to SU(2): the “usual” explanation for the choice of determinant is that the phase factor does not matter, so in passing from U(2) to SU(2), we sort of “remove the redundancies'' in the description of our transformation. But why did we leave the ±1 factor redundancy untouched? Either you remove **every** redundancy, or **none**. It doesn’t make sense (at least to me) that you don’t also remove that sign redundancy as well.
This is actually because the projective representations (obtained using Lie algebra methods) of SO(3) **must** have determinant 1, and this is known prior to constructing such a (projective) representation. This might be briefly explained if I decide to make a video on Lie algebras / groups / representations. I think that using the Lie algebra method means that we are imposing the constraint about analyticity and genuine representation in the neighbourhood of the identity, but I can’t be sure about this.
---------------------
---------------------------------
In a similar vein, people often think that spin-s particles are just particles that magically goes back to its original state after 2pi / s rotations. Even Wikipedia says this! Except this is not even technically correct - it is just plain wrong. I’ll talk a bit about that, again if I decide to make a video about higher spins / Lie groups / algebras / representations.
Hopf fibration / quaternions:
Video chapters:
00:00 Introduction
00:47 Chapter 1: "State"
07:42 Chapter 2: "Rotate"
17:46 Chapter 3: The construction
25:41 Chapter 4: The "spin-1/2 property"
Other than commenting on the video, you are very welcome to fill in a Google form linked below, which helps me make better videos by catering for your math levels:
If you want to know more interesting Mathematics, stay tuned for the next video!
SUBSCRIBE and see you in the next video!
If you are wondering how I made all these videos, even though it is stylistically similar to 3Blue1Brown, I don't use his animation engine Manim, but I use PowerPoint, GeoGebra, and (sometimes) Mathematica to produce the videos.
Social media:
For my contact email, check my About page on a PC.
See you next time!
Комментарии
 0:34:06
0:34:06
 0:14:48
0:14:48
 0:15:20
0:15:20
 0:09:21
0:09:21
 0:08:02
0:08:02
 0:18:10
0:18:10
 0:00:42
0:00:42
 0:04:02
0:04:02
 0:09:05
0:09:05
 0:02:22
0:02:22
 0:00:11
0:00:11
 0:00:56
0:00:56
 0:00:55
0:00:55
 0:01:00
0:01:00
 0:04:16
0:04:16
 0:01:00
0:01:00
 0:00:34
0:00:34
 0:13:16
0:13:16
 0:11:30
0:11:30
 0:29:27
0:29:27
 0:00:11
0:00:11
 0:10:09
0:10:09
 0:00:15
0:00:15
 0:00:52
0:00:52