Superposition and the Wronskian - Ordinary Differential Equations | Lecture 13

preview_player
Показать описание
In this lecture we introduce the basic existence and uniqueness theory for linear second order differential equations. We also present the principle of superposition, which then leads to the Wronskian. We show that with the Wronskian we can identify if two solutions form a fundamental solution set, thus giving us two solutions that can be used to satisfy any initial conditions.

This course is taught by Jason Bramburger for Concordia University.

Follow @jbramburger7 on Twitter for updates.
  1. Jason Bramburger
  2. differential equation
  3. ODE
  4. ordinary differential equation
  5. second order
  6. wronskian
Рекомендации по теме
Superposition and the 0:31:16

Superposition and the Wronskian - Ordinary Differential Equations | Lecture 13

Linear Independence of 0:09:59

Linear Independence of Functions & The Wronskian

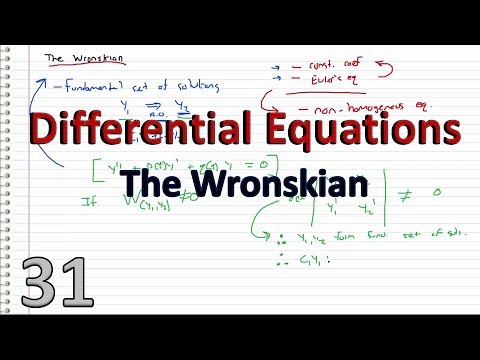
Differential Equations - 0:06:49

Differential Equations - 31 - The Wronskian

🔵19 - Fundamental 0:26:33

🔵19 - Fundamental Sets of Solution and Wronskian, Principle of Superposition of Differential Equ&apo...

The Wronskian for 0:03:09

The Wronskian for systems of differential equations

The Theory of 0:11:19

The Theory of 2nd Order ODEs // Existence & Uniqueness, Superposition, & Linear Independence

Second Order Linear 0:06:02

Second Order Linear ODEs Superposition of Solutions

ODE | Principle 0:04:18

ODE | Principle of superposition

Differential Equations - 0:35:58

Differential Equations - 4.1 Higher Order Linear ODEs (Lecture Part 2 of 2)

The Wronskian | 0:08:13

The Wronskian | Lecture 12 | Differential Equations for Engineers

Superposition Principle and 0:05:37

Superposition Principle and Wronskian in Linear Algebra and Geometry

DEqns: L6, linear 0:59:51

DEqns: L6, linear independence and the wronskian, part 1, 9-15-16

Lecture 10: The 0:46:13

Lecture 10: The Wronskian

Section 3.2: Solutions 0:27:04

Section 3.2: Solutions of Linear Homogeneous Equations (Part 2, Superposition Principle, Wronskian)

Linear operators. Understanding 1:03:05

Linear operators. Understanding the Wronskian

Linear Dependence and 0:38:47

Linear Dependence and Linear Independence of Functions, Wronskian, Superposition Principle

Superposition Principle, Wronskian 0:20:31

Superposition Principle, Wronskian

superposition 0:30:07

superposition

Differential Equations: annihilator 0:59:51

Differential Equations: annihilator method, superposition, 6-15-17, part 2

Prerequisite Lecture 3: 0:30:05

Prerequisite Lecture 3: Second Order Linear Homogeneous Equations and the Wronskian

superposition principle,linear independent&dependent,Basis,Wronskian 0:21:58

superposition principle,linear independent&dependent,Basis,Wronskian problems

The Wronskian Formula 0:08:04

The Wronskian Formula for a Particular Solution

Homogeneous Linear Differential 0:04:16

Homogeneous Linear Differential Equations & Principle of Superposition

Math 261 - 0:31:33

Math 261 - 3.2 - Solutions of Linear Homogeneous Equations; The Wronskian

Комментарии
Автор

Love the videos so far. Keep them coming

jaredvv