💯 Finding Specific Terms by a General Term in Binomial Expansion, Watch this video!

🌟 Enhance Your Understanding with Our Free Slide Download! 🌟

Did you love this video? Dive deeper by downloading the slides used in our lesson—for FREE!

Why download?

Quickly review key concepts anytime.
Deepen your knowledge with detailed explanations and visuals.
Study on the go—easy access from any device.

Remember to like, comment, and subscribe for more resources. Share with friends who’d love this, too!

📢 Receive Free Study Resources and Special Offers

Download Slide Files:

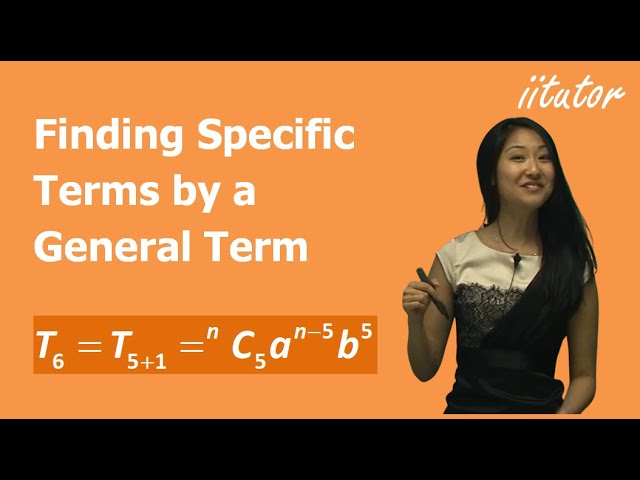
To find a specific term in a binomial expansion, you can use the general term formula, which is given by:

C(n, k) * x^k * y^(n-k)

where C(n, k) is the binomial coefficient, n is the binomial degree, k is the exponent of x, n-k is the exponent of y, and x^k and y^(n-k) are the terms of the expansion.

The binomial coefficient C(n, k) can be calculated using the formula:

C(n, k) = n! / (k! (n-k)!)

where n! is n factorial (the product of all positive integers up to n), and k! and (n-k)! are the factorials of k and n-k, respectively.

For example, to find the coefficient and the term of x^3 in the expansion of (x + y)^5, we would use the general term and the binomial coefficient formula to calculate C(5, 3) as follows:

C(5, 3) = 5! / (3! (5-3)!) = 5! / (3! 2!) = (5 * 4 * 3 * 2 * 1) / (3 * 2 * 1 * 2 * 1) = 10

So the coefficient of the term x^3 in the expansion of (x + y)^5 is 10, and the term itself is:

10 * x^3 * y^(5-3) = 10x^3y^2