Fixed Point Iteration Method Example 1 | Numerical Methods

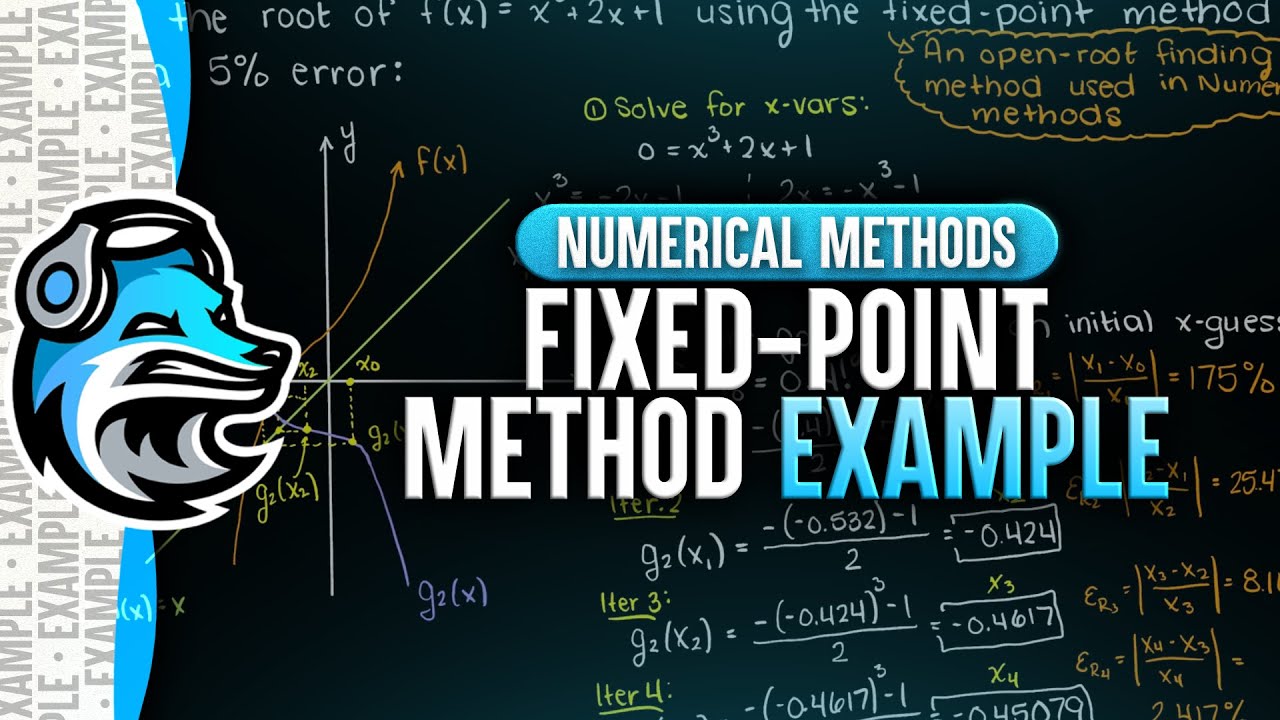
In this fixed point Iteration method example video, we will solve for the root of the function f(x) = x^3+2x+1, using the open root solving method, fixed point Iteration method (or fixed point iteration).

This timeline is meant to help you better understand how to solve a fixed point Iteration method example:
0:00 Introduction
0:27 Solving a problem using fixed point method.
3:38 Outro

Relevant Numerical Methods Playlists:

Follow & Support StudySession:

This video is part of our Numerical Methods course. Numerical methods is about solving math problems through approximating the solution of problems that would be difficult or impossible to solve analytically. In this playlist we will cover topics such as solving systems of linear equations, solving systems of non-linear equations, numerical integration, numerical derivatives, etc..
#studysession #fixedpoint #numericalmethods

[lucascruz]

Excellent, very helpful video! Thank you.

[JamesKevinAmpah-Bennin]

please why did you use 0.4 as initial guess?

[maharanirani]

Is there any rules in determining the initial value of x_o? Or we can choose it arbitrarily? Great video anyways!!

[feebleh]

Are the initial guesses x0 given or we just assume? Also great video!

[rohullahakhlaghy]

Thanks
How can we know which one of the equations should we solve to get the answer?

[Tao-of-london]

I don’t understand this convergence criteria