filmov
tv
Convergent sequences are bounded

Показать описание
Convergent Sequences are Bounded
In this video, I show that if a sequence is convergent, then it must be bounded, that is some part of it doesn't go to infinity. This is an important result that is used over and over again in analysis. Enjoy!
Other examples of limits can be seen in the playlist below.
In this video, I show that if a sequence is convergent, then it must be bounded, that is some part of it doesn't go to infinity. This is an important result that is used over and over again in analysis. Enjoy!
Other examples of limits can be seen in the playlist below.
Proof: Convergent Sequence is Bounded | Real Analysis
Convergent sequences are bounded
Proof that every convergent sequence is bounded
Real Analysis Course #19 - Convergent Sequences are Bounded (Proof)
Real Analysis | A convergent sequence is bounded.
Every Convergent Sequence is Bounded
Convergent sequences are bounded
Convergent sequences are bounded
Example of Using the Monotonic Sequence Theorem | Calculus 2
Convergent sequences are bounded
Calculus - Lecture 4 - Convergent sequences are bounded
Every convergent sequence is bounded | prove that every convergent sequence is bounded|Real analysis
Math 101 100417 Convergent sequences are bounded
Proof: Convergent Sequences are Bounded
Proof that Convergent Sequences are Bounded
Advanced Calculus I, Part 6, Convergent Sequences are Bounded, Comparison Lemma, Subsequences
every convergent sequence is bounded
11.5 Every convergent sequence is bounded
Every Convergent Sequence is Bounded but converse is not true
Convergent Sequence is Bounded | L3 | TYBSc Maths | Completeness @ranjankhatu
Theorem on Convergent Sequence | Every Convergent Sequence is bounded | Real Analysis | NET JRF
Every convergent sequence is bounded | Proof | Real analysis | sequence and series | Real sequence
Sequences - A convergent sequence is bounded - proof
Sequences and Series: Sequences - 09. Convergent Sequences are Bounded
Комментарии
 0:05:47
0:05:47
 0:06:44
0:06:44
 0:03:28
0:03:28
 0:04:24
0:04:24
 0:11:51
0:11:51
 0:15:54
0:15:54
 0:12:53
0:12:53
 0:07:06
0:07:06
 0:08:21
0:08:21
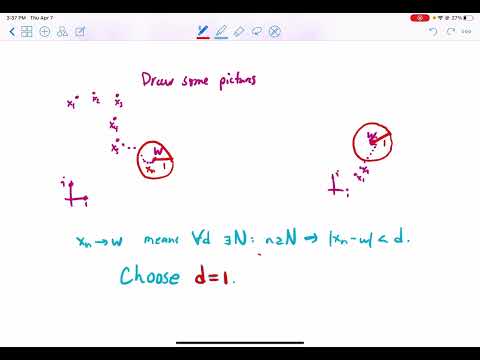 0:16:37
0:16:37
 0:07:50
0:07:50
 0:11:02
0:11:02
 0:08:30
0:08:30
 0:18:38
0:18:38
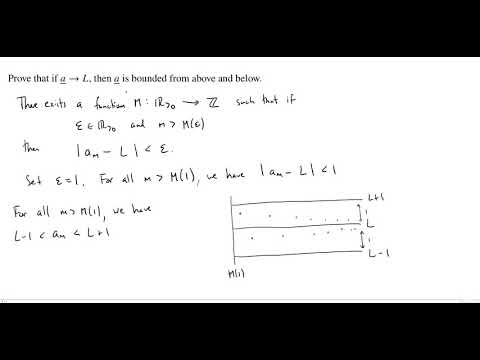 0:07:57
0:07:57
 0:20:46
0:20:46
 0:04:51
0:04:51
 0:08:18
0:08:18
 0:14:48
0:14:48
 0:08:31
0:08:31
 0:06:14
0:06:14
 0:09:21
0:09:21
 0:16:21
0:16:21
 0:05:04
0:05:04