Converting Riemann sums 👉to👉 definite integrals 🤓🤓 #apcalculus #apcalc #unit6 #shorts

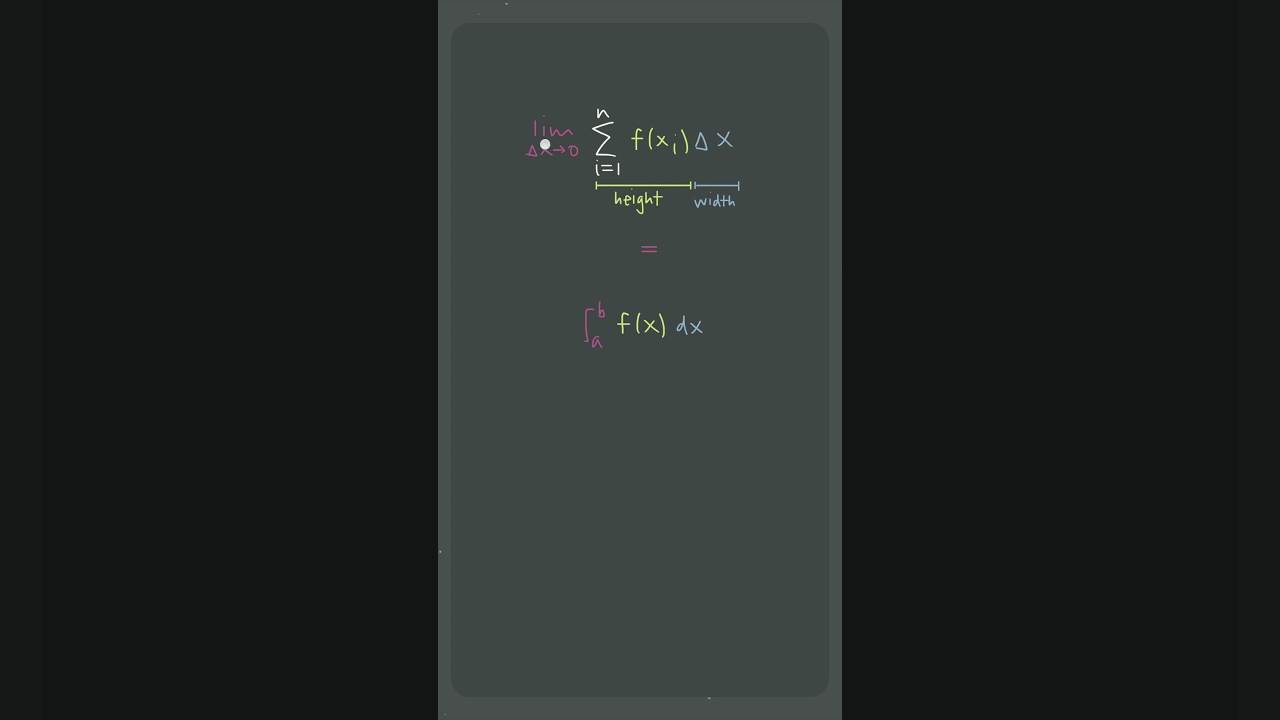
We focus on the relationship between Riemann sums and definite integrals. Riemann sums have us multiply the height of each approximating rectangle, f(x_i), by the width of the rectangle, Delta x, to get the area of each rectangle, and then sum those areas to get approximation of total area under the curve.

Transforming the Riemann sum into a definite integral involves taking the limit as Delta x approaches zero. Applying the limit to the Riemann sum makes the width of the approximating rectangles infinitely thin, which increases the number of rectangles from a finite number, n, to an infinite number. In other words, the integral gives exact area under the curve by using an infinite number of infinitely thin rectangles, in the same way that the Riemann sum gives approximate area under the curve using a finite number of rectangles with a specific width.

#APCalculus #RiemannSum #DefiniteIntegral #MathTutorial #CalculusConcepts #EducationalVideo #MathEducation #StudentResources #ExamPreparation #LearningMath

Unit 6 of AP Calculus is all about Integration and Accumulation of Change:
6.1 Exploring Accumulations of Change
6.2 Approximating Areas with Riemann Sums
6.3 Riemann Sums, Summation Notation, and Definite Integral Notation
6.4 The Fundamental Theorem of Calculus and Accumulation Functions
6.5 Interpreting the Behavior of Accumulation Functions Involving Area
6.6 Applying Properties of Definite Integrals
6.7 The Fundamental Theorem of Calculus and Definite Integrals
6.8 Finding Antiderivatives and Indefinite Integrals: Basic Rules and Notation
6.9 Integrating Using Substitution
6.10 Integrating Functions Using Long Division and Completing the Square
6.11 Integrating Using Integration by Parts (BC only)
6.12 Using Linear Partial Fractions (BC only)
6.13 Evaluating Improper Integrals (BC only)
6.14 Selecting Techniques for Antidifferentiation

_____

For extra help with your AP Calc (AB or BC), get my Ultimate Review Packet to help you review all year long and get prepared for the AP test:

[Thesaddestmomentinourlives]

Love that, but one small detail: we can say delta x approaches zero if there’s every single of segments is equal to the rest. Otherwise it’s the length of maximum segments approaches zero.