Find Laplace Transform using Convolution Property | 13 Practice Problems | Exam Review | Method 8

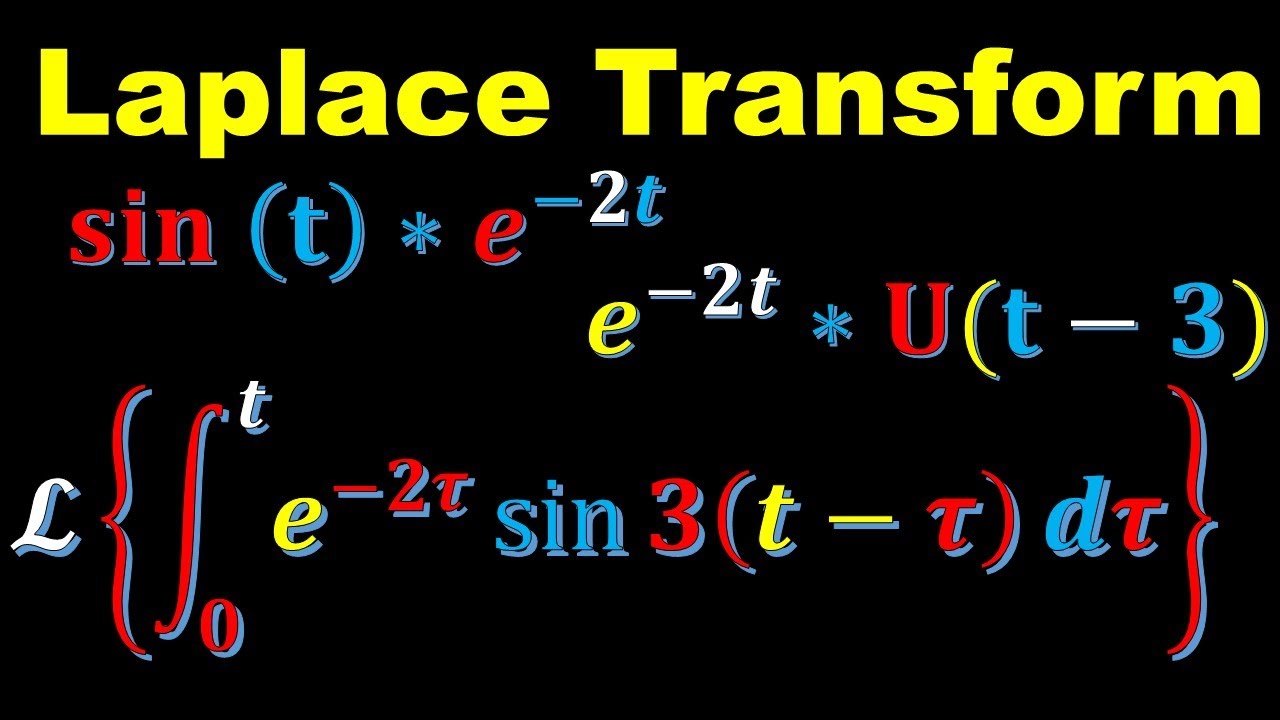
Показать описание

In this video we study the convolution method of finding Laplace transforms of certain functions.

Topics lineup
---------------------------------------------
00:00 Intro
02:34 Find convolutions
02:45 Pb a: 1* t
05:54 Pb b: t * t
07:25 Pb c: t * eᵗ
15:26 Pb d: eᵗ * sin t
21:53 Pb e: eᵐᵗ * eⁿᵗ, m ≠ n
24:59 Pb f: sin 3t * cos 2t
30:14 Convolution property (CP)
30:36 Pb g: ℒ{∫₀ᵗ τeᵗ⁻^τ dτ}
32:10 Pb h: ℒ{∫₀ᵗ (2τ −1)eᵗ⁻^τ dτ}
33:47 Pb i: ℒ{∫₀ᵗ eᵗ⁻^τ dτ}
35:07 Pb j: ℒ{∫₀ᵗ e²ᵗ⁻^τ dτ}
38:10 Pb k: ℒ{∫₀ᵗ τe⁻^τdτ}
41:25 Pb l: ℒ{∫₀ᵗ e⁻²^τsin 3(t−τ)dτ}
42:31 Pb m: ℒ{∫₀ᵗ sin τ sinh (t−τ)dτ}
43:25 Pb n: ℒ{∫₀ᵗ sin t sinh (t−τ)dτ}
44:20 Practice problems

Related videos
----------------------------------

Thank you for watching!

#differential_equations #exam_review #laplace_transforms

The Math Tutor

Рекомендации по теме

Комментарии

Math challenge: See whether you know the convolution of e⁻²ᵗ * U(t−3) and how to find the Laplace transform ℒ{∫₀ᵗ sin t sinh (t−τ)dτ}. If you know the answer please comment below. They may be a bit challenging.

MathTutor

Topics lineup

00:00 Intro
02:34 Find convolutions
02:45 Pb a: 1* t
05:54 Pb b: t * t
07:25 Pb c: t * eᵗ
15:26 Pb d: eᵗ * sin t
21:53 Pb e: eᵐᵗ * eⁿᵗ, m ≠ n
24:59 Pb f: sin 3t * cos 2t
30:14 Convolution property (CP)
30:36 Pb g: ℒ{∫₀ᵗ τeᵗ⁻^τ dτ}
32:10 Pb h: ℒ{∫₀ᵗ (2τ −1)eᵗ⁻^τ dτ}
33:47 Pb i: ℒ{∫₀ᵗ eᵗ⁻^τ dτ}
35:07 Pb j: ℒ{∫₀ᵗ e²ᵗ⁻^τ dτ}
38:10 Pb k: ℒ{∫₀ᵗ τe⁻^τdτ}
41:25 Pb l: ℒ{∫₀ᵗ e⁻²^τsin 3(t−τ)dτ}
42:31 Pb m: ℒ{∫₀ᵗ sin τ sinh (t−τ)dτ}
43:25 Pb n: ℒ{∫₀ᵗ sin t sinh (t−τ)dτ}
44:20 Practice problems

Thank you for watching.

MathTutor

Find Laplace Transform using Convolution Property | 13 Practice Problems | Exam Review | Method 8

The convolution and the laplace transform | Laplace transform | Khan Academy

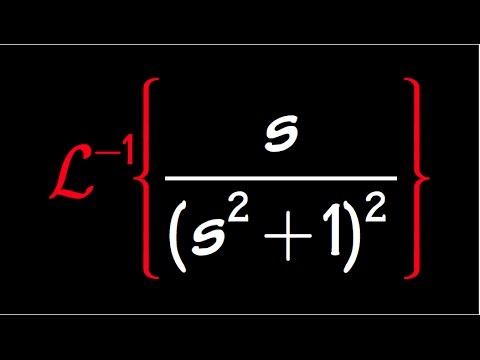
inverse laplace of s/(s^2+1)^2, using convolution theorem

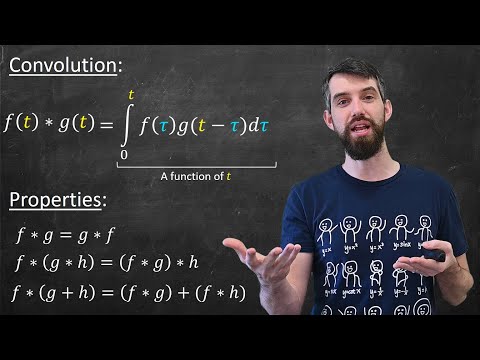
The Convolution of Two Functions | Definition & Properties

Diff Eqn: INVERSE Laplace Transform using convolution Integral

(6.3.102) Determine an Inverse Laplace Transform Using Convolution

Convolution theorem finding inverse Laplace transform example(PART-3) by easy maths easy tricks

Using the convolution theorem to solve an initial value prob | Laplace transform | Khan Academy

How to use the Convolution Theorem to Find the Laplace Transform (Easy Definite Integral Example)

How to use the Convolution Theorem to Find the Laplace Transform of a Convolution EX e^(2t)*sin(t)

Ex: Find the Laplace Transform of the Convolution Integral

How to Find the Laplace Transform of a Definite Integral using the Convolution Theorem

Convolution theorem finding inverse Laplace transform example(PART-1) BY easy maths easy tricks

Convolution Method to Find Inverse Laplace Transforms | Practice Problems

Electrical Engineering: Ch 16: Laplace Transform (52 of 58) What is Convolution? Circuit Ex.

Inverse Laplace Transform Using Convolution Theorem-Laplace Transform

Lesson 69: Using Convolution to Solve Inverse Laplace Transform (Engineering Math)

Convolution of Functions and Laplace Transforms Examples

7.4 Example - Finding Inverse Laplace Transform Using Convolution Theorem

Convolution theorem finding inverse Laplace transform example(PART-2) by easy maths easy tricks

Find inverse laplace transform using convolution theorem

How to Find the Laplace Transform of a Convolution Example with e(-t) and e^tcos(t)

The Convolution Theorem | State and prove Convolution Theorem | The Convolution operation |

Using convolution theorem obtain the inverse Laplace transform simple example

Inverse Laplace Transform Using Convolution Theorem-Laplace Transform