filmov
tv
Discrete Math - 9.3.1 Matrix Representations of Relations and Properties

Показать описание
How to represent a relation using a matrix and easy tips for determining if the relation is reflexive, irreflexive, symmetric, antisymetric, asymmetric and transitive. Matrix operations are also reviewed (meet, join and Boolean product).
Video Chapters:
Introduction 0:00
Matrix Representations 0:12
Matrix Representations in Reverse 3:09
Reflective Property 4:35
Irreflective Property 5:54
Symmetric Property 6:21
Anti-Symmetric Property 7:20
Asymmetric Property 8:34
Operations on Matrices 9:04
Transitive Property 15:55
Check for Understanding 17:00
Up Next 20:57
Textbook: Rosen, Discrete Mathematics and Its Applications, 7e
Video Chapters:
Introduction 0:00
Matrix Representations 0:12
Matrix Representations in Reverse 3:09
Reflective Property 4:35
Irreflective Property 5:54
Symmetric Property 6:21
Anti-Symmetric Property 7:20
Asymmetric Property 8:34
Operations on Matrices 9:04
Transitive Property 15:55
Check for Understanding 17:00
Up Next 20:57
Textbook: Rosen, Discrete Mathematics and Its Applications, 7e
Discrete Math - 9.3.1 Matrix Representations of Relations and Properties
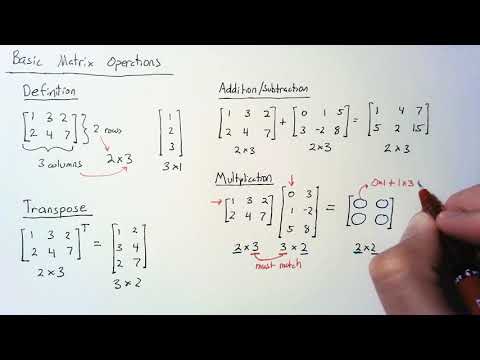
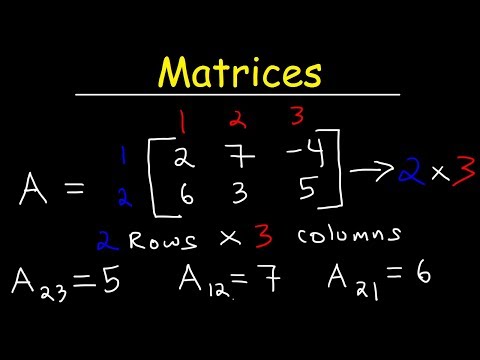
Discrete Math - 2.6.1 Matrices and Matrix Operations
Discrete Math - 2.6.3 Zero-One Matrices
Discrete Math 2.6.3 Zero-One Matrices
Composition of relations | MISTAKE - explained RoS instead of SoR and vice versa | otherwise correct
Linear Algebra - Matrix Operations
Intro to Matrices
Discrete Math 2.6.1 Intro to Matrices and Operations
Multiplying Matrices
How To Multiply Matrices - Quick & Easy!
6÷2(1+2)=???
Discrete Mathematics lecture 11 (A) Representing composite relations via matrices
Discrete Structures Sections 3.3 and 11.3 --- Matrices and Multiplication of matrices.
UPSC VS IIT JEE 🥵 #iitstatus #motivation #toppers #iitjee #jeemains #upscstatus #neet #nit #jee
Cramer's Rule - 3x3 Linear System
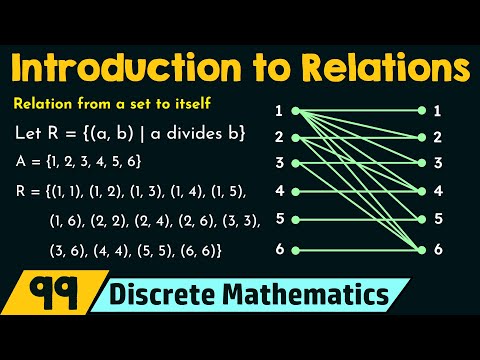
Types of Relations (Part 1)
Solving Matrix Equations
RELATIONS - DISCRETE MATHEMATICS
How to find Adjoint of 3 X 3 Matrix
Introduction to Relations
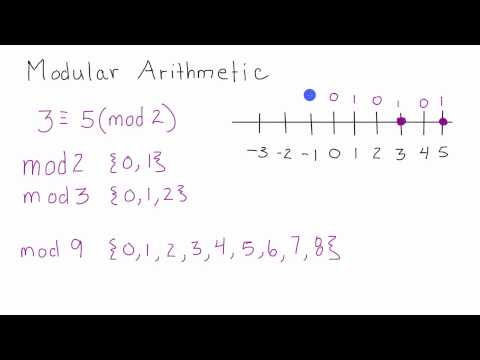
What does a ≡ b (mod n) mean? Basic Modular Arithmetic, Congruence
How much does a PHYSICS RESEARCHER make?
What is Modular Arithmetic - Introduction to Modular Arithmetic - Cryptography - Lesson 2
Simultaneous Equations Matrix Method : ExamSolutions
Комментарии
 0:21:04
0:21:04
 0:20:06
0:20:06
 0:08:34
0:08:34
 0:07:52
0:07:52
 0:03:20
0:03:20
 0:07:08
0:07:08
 0:11:23
0:11:23
 0:20:00
0:20:00
 0:17:40
0:17:40
 0:10:48
0:10:48
 0:01:21
0:01:21
 0:13:17
0:13:17
 1:06:00
1:06:00
 0:00:14
0:00:14
 0:15:03
0:15:03
 0:06:39
0:06:39
 0:06:31
0:06:31
 0:15:36
0:15:36
 0:09:35
0:09:35
 0:07:39
0:07:39
 0:05:45
0:05:45
 0:00:44
0:00:44
 0:04:48
0:04:48
 0:09:23
0:09:23