WildLinAlg16: Applications of row reduction II

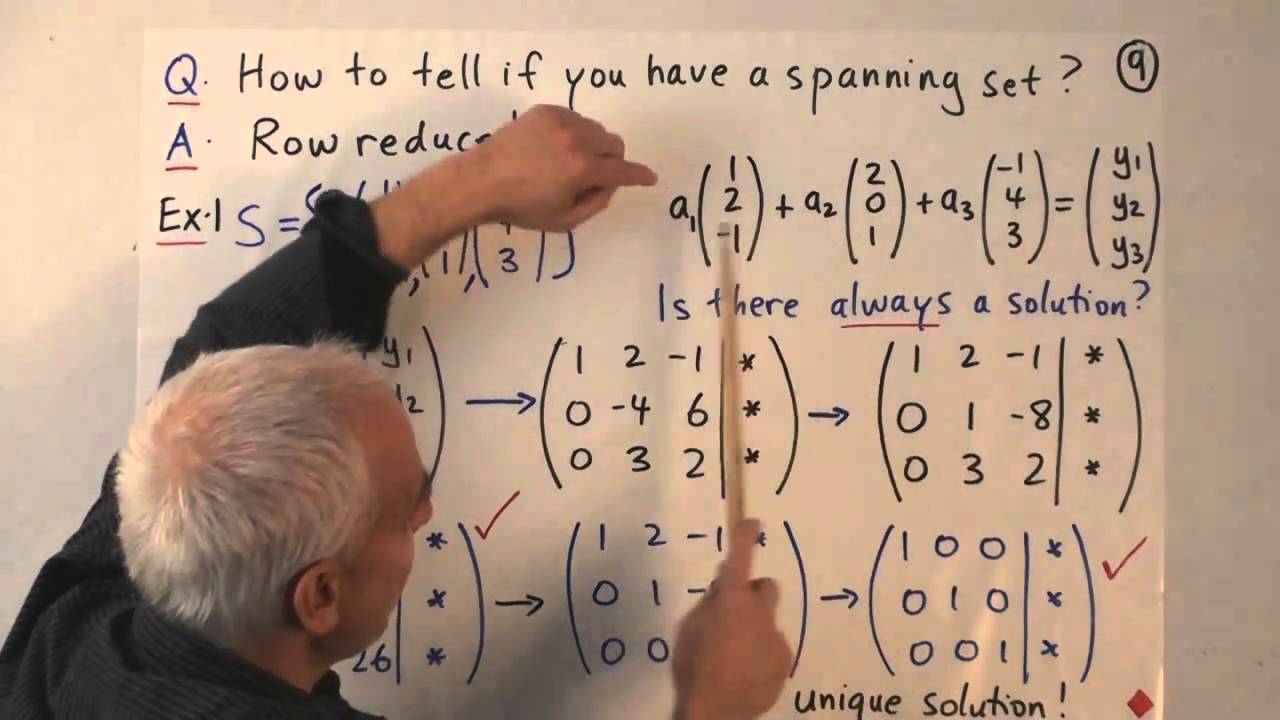
This video looks at various applications of row reduction to working with vectors and linear transformations in 2 and 3 dimensional space. We look at transformations given by 2x3 and by 3x2 matrices, along with the important notions of spanning sets and linearly independent sets of vectors.

This is part of a series on Linear Algebra by N J Wildberger of UNSW.

[lavc]

thank you teacher.going from 2 dim to 3 dim is the bottom line that nobody mentioned in the class.

[unswelearning]

Yes, for example the three vectors (1, 0), (0, 1) and (1, 1) are a spanning set of A^2, but are also linear dependent.

[WittyManQ]

This is good, thanks. I had to watch it in a hurry to meet a deadline... And I just have one question... Can something which is linearly dependent ever be a spanning set?

[jazzymarieke]

great serie, thanks! noticed a small mistake on slide 5: red vector v_2 must be above y_1 axis (-1, 1)

[zainkhn]

heeey, dooo you have the answers to the problems at the end, and also i dont quite fully understand the parameter part wen we back substitute and stuff

[lavc]

001 is not linear and nonlinear comb. is that true?

[unswelearning]

Hi 1966lavc

Not sure what you mean.