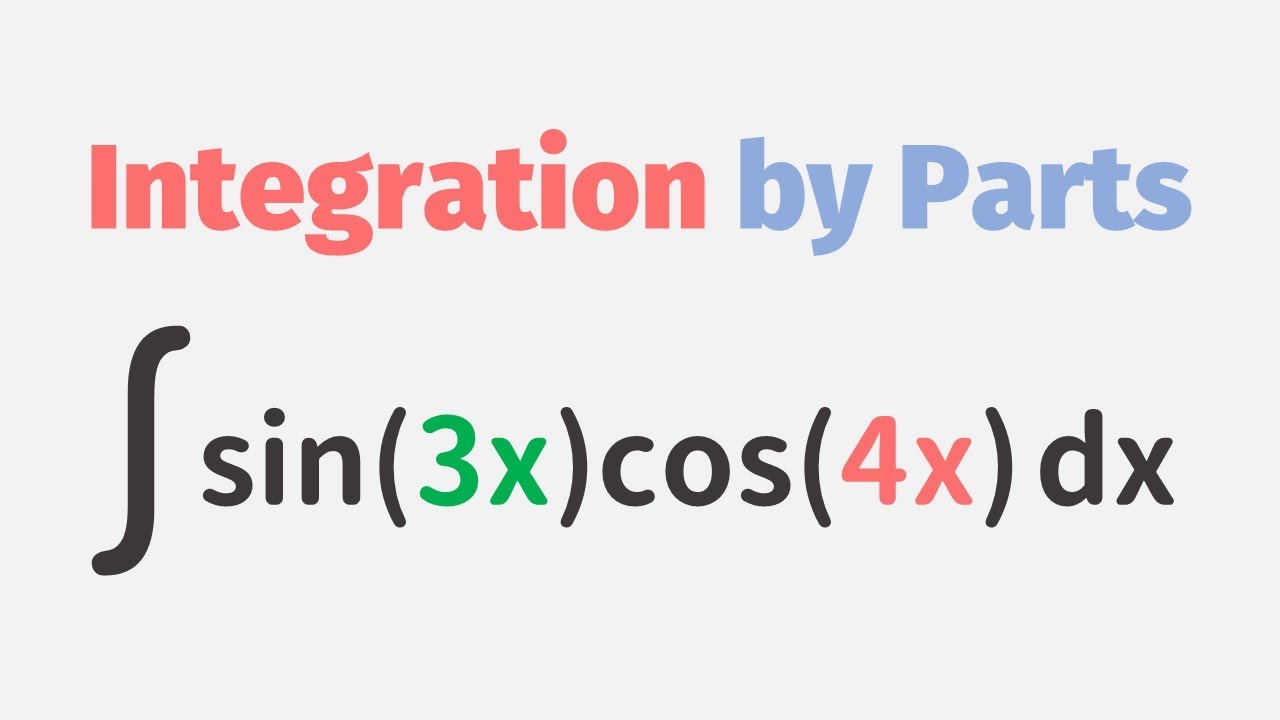
Integrate Sin(3x)Cos(4x) - No Trig Identities Needed! Going in a Loop!

In this video, we use the traditional method of integration by parts to find the antiderivative of a product of two different trigonometric function: sin(3x)cos(4x).

It is presented in textbooks that we are using the product-to-sum formula to turn this expression into a sum. But actually, we don't need to.

It does not matter whether we use u = sin(3x) or u = cos(4x), we are still going to get back to the original problem. So it looks like we are going in a loop!

See another video in which we go in a loop when integrating e^(-2x)sin(3x):

See how to integrate this without using integration by parts:

Please help me by subscribing to my YouTube channel. I want to use my free time to make a lot of math videos to help others and to make education available to the world. Your Likes and Subscribing will help this channel be reached by more people. Thank you!

Follow me:

[davidbrisbane]

sin(ɑ)cos(β) =½[sin(ɑ + β) + sin(ɑ - β)]

So, sin(3x)cos(4x)
= ½[sin(7x) + sin(-x)]
= ½[sin(7x) - sin(x)]

So, ∫sin(3x)cos(4x) dx
= ½∫[sin(7x) - sin(x)] dx
= - (1/14)*cos(7x) + (1/2)*cos(x)
= (1/2)*cos(x) - (1/14)*cos(7x)

[carultch]

Generalizing this method for this kind of integral gives interesting results. Consider integral sin(a*x)*cos(b*x) dx.

When frequencies of sine & cosine are different, going through this method, you get:
[b*sin(a*x)*sin(b*x) + a*cos(a*x)*cos(b*x)]/(b^2 - a^2) + C

When both functions have the same frequency, you'll get a divide by zero error in the above formula. However, you can still use a looper to integrate a sine & cosine product of the same frequency. Stop one row sooner, and your division by zero error goes away.

S ____ D I
+ __ sin(a*x) __ cos(a*x)
- ____ a*cos(x) __ 1/a*sin(x)

Spot the original integral, call it I, and solve for I:
I = 1/a*sin(a*x)^2 - I
2*I = 1/a*sin(a*x)^2
I = 1/(2*a)*sin(x)^2

Add +C and we have our result:
1/(2*a)*sin(x)^2 + C

[lovelyshimly]

Can I do the same order for my second one like my first one