filmov
tv
How to Solve a Buoyant Force Problem - Simple Example

Показать описание
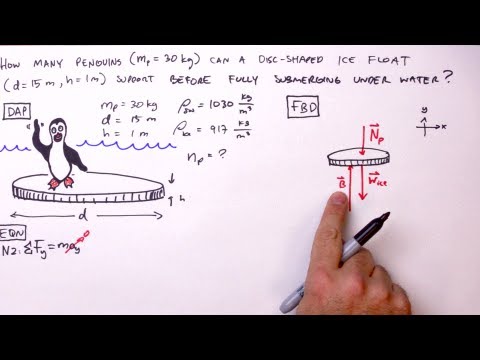
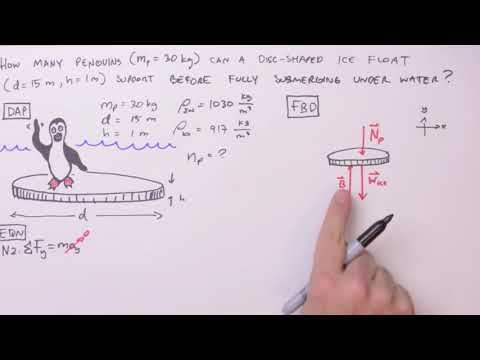
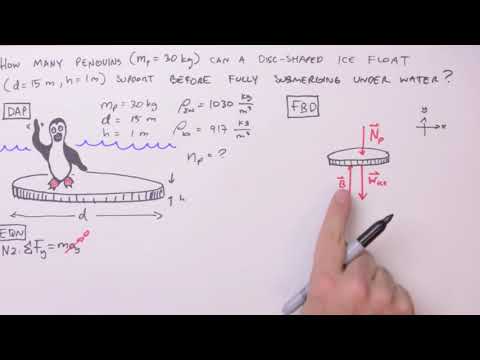
We use Archimedes' Principle to determine the number of penguins an ice float can dryly support.
Archimedes Principle, Buoyant Force, Basic Introduction - Buoyancy & Density - Fluid Statics
How to Solve a Buoyant Force Problem - Simple Example
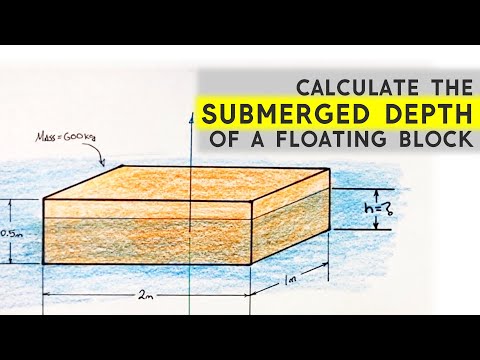
Worked Example | Calculate Submerged Depth of a Floating Block | Buoyancy
Fluids, Buoyancy, and Archimedes' Principle
How to Solve a Buoyant Force Problem Simple Example
How to Solve a Buoyant Force Problem Include Simple Example
Buoyant force example problems edited | Physical Processes | MCAT | Khan Academy
How To Calculate The Fractional Volume Submerged & The Density of an Object In Two Fluids
Buoyancy problem: find the mass of lead needed to sink a wood block (Archimedes' Principle)
Buoyancy and Buoyant Force Equation
Tricky Question | Buoyant Force #universe #brainteaser #science #puzzle #buoyancy #physics
How to Solve Buoyant Force (Buoyancy) Problems
Buoyant force example
Buoyancy & Floatation Metacentre
Problems on Buoyant Force
Buoyant Force Problems & Solution Tagalog
Buoyant Force Example Problem #1
Archimedes Principle: Top 3 Questions (Solved)
Buoyant Force Example Solution #2
How Archimedes Solved the Buoyant Force Puzzle 2000 Years Ago
Archimedes Principle Buoyant Force Wood and Lead in Water
BUOYANT FORCE (FLUID MECHANICS) - RANDOM PAST BOARD EXAM PROBLEM WITH SOLUTION
Buoyant Force on Floating Object
Upthrust | Buoyant force | Floatation #floatation #upthrust #physics #experiment #shortvideo #viral
Комментарии
 0:15:19
0:15:19
 0:07:12
0:07:12
 0:03:15
0:03:15
 0:04:16
0:04:16
 0:07:12
0:07:12
 0:07:12
0:07:12
 0:09:22
0:09:22
 0:14:15
0:14:15
 0:09:18
0:09:18
 0:04:04
0:04:04
 0:01:00
0:01:00
 0:04:35
0:04:35
 0:00:27
0:00:27
 0:03:36
0:03:36
 0:03:58
0:03:58
 0:31:22
0:31:22
 0:02:21
0:02:21
 0:06:31
0:06:31
 0:01:59
0:01:59
 0:08:14
0:08:14
 0:07:42
0:07:42
 0:04:22
0:04:22
 0:02:48
0:02:48
 0:00:09
0:00:09