Pillai Grad Lecture 10A 'Power Spectrum of Stationary Stochastic Processes' (1/2)

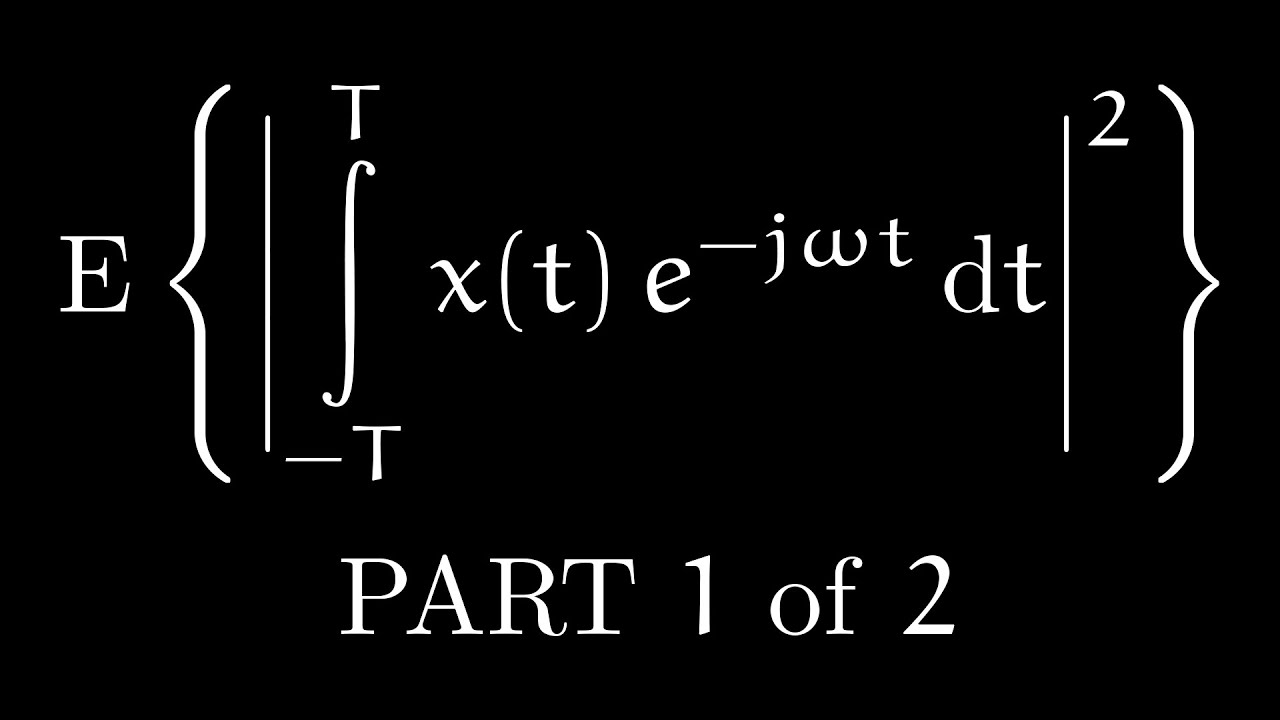
Показать описание

Classic Wiener-Khinchine theorem, where the power spectrum of a stationary stochastic process is shown to be the ordinary Fourier transform of its autocorrelation function. Derivation of the power spectrum of a stationary stochastic process as the Fourier transform of its autocorrelation function.

Probability, Stochastic Processes - Videos

Рекомендации по теме

Комментарии

unbelievable professor, he teaches from his mind....amazing indeed.
thanks for these super high quality videos.

ahmedgaafar

very beautifully presented....it clears a difficult topic ....end of my searching psd of wss

anupmallick

7:13 power is energy upon T. But in 22:20 the power expression E[X^2] does not have T. Why would this be sir? Possible answer: Power expression does indeed have T underneath. But T cancels out with the T from integrating dt. And E[X^2] does not depend upon T.
Update: I have found the answer. I had a misunderstanding. The "T" gets resolved during the integration and limit (he even mentions it, see 16:57 and 18:37). That is why the T is not there in 22:20. Not deleting the question as someone else might have the same doubt. Furthermore, at 22:20 the T is part of S_x(\omega).

niladridas

Prof. Thanks for this series. I was struggling through the book by myself, but it's much clearer now.

stephenmaharaj

Really great content. There is no much detailed and well explained material about Stochastic Process on internet. Thanks, this really helps.

manoelnt

@8:20, Why do we need to split the square of absolute into product of integral and its complement(*)? Why is it wrong to simply continue as product of 2 integrals?

AkashSingh-rvbr

Thank you verry much. I'm finishing my master degree and this lesson realy helped
me.

lucasrcrfull

Awesome.. A roller coaster ride on beautiful signal processing math

sanjaykrish

Thanks Sir YB but how do I recover the standard distribution function F(x) Out of te psd, and vice versa ? And even if this possible ?

samidelhi

Sir, is there any particular book that you follow?

niladridas

these videos r helping me a lot thank you for uploading
i think you should also do some problems

suryateja

Lol...at 4:30 someone wasn't paying attention!

Dominus_Ryder

Finger tap (no reaction)
Tap, tap, tap (reaction)
HELLO (i.e., yo, this is Graduate Lecture, shape up homeboi)

dacianbonta

Video is like taking acid before class.

alirezazamani

Pillai Grad Lecture 10A 'Power Spectrum of Stationary Stochastic Processes' (1/2)

Pillai Grad Lecture 10A 'Power Spectrum of Stationary Stochastic Processes' (1/2)

Pillai: Grad Lecture 10B 'Power Spectrum of Stationary Stochastic Processes' (2/2)

Pillai 'Power Spectrum of a Damped Sinc Function'

Pillai 'Whitening Filter'

PIllai 'Fourier Transforms'

Pillai 'Autocorrelation Function'

Pillai: Power Spectrum of Stationary Stochastic Processes

Pillai EL6333 Lecture 9 April 10, 2014 'Introduction to Stochastic Processes'

Pillai: ARMA Processes

Pillai Grad Lecture 8 'Basics of Stationary Stochastic Processes'

Pillai 'Variance and Covariance'

Pillai: Autocorrelations and Non-negative Definite Matrices

Power Spectral Density of a Signal Exercise

Pillai: Spectrum Extension Problem

Pillai: Grad Probability Lect. 1a: Conditional Probability and Independence

Power Spectrum

Pillai: Grad Probability: Bayes' Theorem and Inference

Pillai: M-ary Hypothesis Testing

Pillai 'Randomly Sampled Stochastic Processes Y(n) = X( ∑ ζ_i ) '

Pillai 'Matched Filter' (Version -2)

Pillai 'Stochastic Process applied to an LTI System'

Linear System Output Random Process Power

Pillai: Random Processes to Linear Systems

SPECTRAL DENSITY PROBLEM 4