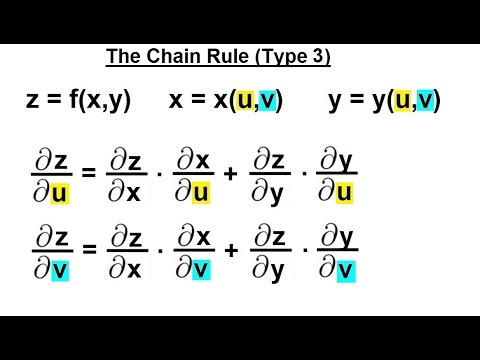
Calculus 3: Partial Derivative (28 of 50) The Chain Rule (Type 3)

Показать описание

In this video I will explain another method (3) of finding the partial derivative of z=f(x,y) where x=x(u,v) and y=y(u,v).

Next video in the series can be seen at:

Рекомендации по теме

Комментарии

Michel van Biezen, a very good introduction of the chain rule. You writing down the encourages me to make notes too. Thanks for uploading!

MechanicalEI

If you multiply out the formula and cancel out the partial dx and dy does it not simplify to 2* dz/du?
(d stands for the weird symbol for partial derivative)

hraklispapageorgiou

Dear sir, Can I assume then that the total change in z is the sum of the two partial derivatives dz/du + dz/dv? Any help would be greatly appreciated. I am trying to understand the formal derivation of La Grange in the calculus of variations whereby we are trying to differentiate F[x, y, y'], with respect to epsilon, and am confused as to which term to consider as a constant. Are we differentiating this F with respect to the terms within it implicitly? Thank you.

barryhughes

The rest of the videos from this point are not on the website, I would've missed them all :)

Tahycoon

Make pateron link alot of your viewers are willing to donate to you

yomomomama

Calculus 3: Partial Derivative (28 of 50) The Chain Rule (Type 3)

Calculus 3: Partial Derivative (28 of 50) The Chain Rule (Type 3)

Chain Rule With Partial Derivatives - Multivariable Calculus

Calculus 3 Lecture 13.3: Partial Derivatives (Derivatives of Multivariable Functions)

Calculus 3: Partial Derivatives (Video #13) | Math with Professor V

Multivariate Calculus: partial derivatives and differentials, integral curves, 9-28-23

Difference Between Partial and Total Derivative

Introduction to Partial Derivatives (Calculus 3)

Section 13.3 Partial Derivative Example

Calculus 3: Partial Derivative (29 of 50) The Chain Rule (Type 3): Example 1

Calculus 3: Partial Derivative (27 of 50) The Chain Rule (Type 2)

The Multi-Variable Chain Rule: Derivatives of Compositions

Section 13.3 Partial Derivatives from a Contour Map

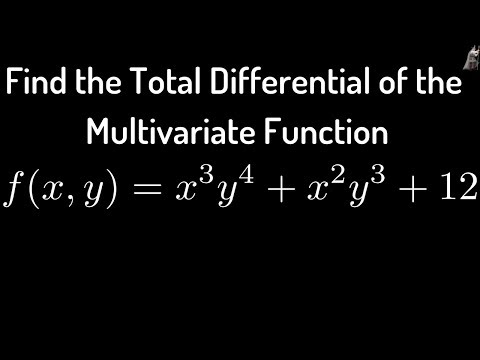
Total Differential of Multivariate Function f(x, y) = x^3y^4 + x^2y^3 + 12

What is differentiability for multivariable functions??

Multivariate Calculus Lecture 25 Partial Derivatives

Calculus 3 -- Partial derivatives -- More practice

Calc III: Find dy/dx using partial derivatives

This is How You Compute Partial Derivatives in Calculus

14.3 Partial derivative of a function of three variables (example 2)

Partial derivatives - How to solve?

Partial Derivative Examples Advanced (Including Derivative of an Integral)

Calculus 3: Partial Derivative (24 of 50) The Chain Rule

Multivariate Calculus: the three derivatives of vector calculus, 3-28-17, part 2

Video 3129 - Calculus 3, Implicit Partial Differentiation