Complex Numbers: Conjugate and Modulus | 22/27 | UPV

Título: Complex Numbers: Conjugate and Modulus

Descripción automática: In this video, the instructor introduces the concept of the conjugate and modulus of a complex number, prerequisites for which are a basic understanding of complex numbers and their operations. The video begins with a review of complex number addition, multiplication, and the calculation of inverses, employing formulas displayed on the screen.

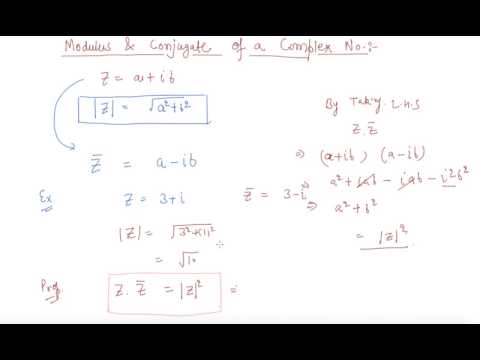
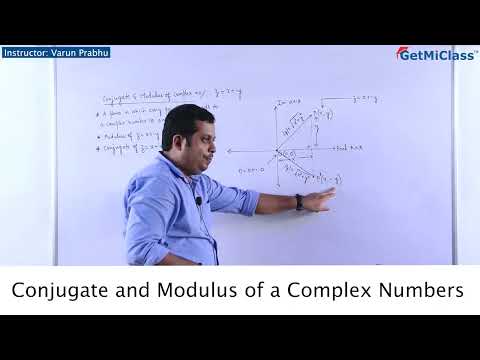
The conjugate of a complex number is explained as being the number with the opposite sign for its imaginary part but the same real part. This concept is illustrated with examples and the property that a complex number multiplied by its conjugate equals the sum of the squares of its real and imaginary parts is demonstrated.

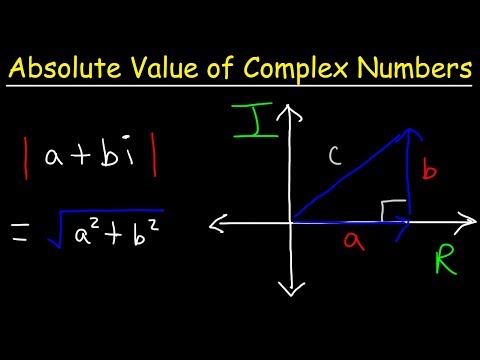
Furthermore, the modulus of a complex number is defined as the square root of the real part squared plus the imaginary part squared. Properties of the modulus are discussed, including its relation to the conjugate and how squaring the modulus yields the product of a complex number and its conjugate.

The video concludes by linking these concepts to the formula for the inverse of a complex number, showing that the inverse can be expressed as the conjugate over the square of the modulus. The session ends with a recap of the properties of conjugates and moduli in complex numbers.

Autor/a: Moll López Santiago Emmanuel

#Complex Number #Modulus #Conjugate #APPLIED MATHEMATICS