Nonhyperbolic Equilibria, Hamiltonian & Gradient Systems, Lyapunov Function, Ideal & Damped Pendulum

preview_player
Показать описание

Bill Kinney's Differential Equations and Linear Algebra Course, Lecture 33A.

(a.k.a. Differential Equations with Linear Algebra, Lecture 33A, a.k.a. Continuous and Discrete Dynamical Systems, Lecture 33A).

#hamiltoniansystems #lyapunovfunction #pendulum

(0:00) Lecture plans
(1:20) What can happen at non-hyperbolic equilibrium points?
(1:58) One-parameter family of nonlinear (cubic) ODEs where the polar coordinate “r” is relevant
(4:01) Use Mathematica to see what happens
(9:00) Transformed differential equation for dr/dt (differential equations in the polar coordinate r)
(12:45) Transformed differential equation for dθ/dt (differential equations in the polar coordinate θ)
(15:53) General Hamiltonian systems, Hamiltonian functions, and their properties
(18:16) General gradient systems, potential functions, and their properties
(21:20) Lyapunov function for a gradient system is the negative of the potential function
(22:36) What does it mean to be a Lyapunov function?
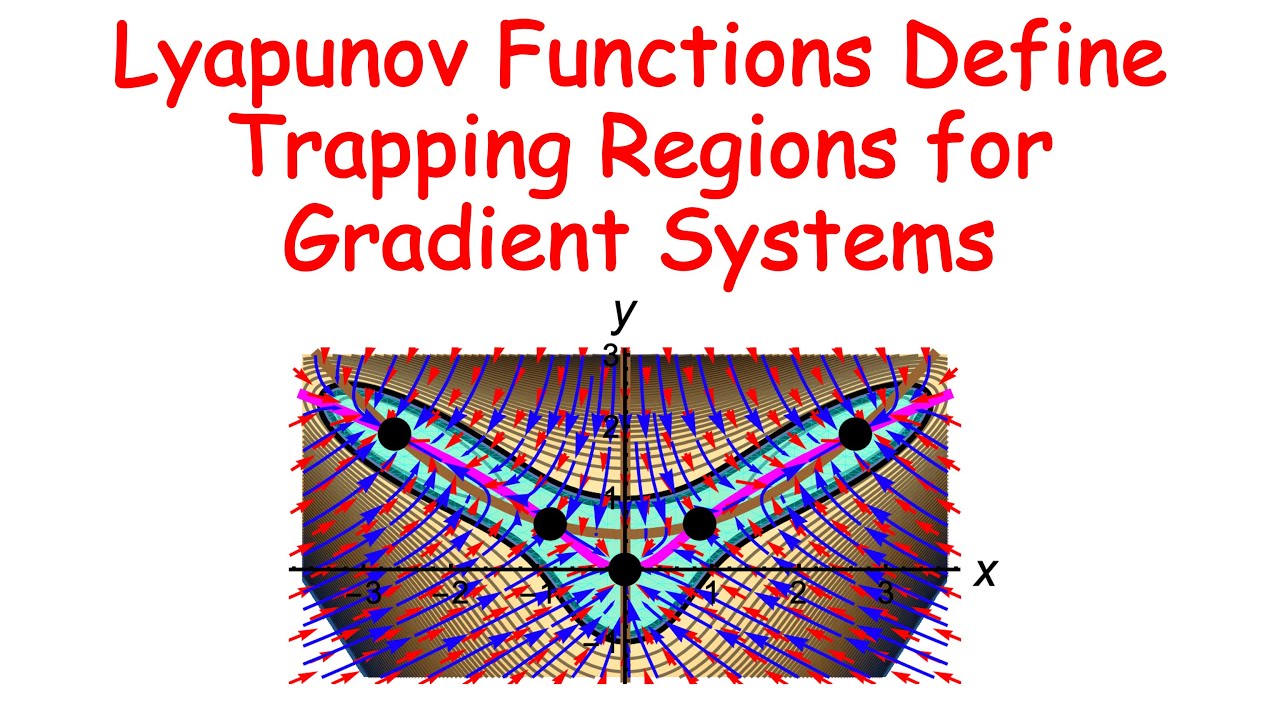
(25:23) Lyapunov functions and trapping regions for gradient systems (Lyapunov functions can be used to define trapping regions)
(28:34) Theorem about closed and bounded trapping region (there is at least one solution curve inside the trapping region for all time t)
(32:51) Ideal Pendulum (Undamped) Nonlinear and Unforced ODE
(36:37) This is a Hamiltonian system with Hamiltonian function representing total mechanical energy
(39:25) Physical interpretation of solutions (including separatrices)
(40:54) Damped Pendulum (include friction)
(46:33) Visualize the pendulum motion on Mathematica

AMAZON ASSOCIATE
As an Amazon Associate I earn from qualifying purchases.
  1. Bill Kinney
  2. nonhyperbolic equilibrium point
  3. nonhyperbolic equilibria
  4. nonhyperbolic equilibrium points
  5. nonhyperbolic fixed point
  6. hamiltonian systems
Рекомендации по теме
Nonhyperbolic Equilibria, Hamiltonian 0:48:26

Nonhyperbolic Equilibria, Hamiltonian & Gradient Systems, Lyapunov Function, Ideal & Damped ...

Diff Eqs Lec 0:51:43

Diff Eqs Lec #30, Hyperbolic Equilibria, Hamiltonian Systems, Pendulum

MATC58 Lec 2.4a: 0:34:59

MATC58 Lec 2.4a: nonhyperbolic equilibria and cobwebbing

Gradient Systems 1:12:27

Gradient Systems

Hamiltonian Systems - 0:12:22

Hamiltonian Systems - Nonlinear Systems with Special Structure, Part 2

Completely Integrable Hamiltonian 1:16:36

Completely Integrable Hamiltonian Systems | Poisson Bracket Series Expansions, Standard Map, Lect 14

Gradient Systems - 0:07:53

Gradient Systems - Nonlinear Differential Equations with Special Structure, Part 1

Hamiltonian Normal Forms, 1:00:54

Hamiltonian Normal Forms, Lie Series Method | Infinite-Dimensional Hamiltonians, Lecture 15

Non Euclidean Phase 0:32:30

Non Euclidean Phase Spaces (e.g. Invariant Spheres), Lyapunov's 2nd Method (Non Hyperbolic) Exa...

Lecture 4 (Part 0:30:31

Lecture 4 (Part 4): Lyapunov function & its properties; Gradient systems and gradient flows

Center Manifolds for 1:06:20

Center Manifolds for Hamiltonian Systems and PDEs | Lorenz System Bifurcation, Part 2 | Lecture 5

Change of Variables 0:05:53

Change of Variables 1 Dimension (u-substitution)

Hamiltonian PDEs, Shallow 1:13:47

Hamiltonian PDEs, Shallow Water Equations, Solitons, KdV, Parametric Resonance Intro, Lecture 16

DSA2021. Professor Angel 1:10:09

DSA2021. Professor Angel Jorba (UB) - Invariant Manifolds in Celestial Mechanics - Lecture 1

Integrable & Non-Integrable 1:14:22

Integrable & Non-Integrable Hamiltonian Systems, KAM Tori, Poincare Section, Poisson Bracket, Le...

Hamiltonian Systems 0:12:38

Hamiltonian Systems

M-07. The Hamilton’s 0:43:36

M-07. The Hamilton’s Formalism

MATH356 Lecture 8 1:08:57

MATH356 Lecture 8 Part 2

Hyperbolic vs Non-Hyperbolic 1:15:59

Hyperbolic vs Non-Hyperbolic Fixed Points- Computing Invariant Manifolds via Taylor Series Lecture 2

Canonical Transformations | 1:12:41

Canonical Transformations | Hamiltonian Changes of Variables | Symplectomorphisms | Lecture 5

Hamiltonian Flow is 1:01:47

Hamiltonian Flow is a Canonical Transformation | Strange Non-Intuitive Momenta | Lecture 8

Conservative Systems - 0:21:39

Conservative Systems - Nonlinear Systems with Special Structure, Part 3

Hyperbolic umbilic singularity 0:00:12

Hyperbolic umbilic singularity D4+

Limit Cycles, Part 0:14:27

Limit Cycles, Part 4: Van der Pol Equation, Strongly Nonlinear Limit, Relaxation Oscillations

Комментарии
Автор

Professor Kinney thank you for taking the time to summarize the Hamiltonian Systems, Gradient Systems and the Potential Functions and their contributions to Ordinary Differential Equations. The classical pendulum which is described mathematically as a Nonlinear/Linear Differential Equation is an excellent example of a Lyapunov Functions and Hamiltonian Systems for the damped case. These topics are not covered in Introductory Differential Equations.

georgesadler