filmov
tv
Integrable & Non-Integrable Hamiltonian Systems, KAM Tori, Poincare Section, Poisson Bracket, Lec 11

Показать описание
► Next: Poisson brackets, non-canonical Hamiltonian systems and Euler's rigid body equations
► Previous, Action-Angle Variables in Hamiltonian Systems | Visualizing Tori and Spheres in N Dimensions
► *Advanced Dynamics - Hamiltonian Systems and Nonlinear Dynamics*
► *Dr. Shane Ross, Virginia Tech professor (Caltech PhD)*
► *Follow me*
► Missed the first lecture, introducing Hamiltonian systems?
► A shorter, gentler introduction to Hamiltonian systems in 2D
► Course lecture notes (PDF)
Lecture 2020-03-26
► Course lecture notes (OneNote):
► Continuation of this course on a related topic
Center manifolds, normal forms, and bifurcations
► Chapters:
0:00 Introduction
0:30 Integrable and Non-Integrable Hamiltonian Systems
22:12 Non-Integrable Hamiltonian Systems
33:46 KAM Theorem and KAM tori
40:19 Poincare section, Poincare map
1:03:23 Poisson brackets and Poisson systems
Part of a graduate level course:
Advanced Dynamics (ESM/AOE 6314)
Spring Semester, 2020
► Courses and Playlists by Dr. Ross
📚Lagrangian and 3D Rigid Body Dynamics
📚Center Manifolds, Normal Forms, and Bifurcations
📚Attitude Dynamics and Control
📚Nonlinear Dynamics and Chaos
📚Hamiltonian Dynamics
📚3-Body Problem Orbital Dynamics
📚Space Manifolds
Charles Conley index theory gradient system autonomous on the plane phase plane are introduced 2D ordinary differential equations 2d ODE vector field topology cylinder bifurcation robustness fragility cusp unfolding perturbations structural stability emergence critical point critical slowing down supercritical bifurcation subcritical bifurcations buckling beam model change of stability nonlinear dynamics dynamical systems differential equations dimensions phase space Poincare Strogatz graphical method Fixed Point Equilibrium Equilibria Stability Stable Point Unstable Point Linear Stability Analysis Vector Field Two-Dimensional 2-dimensional Functions Hamiltonian Hamilton streamlines weather vortex dynamics point vortices pendulum Newton's Second Law Conservation of Energy topology
#Hamiltonian #NonlinearDynamics #DynamicalSystems #Poisson #Kolmogorov #Arnold #Moser #KAM #KAMtorus #Euler #DynamicalSystems #Poincare #PoincareMap #PoincareSection #Torus #geometry #dynamics #ChaoticDynamics #StandardMap #tsunami #transformations #mechanics #DynamicalSystems #NonlinearDynamics #Hamiltonian #dynamics #mechanics #physics #sphere #Nsphere #hypersphere #Tori #torus #topology #QuasiPeriodic #Oscillations #LimitCycles #VectorFields #topology #IndexTheory #EnergyConservation #Hamiltonian #Streamfunction #Streamlines #Vortex #SkewGradient #Gradient #PopulationBiology #FixedPoint #DifferentialEquations #Bifurcation #SaddleNode #Eigenvalues #HyperbolicPoints #NonHyperbolicPoint #CuspBifurcation #CriticalPoint #buckling #PitchforkBifurcation #robust #StructuralStability #DifferentialEquations #dynamics #dimensions #PhaseSpace #PhasePortrait #PhasePlane #Poincare #Strogatz #Wiggins #VectorField #GraphicalMethod #FixedPoints #EquilibriumPoints #Stability #NonlinearODEs #StablePoint #UnstablePoint #Stability #LinearStability #LinearStabilityAnalysis #StabilityAnalysis #VectorField #TwoDimensional #Functions #PopulationGrowth #DynamicalSystems #PopulationDynamics #Population #Logistic #GradientSystem #GradientVectorField #Cylinder #Pendulum #Newton #LawOfMotion #dynamics
Комментарии
 1:14:22
1:14:22
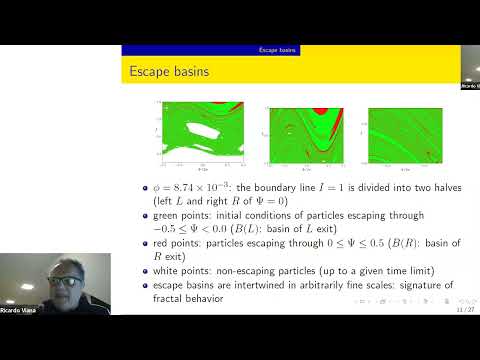 0:31:18
0:31:18
 0:46:54
0:46:54
 1:23:14
1:23:14
 0:31:23
0:31:23
 0:33:20
0:33:20
 0:55:47
0:55:47
 1:16:36
1:16:36
 0:54:13
0:54:13
 0:09:30
0:09:30
 0:15:19
0:15:19
 1:29:39
1:29:39
 0:00:38
0:00:38
 1:43:11
1:43:11
 1:13:30
1:13:30
 0:37:20
0:37:20
 0:59:53
0:59:53
 1:10:21
1:10:21
 1:22:29
1:22:29
 1:33:05
1:33:05
 0:00:35
0:00:35
 3:14:49
3:14:49
 0:46:17
0:46:17
 1:04:26
1:04:26