filmov
tv
(Example) Calculus II Work: Spring Problem
Показать описание
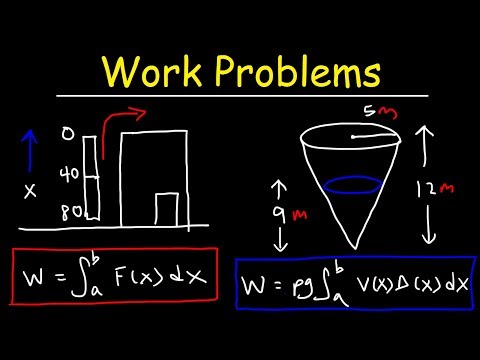
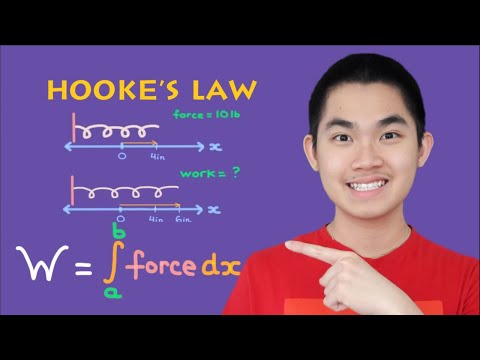
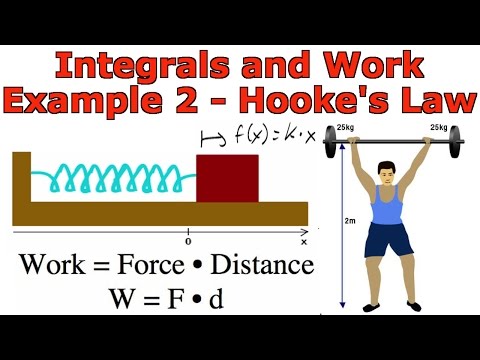
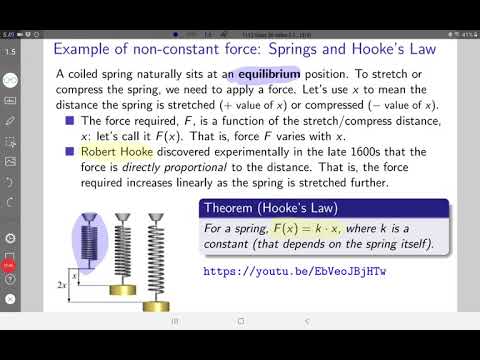
A spring has a natural length of 10 cm. If a 22 N force is required to keep it stretched to a length of 20 cm, how much work is required to stretch it from 14 cm to 19 cm?
0:07:52
0:07:42
0:03:59
0:14:27
0:32:06
0:06:58
0:57:10
0:01:53
0:26:19
0:04:14
0:03:30
0:03:18
0:02:37
0:06:32
0:14:57
0:57:28
0:26:33
0:27:27
0:16:27
0:04:12
0:09:16
0:15:13
0:22:56
0:34:30