IMO SL 2010 A6 - One of my favorite functional equations of all time

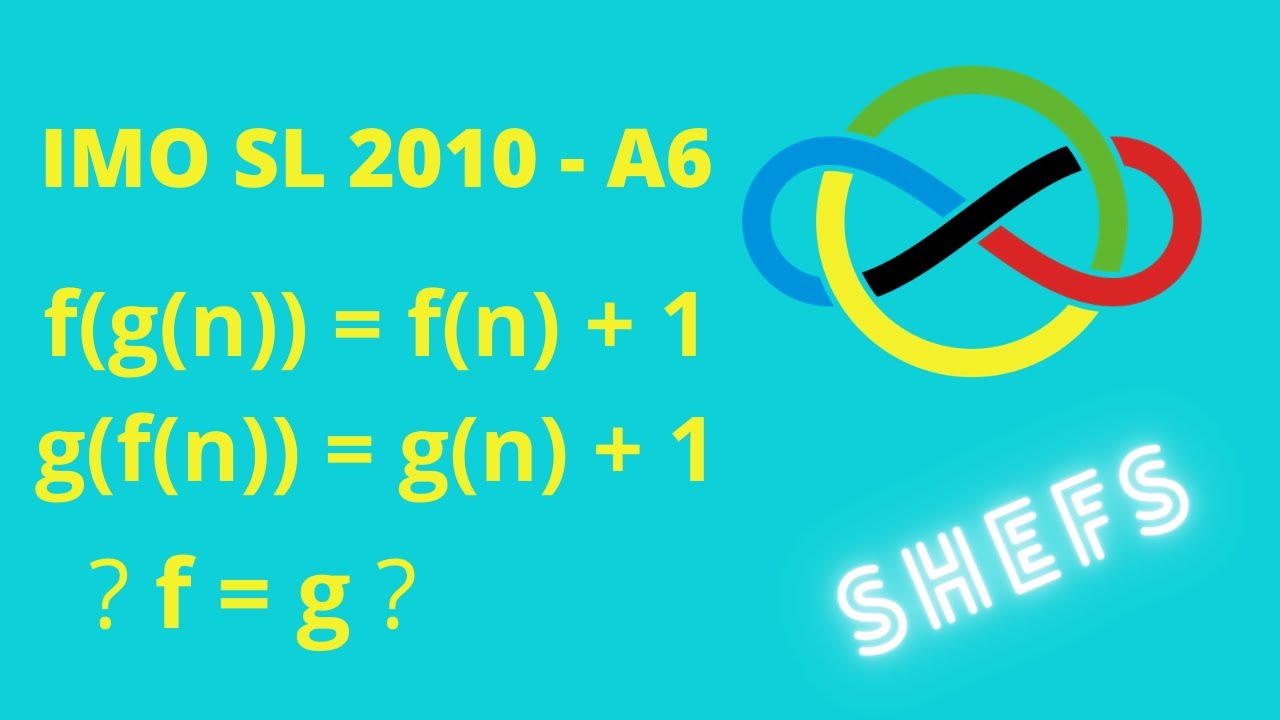
Показать описание

Hello fellow problem solver!

This is a problem from the 2010 International Math Olympiad Shortlist, the 6th Algebra problem from the list (out of 8 algebras), or as it's otherwise known A6. The problem rated more difficult than it, A7, was the 6th problem at the IMO (by some estimates, namely the mean of points, the hardest one at that IMO).

I absolutely love this problem! For me, the beauty is in the fact that it's not a regular functional equation problem and that it's about asking yourself some "curiosity inspired" questions to lead your focus. There's a lot of different facts you will discover about the problem. The goal is to figure out which facts to "push further" and how far can you "push" them.

So for this problem you need to understand:
- what functions are
- how to solve functional equations in the positive integers

Experience helps a lot here, especially with the positive integers (which is the domain and codomain of the function). That being said, this is not a pure "textbook" function. Rather, it involves a lot of thinking and creativity (not an A6 for no good reason).

Here are the timestamps:
00:00 - 00:40 Problem statement
00:40 - 01:00 Expressions of love
01:00 - 01:50 Problem solving philosophy
01:50 - 02:10 First idea
02:10 - 05:40 Exploring the first idea
05:40 - 06:20 Second idea development
06:20 - 08:00 Working on the second idea
08:00 - 09:10 Looking for more ideas
09:10 - 10:40 A new focus
10:40 - 12:20 Third idea
12:20 - 12:30 Loving the problem again
12:30 - 14:30 Developing a new idea
14:30 - 16:00 Fourth idea
16:00 - 18:45 Fifth idea
18:45 - 20:40 The finish
20:40 - 26:57 Summary of the solution

And as always,
Thanks for problem solving :)