Algebra 1 Practice - Solve and Graph a Multi-Step Inequality (Example 4)

### Algebra 1 Practice: Solve and Graph a Multi-Step Inequality

Solving and graphing a multi-step inequality in Algebra 1 involves applying multiple operations, including both addition/subtraction and multiplication/division, to isolate the variable and then representing the solution set visually on a number line. Here's a guide to help you tackle this:

---

#### **Steps to Solve a Multi-Step Inequality**

1. **Identify the Inequality:**
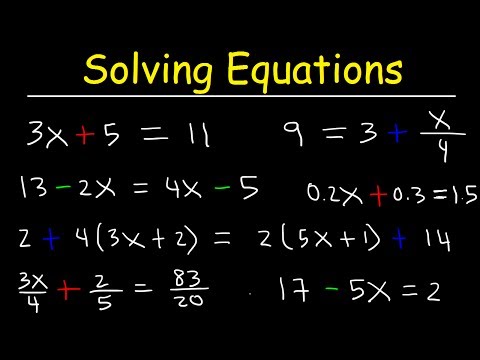
- Recognize the inequality form, which often includes multiple operations, such as \( ax + b \) is less than or equal to \( c \) or \( dx - e \) is greater than \( f \).

2. **Isolate the Variable:**
- Use a combination of addition/subtraction and multiplication/division to isolate the variable.
- **Undo Addition/Subtraction:** If the variable is added to or subtracted by a number, perform the opposite operation on both sides.
- **Undo Multiplication/Division:** If the variable is multiplied or divided by a number, perform the opposite operation on both sides. Remember to reverse the inequality sign if you multiply or divide by a negative number.

3. **Solve the Inequality:**
- After isolating the variable, simplify to find the inequality solution.

4. **Example:**
- Solve \( 2(x - 3) + 5 \) is greater than \( 7x - 3 \):
1. Distribute and simplify: \( 2x - 6 + 5 \) is greater than \( 7x - 3 \)
2. Combine like terms: \( 2x - 1 \) is greater than \( 7x - 3 \)
3. Subtract \( 2x \) from both sides: \( -1 \) is greater than \( 5x - 3 \)
4. Add 3 to both sides: \( 2 \) is greater than \( 5x \)
5. Divide both sides by 5: \( \frac{2}{5} \) is greater than \( x \)

---

#### **Steps to Graph the Inequality on a Number Line**

1. **Draw a Number Line:**
- Create a number line that includes the critical values found in the inequality.

2. **Mark the Critical Values:**
- Identify the critical values on the number line.

3. **Determine the Type of Circle:**
- Use an **open circle** (○) or a **closed circle** (●) based on whether the boundary value is included in the solution or not.

4. **Shade the Region:**
- Shade the region on the number line that satisfies the inequality.

5. **Example:**
- For \( x \) is less than \( \frac{2}{5} \):
- Draw a number line.
- Mark \( \frac{2}{5} \) with an open circle (○) because \( x \) is less than \( \frac{2}{5} \).
- Shade to the left of \( \frac{2}{5} \).

---

#### **Complete Example: Solving and Graphing**

- **Inequality:** \( 2(x - 3) + 5 \) is greater than \( 7x - 3 \)
1. **Solve:**
- Distribute and simplify: \( 2x - 6 + 5 \) is greater than \( 7x - 3 \)
- Combine like terms: \( 2x - 1 \) is greater than \( 7x - 3 \)
- Subtract \( 2x \) from both sides: \( -1 \) is greater than \( 5x - 3 \)
- Add 3 to both sides: \( 2 \) is greater than \( 5x \)
- Divide both sides by 5: \( \frac{2}{5} \) is greater than \( x \)
2. **Graph:**
- Draw a number line.
- Mark \( \frac{2}{5} \) with an open circle (○) because \( x \) is less than \( \frac{2}{5} \).
- Shade to the left of \( \frac{2}{5} \).

---

By following these steps, you can effectively solve and graph multi-step inequalities, visually representing the solution set on a number line. This process helps in understanding and interpreting the solutions for various inequality problems in Algebra 1. Regular practice with different types of inequalities will help reinforce these skills.

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#math #algebra #algebra2 #maths