filmov
tv
Algebraic geometry 38: The Zariski tangent space (replacement)

Показать описание
This lecture is part of an online algebraic geometry course, based on chapter I of "Algebraic geometry" by Hartshorne. It covers the Zariski tangent space, and describes some other ways of viewing tangent spaces.
(This is a replacement for the original video, which had poor audio quality.)
(This is a replacement for the original video, which had poor audio quality.)
Algebraic geometry 38: The Zariski tangent space (replacement)
Algebraic Geometry: Spec A compact in Zariski Topology | THESUBNASH - Jeden Tag ein neues Mathevideo
Fermat's Last Theorem: The Zariski Tangent Space, Functorially! (129, 8.10)
3.1 The Zariski topology (Commutative Algebra and Algebraic Geometry)
Tutorial 10 : Zariski Tangent Space at a Point of an Affine Variety
Closed Subsets of A1 in the Zariski Topology Algebraic Geometry Mathematics Mathchannel
12.1 The tangent space - concretely (Commutative Algebra and Algebraic Geometry)
9.2 Projective Zariski topology and Nullstellensatz (Commutative Algebra and Algebraic Geometry)
Zariski Tangent Space
Zariski Tangent Space, Singular & Nonsingular Points
Christian Dahlhausen - Continuous K-theory and K-theory of Zariski-Riemann spaces
What is Zariski topology?
The Zariski Topology and Affine Space #swayamprabha #CH38SP
Irreducibility in the Zariski Topology #swayamprabha #CH38SP
Alexander Kuznetsov: Homological algebraic geometry
Analyzing Open Sets and Basic Open Sets for the Zariski Topology #swayamprabha #CH38SP
Understanding the Zariski Topology on the Affine Line; The Noetherian prop.... #swayamprabha #CH38SP
Commutative algebra 32 Zariski's lemma
The Ring of Functions on a Basic Open Set in the Zariski Topology #swayamprabha #CH38SP
Yohann Genzmer : The Zariski problem for homogeneous and quasi-homogeneous curves
Zariski tangent space | Wikipedia audio article
Affine algebraic geometry: Algebraic Incarnation of Points
8.2 Algebraic varieties (Commutative Algebra and Algebraic Geometry)
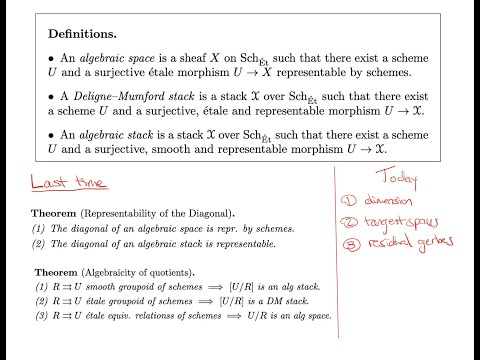
Lecture 8: Dimension, tangent spaces, and residual gerbes
Комментарии
 0:22:25
0:22:25
 0:09:02
0:09:02
 0:07:37
0:07:37
 0:10:58
0:10:58
 0:33:05
0:33:05
 0:01:59
0:01:59
 0:14:59
0:14:59
 0:23:17
0:23:17
 0:35:59
0:35:59
 0:29:06
0:29:06
 1:13:58
1:13:58
 0:07:15
0:07:15
 0:51:57
0:51:57
 0:53:39
0:53:39
 1:00:12
1:00:12
 0:45:35
0:45:35
 0:57:51
0:57:51
 0:23:19
0:23:19
 0:47:32
0:47:32
 1:06:56
1:06:56
 0:08:26
0:08:26
 0:11:38
0:11:38
 0:21:20
0:21:20
 1:14:16
1:14:16