Problem 13-99 | Force & Acceleration | Chapter: 13 | Kinetics of a Particle

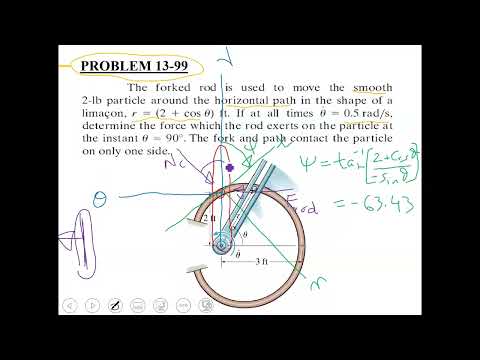
Problem 13-99 : The forked rod is used to move the smooth 2-lb particle around the horizontal path in the shape of a limacon, r= ( 2 + cos theta) ft. if at all times theta dot = 0.5 rad/sec, determine the force which the rod exerts on the particle at the instant theta = 90 degrees the fork and the path contact the particle on only one side.

Welcome to Chapter 13 of "Engineering Mechanics Dynamics," where we dive deep into the dynamic world of particle kinetics, focusing specifically on force and acceleration. In this intriguing topic, we're about to tackle "Problem 13-99," an engaging scenario that involves a forked rod and a smooth 2-lb particle navigating a unique horizontal path shaped like a limaçon.

📚 Book Name: Engineering Mechanics Dynamics
📝 Chapter: 13 | Kinetics of a Particle

⚙️ Topic: Force & Acceleration Problem 13-99

In this dynamic problem, we encounter a fascinating situation where a forked rod is employed to guide a smooth 2-lb particle along an unconventional path—a limaçon, described by the equation r = 2 + cos(θ) ft. What makes this problem captivating is the intricacy of the particle's motion, guided by the angular velocity θ dot = 0.5 rad/sec at all times.

Our objective is clear: We aim to unveil the force exerted by the forked rod on the particle at a pivotal instant—when θ equals 90 degrees. This is no ordinary scenario, as the fork and the path make contact with the particle on just one side, introducing an intriguing asymmetry that warrants thorough analysis.

Through the lens of dynamics, we're equipped to harness principles of kinematics and force analysis. By dissecting the particle's motion and unraveling the interactions between the forked rod and the particle, we'll derive insights into the intricate forces at play. We'll navigate the nuances of acceleration, angular velocity, and contact forces to decipher the dynamic equilibrium governing this situation.

As we embark on this journey, we'll engage with the dynamic world of mechanics, where math and physics converge to provide a holistic understanding of motion and forces. By dissecting this complex problem, we'll gain a deeper appreciation for the mechanics that underpin the motion of particles along unconventional paths.

Tags:
#ParticleKinetics, #ForceAndAcceleration, #EngineeringMechanicsDynamics, #DynamicScenario, #ForkedRodMotion, #LimaçonPath, #AngularVelocityAnalysis, #ContactForces, #DynamicEquilibrium, #KinematicsAndForce, #MechanicalInteractions, #ParticleMotion, #AngularVelocityConcepts, #ForceInfluence, #PhysicsAndMathematics, #ParticleInteractions, #KinematicPrinciples, #DynamicPhysics, #ParticleDynamics, #AngularVelocityInfluence, #EquilibriumInMotion, #ForkedRodPhysics, #DynamicPhysicsLaws, #ParticleEquilibrium, #PhysicsLaws, #EngineeringInsights, #ParticleContactForces, #DynamicEquations, #KinematicPhysics, #MechanicsExploration, #ParticleForces, #DynamicApplications, #EquationsAndForces, #DynamicPrinciples, #PhysicsAndEngineering, #DynamicEquilibriumAnalysis, #ForkedRodEquations, #MechanicsConcepts, #AngularVelocityMath, #PhysicsInMotion, #ParticlePaths, #DynamicChallenges, #PhysicsAndMotion, #ParticlePhysics, #DynamicMath, #ParticleEquations, #PhysicsPrinciples, #AngularVelocityPhysics, #EngineeringExamples, #DynamicCalculations, #ParticleMotionAnalysis, #AngularVelocityEquations, #DynamicAnalysis, #ParticleKinematics, #DynamicMathematics, #AngularVelocityDynamics, #ParticleEquilibriumAnalysis, #EngineeringStudies, #DynamicInterplay, #RealWorldDynamics

[quirkyaxis]

thx big slatt helped me out in dynamics alot