Synthetic Division of Polynomials | The Easy Way

Показать описание

Today, I'm going to show you the second way to divide polynomials using synthetic division. Now, in the last video, we talked about long division, which is the first method of dividing polynomials. So, the difference is that synthetic division works faster, but it only works when the divisor is first-degree (linear), meaning that x is to the power of 1. If the divisor has x to the power of 2 or 3, the synthetic division will not work!

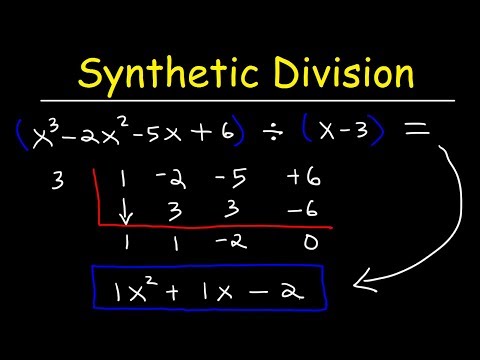
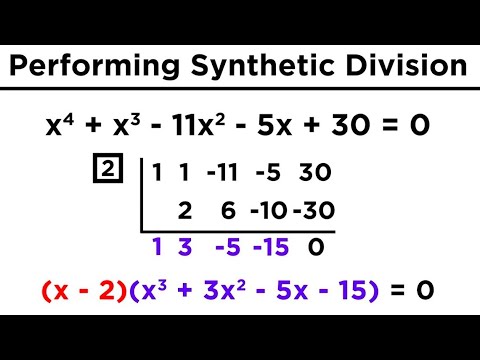
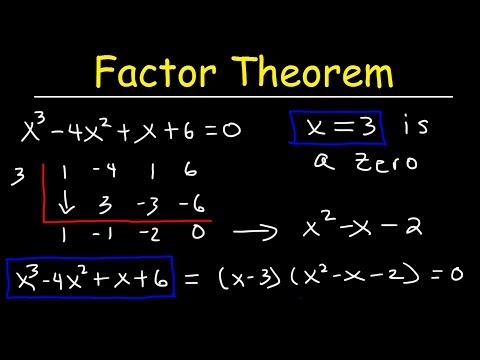
The first step is to drop down the coefficients of the dividend (or the polynomial that is being divided). You must follow the sequence of x, meaning that if the first term is x^4, then make sure you continue that sequence to x^3, x^2, x, etc. For example, you have x^3 + 5. After you follow the sequence, you will have x^3 + 0x^2 + 0x + 5. Notice the coefficients that you drop down are 1, 0, 0, 5 respectively.

The second step is to put the divisor (the polynomial that's is used to divide) on the left side of the division sign. To do that, set the divisor equal to zero. Then, you solve for x. After you get x, put that number on the left sign of the division sign.

We're almost done. The idea is you drop down the first term, multiply it by the "divisor" and add it to the second term. Watch the video to see what I mean. You repeat the process until you get the remainder. I want you to circle the remainder so you can add it later to your quotient.

Thank you so much for watching my videos. I hope you learnt something new!

LIKE & SUBSCRIBE:

MY EQUIPMENT:
Camera: Samsung Galaxy J7