filmov
tv
dimension of subspace W consisting of diagonal element Basis bhu 2020 linear algebra Mathematics
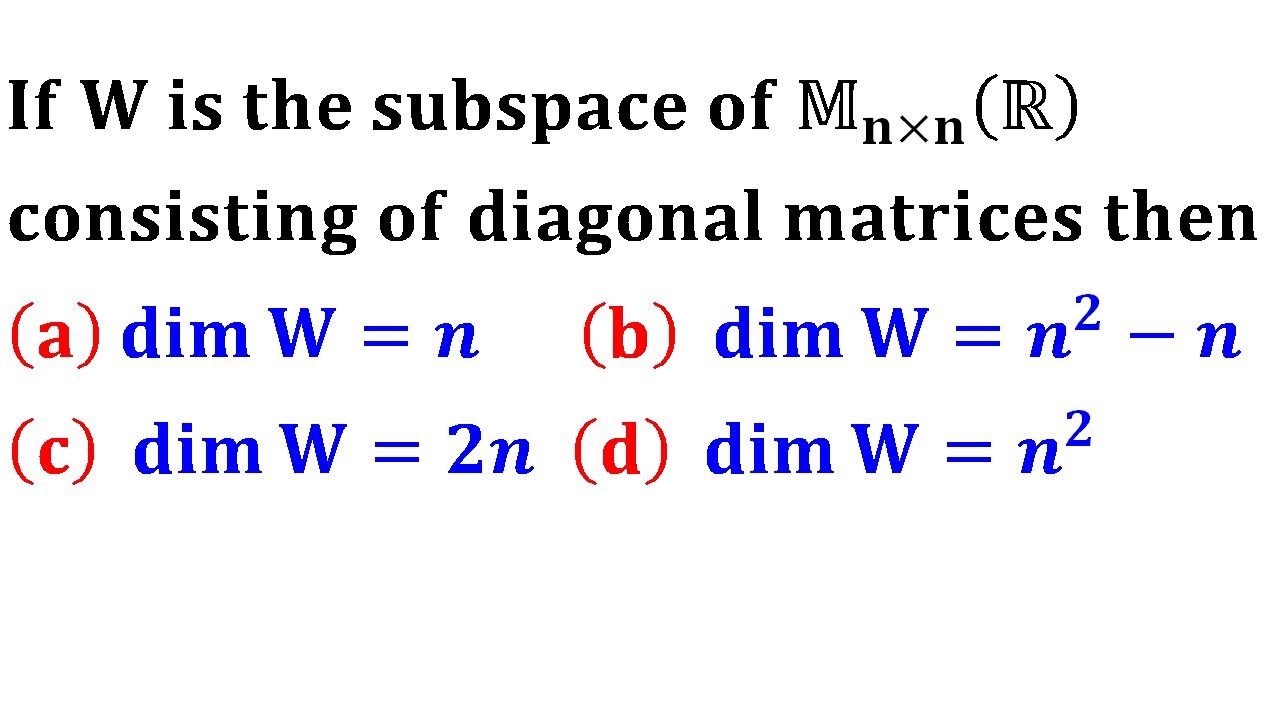
Показать описание
For Notes and Practice set WhatsApp @ 8130648819 or visit our Website
Join this channel to get access to perks:
Linear Algebra: Finite dimensional vector spaces, linear independence of vectors, basis, dimension, linear transformations, matrix representation, range space, null space, rank-nullity theorem. Rank and inverse of a matrix, determinant, solutions of systems of linear equations, consistency conditions, eigenvalues, and eigenvectors for matrices, Cayley-Hamilton theorem.
14. If W is the subspace of M_(n×n) (R) consisting of diagonal matrices then
(a) dimW=n (b) dimW=n^2-n (c) dimW=2n (d) dimW=n^2
En BHU PET 2020, 14
Key (a)
Join this channel to get access to perks:
Linear Algebra: Finite dimensional vector spaces, linear independence of vectors, basis, dimension, linear transformations, matrix representation, range space, null space, rank-nullity theorem. Rank and inverse of a matrix, determinant, solutions of systems of linear equations, consistency conditions, eigenvalues, and eigenvectors for matrices, Cayley-Hamilton theorem.
14. If W is the subspace of M_(n×n) (R) consisting of diagonal matrices then
(a) dimW=n (b) dimW=n^2-n (c) dimW=2n (d) dimW=n^2
En BHU PET 2020, 14
Key (a)
 0:01:46
0:01:46
 0:02:26
0:02:26
 0:01:53
0:01:53
 0:05:58
0:05:58
 0:10:06
0:10:06
 0:03:38
0:03:38
 0:04:09
0:04:09
 0:14:04
0:14:04
 0:01:57
0:01:57
 0:05:16
0:05:16
 0:04:21
0:04:21
 0:06:20
0:06:20
 0:08:32
0:08:32
 0:01:56
0:01:56
 0:10:20
0:10:20
 0:15:18
0:15:18
 0:10:10
0:10:10
 0:42:40
0:42:40
 0:09:44
0:09:44
 0:03:08
0:03:08
 0:03:54
0:03:54
 0:00:58
0:00:58
 0:04:31
0:04:31
 0:15:58
0:15:58