Random Processes - 04 - Mean and Autocorrelation Function Example

Join the YouTube channel for membership perks:

The previous videos provided definitions of the mean and autocorrelation function of a random process.

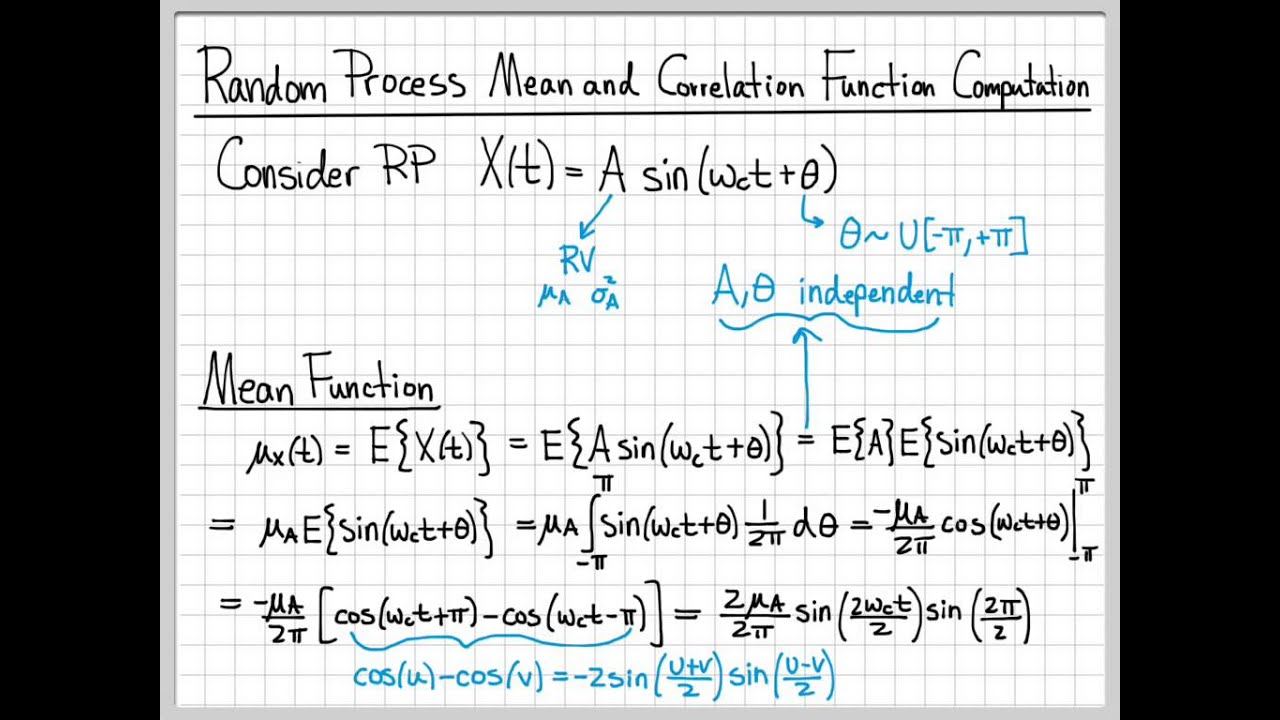
In this video we work with the random process X(t) = Asin(wc*t + theta) where both A and theta are random variables. We compute the mean function and autocorrelation function of this random process.

We show that the mean function is zero, and the autocorrelation function is just a function of the time difference t1-t2. Thus, this random process is a wide-sense stationary (WSS) random process (which we'll formally define late).

[jimmysantadeo]

Anyone that can explain a concept in a clear way definitely understands it, and YES YOU DO UNDERSTAND! LEGEND!

[BronzyEgames]

God bless you soon much🙏🙏🙏.A video you made 6 years ago is helping me celebrate today, thanks so much for this🥳🥳🥳.

[danieljamescarter]

You made something complicated very simple to understand, a true engineer, what a boss!

[drewphillips]

Adam this is such a fantastic explanation. You have no idea how much this helped on my grad school final exam!

[usmanskp]

Couldn't get more simpler and easy to digest! Bravo!

[karennakye]

You are the best you simplified this for me...I went through tonnes of videos before I found this.

[davidelias]

Thank you so much for these videos! Cannot begin to tell you how much these have helped me!

[atogh]

You are a genius and We need more of your examples

[MLDawn]

In 5:31, isn't that the formula for Covariance? I beliebe it needs to be divided by variance, if we want to get the correlation. Am I missing something?

[captainsquirtle]

Thank you so much!
Searched for a good example of such calculations for quite a long time, could have saved me some hours if i found it earlier :D

[trioxyify]

The trig-identity used above has a little mistake.
sin(X)sin(Y) = (1/2) [ cos (X - Y) - cos (X + Y) ]

[GiI]

Great job man, this is incredibly clear. Now I see how autocorrelation is related to expectation values ;)

[Jarrod_C]

Hey I am taking a Random Processes graduate course, and was wondering where I should begin on your channel and other channels. Thanks!

Further, I have no signals background, so do I need to start from the very beginning or can I jump right into the concept of random processes?

[Tschaegger]

THX! Good explanation, saw the same problem elsewhere and it was calculated directly with the integral for the expactation. My question is, would it be a single or a double integral if you would write the integral for the expecation? Becaue X(t1) and X(t1) or two random variables.

[manjitsingh]

Thank you so much for the lesson Adam 👍

[loicturounet]

Hi! Do you know a good book of exercises/problems about stochastic processes with solutions? I would like to practice before my exam
Thanks in advance

[thesoftwareguy]

how did you take the PDF of theta as 1/2pi ??

[gulfshores]

Hi Adam. Thanks for a very helpful video. I was wondering what tablet and software did you use? For a long time I've been wanting to buy a thinkpad tablet to write lecture notes on for easy navigation instead of writing on a paper and then later losing them. The device and program that you are using for hand writing seems very neat.

[syedalaiyba]

Are you making currently more vedioz on stochastic processes

[ramatbobby]

what if the value of x(t)=k where k is random variable uniformly distributed in range (-1, 1) ???
is it wide-sense stationary? or it is ergodic? if it is wide-sense stationary, what it is power??
appreciate for your help sir!!!