filmov
tv
Challenging physics problem | 2D motion

Показать описание
Three particles A, B and C are situated at the vertices of an equilateral triangle ABC of side d at time t = 0. Each of the particles moves with constant speed v. A always has its velocity along AB, B along BC and C along CA. At what time will the particles meet each other?
This is a famous problem from the 2D kinetmatics (Motion in a plane). The best way to attack this, and other such physics numerical, is to change your perspective!
================================================
Follow me at
================================================
Support me and learn more physics at the same time by enrolling in my Udemy course
================================================
Check my videos at Khan Academy
================================================
This is a famous problem from the 2D kinetmatics (Motion in a plane). The best way to attack this, and other such physics numerical, is to change your perspective!
================================================
Follow me at
================================================
Support me and learn more physics at the same time by enrolling in my Udemy course
================================================
Check my videos at Khan Academy
================================================
Challenging physics problem | 2D motion
IQ TEST
How To Solve Any Projectile Motion Problem (The Toolbox Method)
Tough AP 2D Kinematics Problem
🥵Toughest JEE ADV Physics Question in History 🤫| Full Solution #jeeadvanced #iit
2D Projectile Motion Made EASY in Physics PT 1! #physics #apphysics #apphysics1 #projectilemotion
Conservation of Momentum In Two Dimensions - 2D Elastic & Inelastic Collisions - Physics Problem...
Harder 2D Projectile Motion Made EASY in Physics PT 1 #physics #apphysics #apphysics1
Harder 2D Projectile Motion Made EASY in Physics PT 4 #physics #apphysics #apphysics1
2D Projectile Motion Made EASY in Physics PT 2! #physics #apphysics #apphysics1 #projectilemotion
the circle dot trick
Most Difficult Exams in INDIA 🤯 #upsc #iit #jeeadvanced #nda
Harder 2D Projectile Motion Made EASY in Physics PT 3 #physics #apphysics #apphysics1
2D Projectile Motion Made EASY in Physics PT 3! #physics #apphysics #apphysics1 #projectilemotion
Harder 2D Projectile Motion Made EASY in Physics PT 2 #physics #apphysics #apphysics1
2 OG of Physics 😈 in One Frame | PhysicsWallah
2D Projectile Motion Made EASY in Physics! #physics #apphysics #apphysics1 #projectilemotion
Harder 2D Projectile Motion Made EASY in Physics #physics #apphysics #apphysics1
Solving JEE Adv question in 30 sec 🤣#jee #jee2024 #jeeadvanced #iitjee #meme #funny
2D Projectile Motion Made EASY in Physics PT 4! #physics #apphysics #apphysics1 #projectilemotion
Sample AP Physics 1 Type Question (Dynamics + 2D Kinematics)
Pulley Physics Problem - Finding Acceleration and Tension Force
2D Elastic Collision Between Billiard Balls
motion in a plane numericals | class 11 physics numericals | motion in 2-d numericals | physics pyq
Комментарии
 0:08:44
0:08:44
 0:00:29
0:00:29
 0:13:02
0:13:02
 0:18:29
0:18:29
 0:00:19
0:00:19
 0:00:56
0:00:56
 0:10:25
0:10:25
 0:00:58
0:00:58
 0:00:50
0:00:50
 0:00:47
0:00:47
 0:00:47
0:00:47
 0:00:21
0:00:21
 0:00:59
0:00:59
 0:00:58
0:00:58
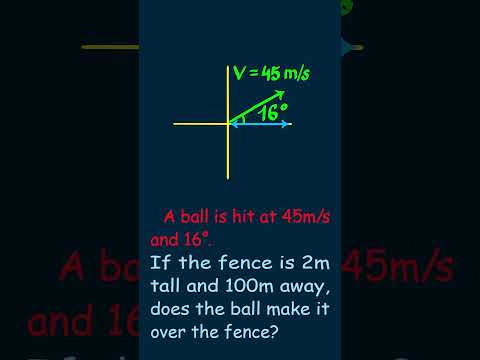 0:00:55
0:00:55
 0:00:15
0:00:15
 0:02:44
0:02:44
 0:02:55
0:02:55
 0:00:13
0:00:13
 0:00:53
0:00:53
 0:09:37
0:09:37
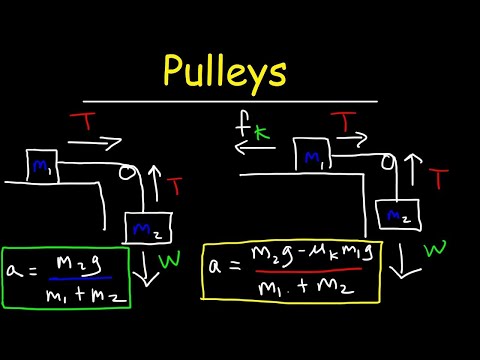 0:22:57
0:22:57
 0:15:20
0:15:20
 0:00:10
0:00:10