filmov
tv
Approximating the Definite Integral | Visualization | Animation | Calculus | R Programming

Показать описание
In this video, we explore the Riemann Sum concept applied to a cubic equation. To solve for the Riemann Sum, we compute the width of each subinterval and multiply it by the corresponding height (function evaluated within each subinterval), which gives us the area of each rectangle, and then we add the rectangles up. The Riemann Sum is an approximation to calculate the area under a curve using a definite integral. If we increase the number of rectangles over the interval, we decrease the error and obtain a better approximation. In essence, the Riemann Sum asymptotically approaches the Definite Integral as the number of subintervals (rectangles) approaches infinity.
#Animation
#Calculus
#Convergence
#DefiniteIntegral
#Integration
#Illustration
#Math
#Mathematics
#MathAnimation
#MathForHighSchool
#MathVisualization
#NumericalMethods
#RiemannSummation
#Visualization
#Animation
#Calculus
#Convergence
#DefiniteIntegral
#Integration
#Illustration
#Math
#Mathematics
#MathAnimation
#MathForHighSchool
#MathVisualization
#NumericalMethods
#RiemannSummation
#Visualization
 0:05:38
0:05:38
 0:09:50
0:09:50
 0:20:10
0:20:10
 0:11:05
0:11:05
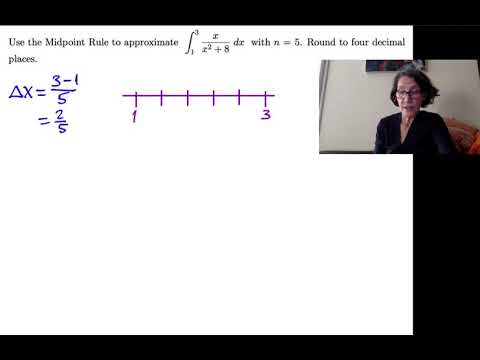 0:05:34
0:05:34
 0:09:24
0:09:24
 0:00:30
0:00:30
 0:10:36
0:10:36
 0:26:06
0:26:06
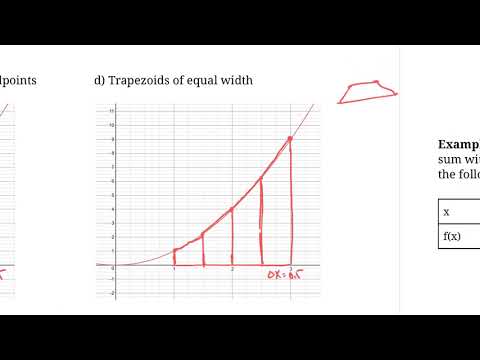 0:21:02
0:21:02
 0:12:10
0:12:10
 0:08:59
0:08:59
 0:05:17
0:05:17
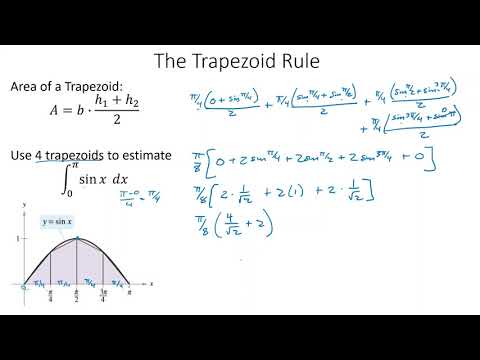 0:07:14
0:07:14
 0:28:56
0:28:56
 0:06:16
0:06:16
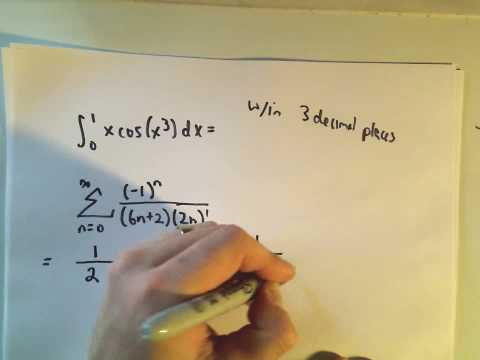 0:10:44
0:10:44
 0:00:53
0:00:53
 0:04:12
0:04:12
 0:12:49
0:12:49
 0:07:44
0:07:44
 0:14:36
0:14:36
 0:04:24
0:04:24
 0:12:54
0:12:54