filmov
tv
Riemann Sums - Left Endpoints and Right Endpoints
Показать описание
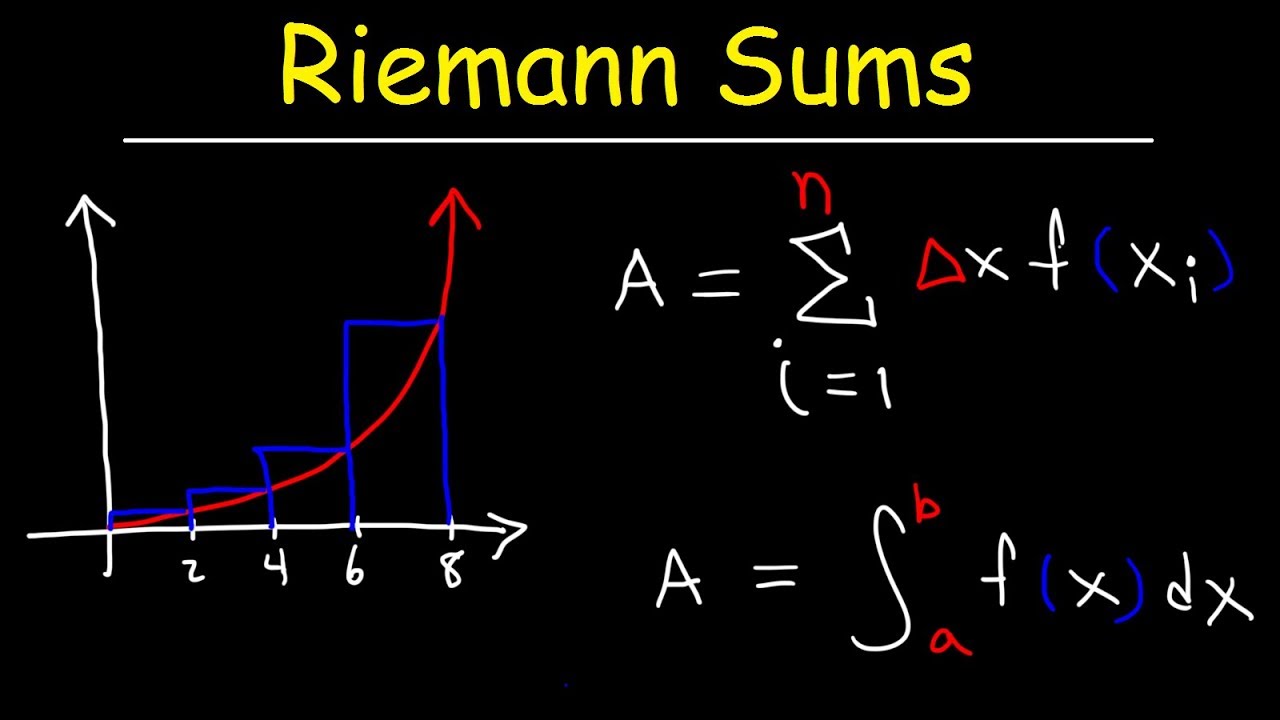
This calculus video tutorial provides a basic introduction into riemann sums. It explains how to approximate the area under the curve using rectangles over a closed interval. It explains how to determine the area of the region using left endpoints and right endpoints. The area under the curve is also equal to the definite integral of the function over the closed interval. The area can be approximated by taking the sum of the area of each rectangle. You can express this using sigma notation and calculate the sum using the appropriate summation formulas. The area of each rectangle is simply the product of the width and the height. The width is delta x and it represents the length of each subinterval. The height of the rectangle is represented by y which is a function of x or simply f(x). The average of the areas using the left and right endpoints is very close to the actual area under the curve. This tutorial contains a few examples and practice problems. The area using left endpoints is an under approximation or lower sum and the area using right endpoints is an over approximation or upper sum when the function is increasing. The upper sum is the higher of the two values and the lower sum is the lower of the two values.
Antiderivatives:
Basic Integration Problems:
Indefinite Integral:
Definite Integral:
Differential Equations:
_______________________________
Properties of Definite Integrals:
Rectilinear Motion Problems:
Sigma Notation - Calculus:
Riemann Sums - Area:
The Midpoint Rule:
________________________________
Finding Area - Limit Definition:
Definite Integrals - Geometry:
Fundamental Theorem - Part 1:
Fundamental Theorem - Part 2:
Net Change Theorem:
__________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
Antiderivatives:
Basic Integration Problems:
Indefinite Integral:
Definite Integral:
Differential Equations:
_______________________________
Properties of Definite Integrals:
Rectilinear Motion Problems:
Sigma Notation - Calculus:
Riemann Sums - Area:
The Midpoint Rule:
________________________________
Finding Area - Limit Definition:
Definite Integrals - Geometry:
Fundamental Theorem - Part 1:
Fundamental Theorem - Part 2:
Net Change Theorem:
__________________________________
Final Exams and Video Playlists:
Full-Length Videos and Worksheets:
0:20:10
Riemann Sums - Left Endpoints and Right Endpoints
0:09:01
How to find a Riemann sum using LEFT ENDPOINTS (KristaKingMath)
0:06:45
Riemann Sum - Left Endpoints | Set Up + TI84 Tip
0:01:54
How to use left hand riemann sums from a table
0:08:32
Left, Right, & Midpoint Riemann Sum Formulas
0:22:05
Area Problem - Riemann Sums - Left Endpoints - Right Endpoints | Calculus
0:13:34
Riemann Sum left endpoint - Algebraically
0:07:23
Riemann Sum: Left Endpoint & Right Endpoint Rectangles
0:06:42
Estimate the area under 1/x with left endpoint and right endpoint approximation
0:18:51
Riemann Integral/Sum: Right/Left Endpoints, Upper Sum, Lower Sum and Midpoint Estimate
0:06:31
How to use left hand riemann sum approximation
0:02:32
Riemann Sums: Left Endpoint
2:07:03
Calculus 1 Lecture 4.3: Area Under a Curve, Limit Approach, Riemann Sums
0:05:46
Riemann Sum Left end point
0:11:40
Midpoint Rule & Riemann Sums
0:13:18
How to Find a Definite Integral using Riemann Sums and the Limit Definition: Quadratic Example
0:08:12
Left hand Riemann sum e^-x^2 left endpoints rectangle approximation + left sum introduction.
0:28:02
Calculus AB/BC – 6.2 Approximating Areas with Riemann Sums
0:20:21
Riemann Sums - Left Endpoints - Right Endpoints | Estimate area Under y = sin x | Calculus
0:15:41
Riemann sums with right endpoints
0:09:51
Riemann Sums Left and Right Endpoint Approximations
0:04:47
Left hand Riemann sum e^sin(x) left endpoints rectangle approximation of integral e^sin(x).
0:05:16
Jordan McRae | Riemann Sums - Left Endpoints & Right Endpoints
0:05:29
Evaluate the Riemann sum for f(x)=5−12x, 2≤x≤14, with six subintervals, points to be left endpoints....
Комментарии