filmov
tv
A Nice Radical Equation Challenge | Algebra | Math Olympiad

Показать описание
A Nice Radical Equation Challenge | Algebra | Math Olympiad
Welcome to infyGyan!
In this algebraic video, we explore an intriguing radical equation that’s sure to challenge our algebraic skills. This problem is an excellent exercise for anyone preparing for Math Olympiad or simply looking to deepen their understanding of advanced algebra. Follow along as we break down the steps to solve this complex equation, and try to solve it yourself before we reveal the solution. Perfect for students and math enthusiasts alike!
If you enjoy tackling challenging math problems, be sure to like, share, and subscribe for more content like this!
📌 Topics Covered:
Radical equations
Algebraic manipulation
Problem-solving strategies
Quadratic equations
Quadratic formula
Substitution
Real solutions
Verification
Additional Resources:
#math #radicalequation #algebra #problemsolving #education #matholympiad #matholympiadpreparation #tutorial #quadraticequations
🔔 Don't forget to subscribe and hit the bell icon to stay updated with our latest math challenges and tutorials. If you enjoy the video, please give it a thumbs up and share it with your friends!
💬 Comment below if you have any questions or if you were able to solve the problem. We love hearing from our viewers!
Thank you for watching videos and happy problem-solving!
Welcome to infyGyan!
In this algebraic video, we explore an intriguing radical equation that’s sure to challenge our algebraic skills. This problem is an excellent exercise for anyone preparing for Math Olympiad or simply looking to deepen their understanding of advanced algebra. Follow along as we break down the steps to solve this complex equation, and try to solve it yourself before we reveal the solution. Perfect for students and math enthusiasts alike!
If you enjoy tackling challenging math problems, be sure to like, share, and subscribe for more content like this!
📌 Topics Covered:
Radical equations
Algebraic manipulation
Problem-solving strategies
Quadratic equations
Quadratic formula
Substitution
Real solutions
Verification
Additional Resources:
#math #radicalequation #algebra #problemsolving #education #matholympiad #matholympiadpreparation #tutorial #quadraticequations
🔔 Don't forget to subscribe and hit the bell icon to stay updated with our latest math challenges and tutorials. If you enjoy the video, please give it a thumbs up and share it with your friends!
💬 Comment below if you have any questions or if you were able to solve the problem. We love hearing from our viewers!
Thank you for watching videos and happy problem-solving!
Комментарии
 0:10:49
0:10:49
 0:01:58
0:01:58
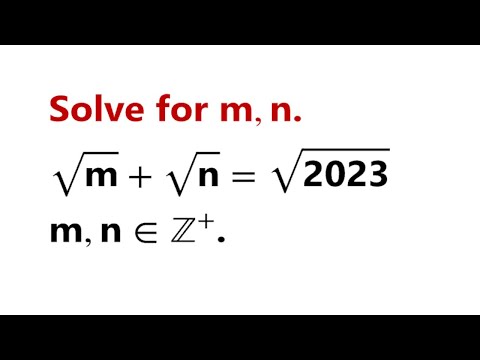 0:03:18
0:03:18
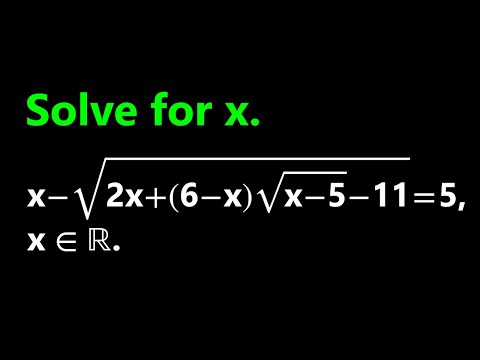 0:11:35
0:11:35
 0:11:36
0:11:36
 0:07:08
0:07:08
 0:14:49
0:14:49
 0:01:52
0:01:52
 0:00:41
0:00:41
 0:00:32
0:00:32
 0:12:41
0:12:41
 0:02:29
0:02:29
 0:00:52
0:00:52
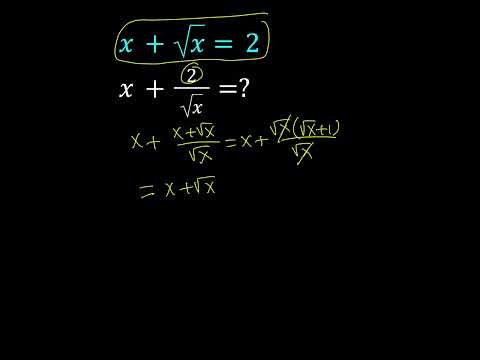 0:00:44
0:00:44
 0:11:19
0:11:19
 0:08:49
0:08:49
 0:06:25
0:06:25
 0:06:04
0:06:04
 0:05:33
0:05:33
 0:12:22
0:12:22
 0:08:59
0:08:59
 0:00:39
0:00:39
 0:19:51
0:19:51
 0:05:00
0:05:00