The Dirac Delta 'Function': How to model an impulse or infinite spike

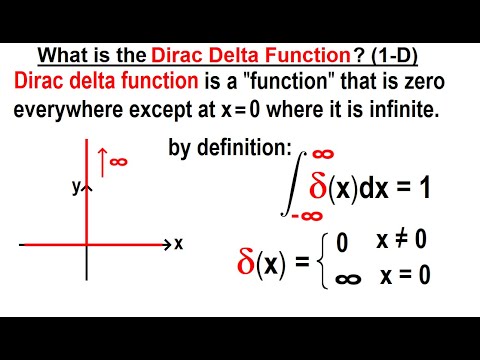
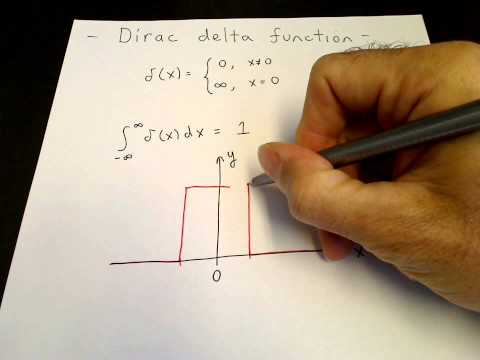
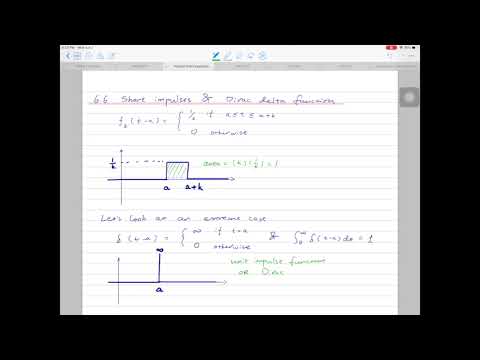
In this introduction to the Dirac Delta Function we'll see how we can deal with something happening instantaneously like a hammer hit. We will model this impulse with a 'function' that is infinite at one point and zero everywhere else. But such a thing isn't really a function! Sometimes we called it a functional or a generalized function. Regardless, it is defined by its pleasing properties such as what happens when you integrate the dirac delta function multiplied by another function. It can also be thought of as the derivative of the step function. In our next video we will study the Laplace Transform of the Dirac Delta Function and see how we can use it when studying differential equations.

****************************************************
Other Course Playlists:

***************************************************

► Want to learn math effectively? Check out my "Learning Math" Series:

►Want some cool math? Check out my "Cool Math" Series:

****************************************************

*****************************************************

This video was created by Dr. Trefor Bazett. I'm an Assistant Teaching Professor at the University of Victoria.

BECOME A MEMBER:

MATH BOOKS & MERCH I LOVE:

[kittyblossom]

Wow! You explained it really well. I've been trying to understand this Dirac Delta Function for about an hour now, and couldn't find any resources that explains it visually. Your explanation is just what I needed. Thank you.

[homervalizadeh]

It's not even about distribution theory, but I found here a right place to learn about distribution theory. You're awesome in your job.

[disasterslight]

I can' thank you enough for this video. We were doing generalized functions rigorously, and I was so lost. This explanation just made so much sense. Please consider making some rigorous advanced math videos! We need you!

[ayanbhowmick]

I'm Doing My MS in Chemical Science at Indian Association for Cultivation of Science, We have a course on Mathematical and Computational Chemistry, this video really helped me a lot.

[intereststcentury]

Thank You, Dr!!!! A wonderful and clear explanation

[NumbToons]

Came here to hear the pronunciation of 'Dirac', forgot about it middway, ended up watching the whole video. Then realised No utterance of 'Dirac' but still, very bounding explanation.

[sumitkumarsahoo]

your vector calculus helped me too much, very very grateful to you

[subhadipkarmakar]

Thank you Sir for explaining these concepts 🌻

[ahmed]

The neatest explanation one can find on youtube ! Thanks

[porit]

I didn't even know there is a mean value theorem for integrals. Thank you so much!

[asnakeseyoum]

I watched your linear algebra.It help me a lot. Thanks!

[tylerellison]

Thanks! Your content has helped me with conceptualizing many ideas presented in my EE courses.

[bernardlemay]

Thank you so much for that clear explanation.

[RadoslavFicko]

The Dirac distribution is the Fourier transform of unity and a special case of convolution, where A*f=g, g(x)=d(x-y). f(y)dy, if we imagine the gravitational interaction as a function of g(x) and the electromagnetic interaction as a function of f(y), then these forces (i.e. the lines of force) only interact when x is equal to y ( the Dirac impulse).

[taylorb]

cool video. i remember first encountering this function in the context of point charges in EM theory.

[Probably-Bobby]

Great video as always! Could you elaborate on why the delta function isn't considered a function? At about 2:24 you mention that it's because the value at x=a isn't a number, but neither is x=0 for the function 1/x, so what's the difference between the two? Thanks!

[RichardJohnson_dydx]

Thank you. Once again, your videos are getting me through engineering school.

[TheRevAlokSingh]

The delta is in fact a function if you use the hyperreals. One model of it is a gaussian. It cannot be given as a real function because both its domain and range are not standard (infinitesimal and unlimited)

[wilurbean]

"Step-function... what are you doing back there..!" 😲😲😈😈

[jhmaths]

Hi Trefor, I think you could be a great teacher but unfortunately I can't tolerate this thing that seems fashionable at the moment (especially among UK news reporters) of speeding up and slowing down speech for no apparent reason.