filmov
tv
a crazy iterated limit.
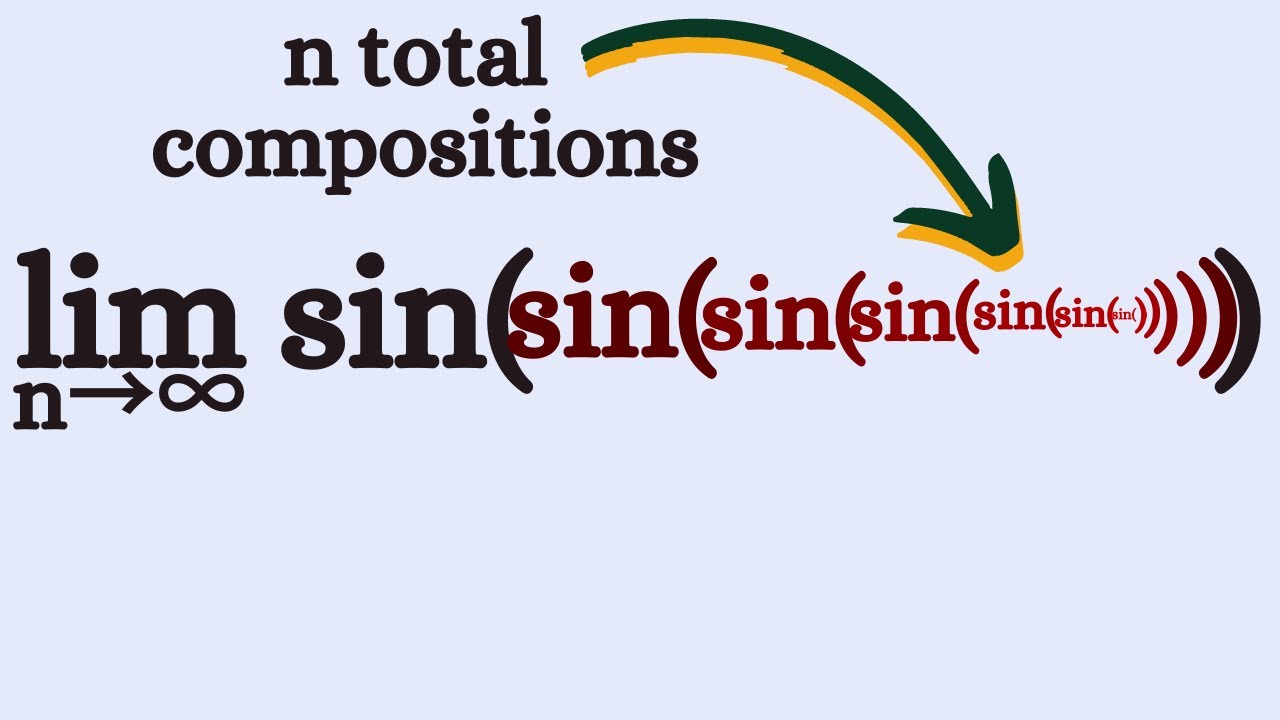
Показать описание
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟Suggest a problem🌟
🌟my other channels🌟
🌟My Links🌟
🌟Suggest a problem🌟
a crazy iterated limit.
Iterated Limits Existing Does Not Imply the Limit Exists
Advanced Calculus | Limit of functions of Several Variables Part-III Repeated OR Iterated limits
Iterated Limits 2
Iterated(Repeated) Limits & Simultaneous Limit of function of two variables x and y
a surprising collapse of this iterated sine function.
Limits of Multivariable Functions - Calculus 3
What is the value of this crazy iterated square??
Iterated Limits 1
What is Repeated Limit
Simultaneous and Iterated Limits | Limit and Continuity of Functions of Two Variables |
Calculus 3 Lecture 14.2: How to Solve Double/Repeated/Iterated Integrals
Iterated limit & Continuity & Examples|Lecture no.:-52
Lec 1 Function of several variable, simultaneous limit, double limit, repeated limit, iterated limit
functions of several variables (part 8) repeated limits / iterated limit functions two variables Hd
Iterated Limits Mathvyas #B.Sc. #Mathematics #tutorials #EngineeringMaths #btechmathematics
Iteration Limit Reached in AMOS
functions of several variables (L9) repeated limits / iterated limit functions two variables Hd sir
How An Infinite Hotel Ran Out Of Room
functions of several variables/ multivariable calculus/ simultaneous limit/ iterated limit by Hd sir
Mysterious Holes || Mathematical Analysis || Repeated Series
calculus III-Sketching the region represented by an iterated integral
Iterated Integrals
The Simple Solution to Traffic
Комментарии
 0:10:57
0:10:57
 0:03:48
0:03:48
 0:19:11
0:19:11
 0:07:15
0:07:15
 0:09:35
0:09:35
 0:18:28
0:18:28
 0:19:04
0:19:04
 0:16:10
0:16:10
 0:10:00
0:10:00
 0:00:41
0:00:41
 0:31:13
0:31:13
 3:23:48
3:23:48
 0:17:06
0:17:06
 0:25:53
0:25:53
 0:40:52
0:40:52
 0:02:17
0:02:17
 0:04:01
0:04:01
 0:28:18
0:28:18
 0:06:07
0:06:07
 0:43:21
0:43:21
 0:15:49
0:15:49
 0:08:44
0:08:44
 0:07:25
0:07:25
 0:05:14
0:05:14