filmov
tv
The Distance Between Numbers - Numberphile

Показать описание
More links & stuff in full description below ↓↓↓
Tom discusses a series that, by one definition, has an unexpected property.
Thanks to Gavin Jared Bala for assistance with the calculation of other convergent p-adic sequences.
NUMBERPHILE
Videos by Brady Haran
Tom discusses a series that, by one definition, has an unexpected property.
Thanks to Gavin Jared Bala for assistance with the calculation of other convergent p-adic sequences.
NUMBERPHILE
Videos by Brady Haran
The Distance Between Numbers - Numberphile
Absolute value as distance between numbers | 7th grade | Khan Academy
GED Math Distance on Number Line!
Calculate the Distance Between Two Complex Numbers (Example)
The Distance Between Two Complex Numbers (Example)
How to Find the Distance Between Two Numbers | Measuring Distance, Absolute Value
How to Find the Distance Between Two Points - How to Use the Distance Formula
How To Find The Distance Between Two Points
CAT 2024 | Quantitative Aptitude | 250+ Must-Do Quant Questions | Quant for CAT 06
Finding the distance between 2 numbers on a number line
Find the Distance Between Two Points on a Number Line
How do we Find the Distance between Two Points on a Number Line? | Don't Memorise
Distance between complex numbers
Determine the distance of two points on a number line
Find distance between two points on a real number line
Finding the Distance Between Points on a Number Line
How to Find the Distance Between 2 Points on a Number Line for GED Math Fast Lesson
Finding the Distance Between Two Complex Numbers Using Vectors
Applying the distance formula to find the distance between two points
Midpoint and distance on complex plane | Imaginary and complex numbers | Precalculus | Khan Academy
Find the Distance on the Real Number Line Between Two Points using the Absolute Value Function
How to understand ABSOLUTE VALUES and DISTANCE - Precalculus
Find the distance between the points (-2,0) and (4,5)
Find the minimum distance between two numbers | GeeksforGeeks
Комментарии
 0:21:34
0:21:34
 0:05:29
0:05:29
 0:04:19
0:04:19
 0:02:34
0:02:34
 0:01:30
0:01:30
 0:05:39
0:05:39
 0:04:36
0:04:36
 0:05:19
0:05:19
 1:03:14
1:03:14
 0:04:01
0:04:01
 0:02:33
0:02:33
 0:02:51
0:02:51
 0:04:48
0:04:48
 0:02:59
0:02:59
 0:01:35
0:01:35
 0:02:16
0:02:16
 0:01:51
0:01:51
 0:02:01
0:02:01
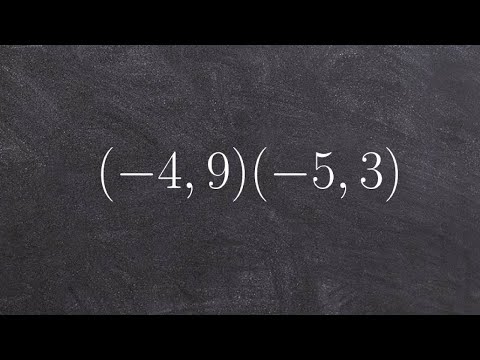 0:04:21
0:04:21
 0:06:10
0:06:10
 0:01:23
0:01:23
 0:13:45
0:13:45
 0:03:03
0:03:03
 0:15:56
0:15:56