filmov
tv
A-Level Maths: A1-16 Proof by Contradiction Examples

Показать описание
A-Level Maths: A1-16 Proof by Contradiction Examples
A-Level Further Maths: A1-16 Proof by Induction: 2^n+6^n is divisible by 8
Proof 1 • Proof by Contradiction • P2 Ex1A • 💡
A-Level Further Maths: A1-17 Proof by Induction: Sequence u_(n+1) = 1 - 2u_n
A-Level Maths: A1-15 Proving there are Infinitely Many Primes
A-Level Maths G1-16 Differentiation: Differentiate sin(x) from First Principles
YEAR 2 ➡️ Day 38: Proof by Contradiction pt. 1 • 100 Days of A-Level Maths 🧮
Edexcel A level Maths: 1.1 Proof By Contradiction
A-Level Maths: A1-04 [Introducing Proof by Exhaustion]
A-Level Maths: A1-05 [Proof by Exhaustion Examples]
[P4], Edexcel, (GCE), 9MA0/01, A2, (UK), Pure Maths (Paper 1) OCT 2020, Q16, Proof by Contradiction
A-Level Maths: A1-10 Introducing Proof by Contradiction
Proof 1 • Proof by Deduction • P1 Ex7D • 💡
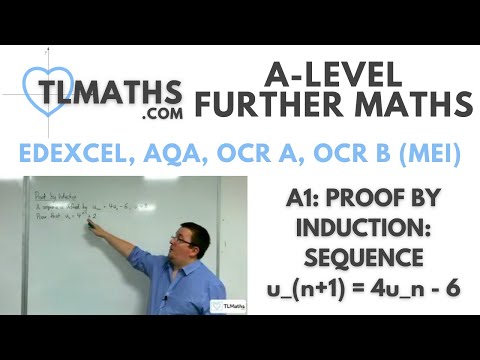
A-Level Further Maths: A1-18 Proof by Induction: Sequence u_(n+1) = 4u_n - 6
What’s YOUR English level? 🇬🇧
What's your level in English? A1/A2? B1/B2? C1/C2? Check this video! 👀 🇺🇸 🇬🇧
A-Level Maths: A1-06 [Introducing Proof by Deduction]
A-Level Maths: A1-01 [Introduction to Proof]
A-Level Further Maths: A1-19 Proof by Induction: Sequence u_(n+1) = 3u_n - 2^n
#Trigonometry all formulas
NEWYES Calculator VS Casio calculator
A-Level Maths: A1-12 Proving √3 is Irrational
A-Level Further Maths: A1-26 Proof by Induction: Inequality Example 1
One Day Before 😂 Maths Exam || Hard Working 😭 || #shorts #youtubeshorts #motivation
Комментарии
 0:06:36
0:06:36
 0:08:45
0:08:45
 0:17:20
0:17:20
 0:05:38
0:05:38
 0:06:47
0:06:47
 0:04:53
0:04:53
 0:00:49
0:00:49
 0:13:40
0:13:40
 0:09:07
0:09:07
 0:15:40
0:15:40
![[P4], Edexcel, (GCE),](https://i.ytimg.com/vi/Iq853ccRuKY/hqdefault.jpg) 0:11:30
0:11:30
 0:01:13
0:01:13
 0:15:27
0:15:27
 0:03:17
0:03:17
 0:00:20
0:00:20
 0:00:30
0:00:30
 0:04:45
0:04:45
 0:02:20
0:02:20
 0:03:31
0:03:31
 0:00:16
0:00:16
 0:00:14
0:00:14
 0:05:21
0:05:21
 0:08:34
0:08:34
 0:00:16
0:00:16